Answered step by step
Verified Expert Solution
Question
1 Approved Answer
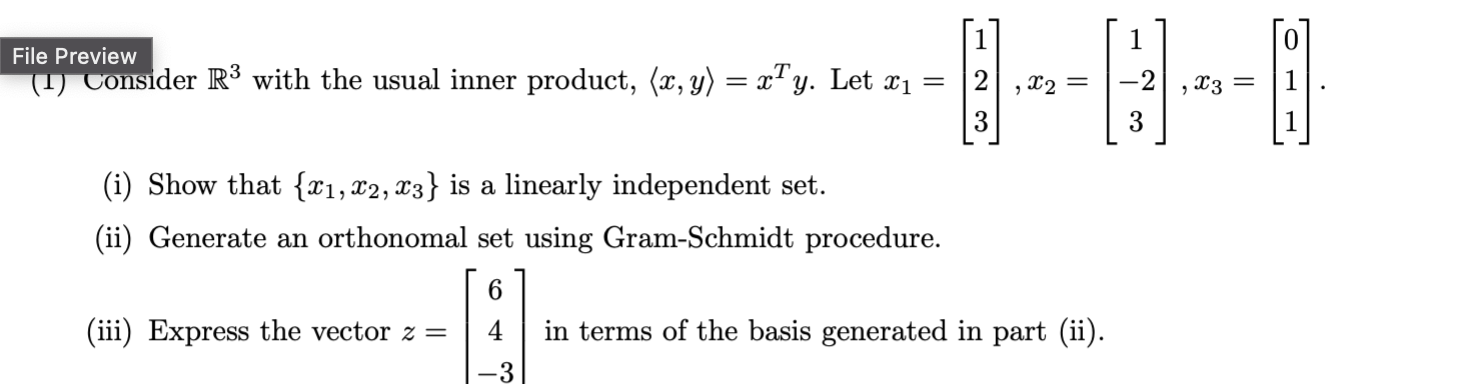
File Preview (I) Consider R^(3) with the usual inner product, (:x,y:)=x^(T)y . Let x_(1)=[[1],[2],[3]],x_(2)=[[1],[-2],[3]],x_(3)=[[0],[1],[1]] . (i) Show that {x_(1),x_(2),x_(3)} is a linearly independent set. (ii)
File Preview\ (I) Consider
R^(3)with the usual inner product,
(:x,y:)=x^(T)y. Let
x_(1)=[[1],[2],[3]],x_(2)=[[1],[-2],[3]],x_(3)=[[0],[1],[1]].\ (i) Show that
{x_(1),x_(2),x_(3)}is a linearly independent set.\ (ii) Generate an orthonomal set using Gram-Schmidt procedure.\ (iii) Express the vector
z=[[6],[4],[-3]]in terms of the basis generated in part (ii).
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


