FINANCIAL RISK MANAGEMENT

----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
MAKE USE OF SOME OF THE FORMULAE HERE:
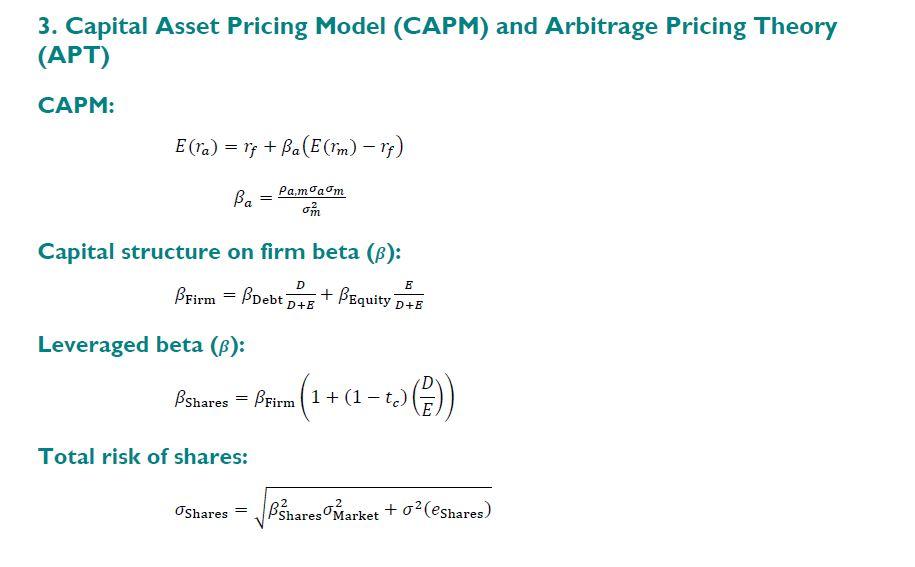
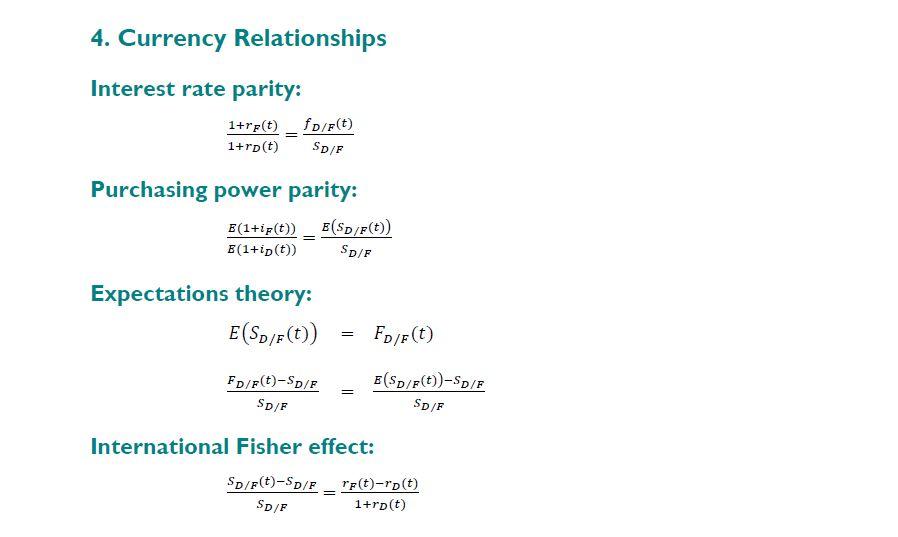
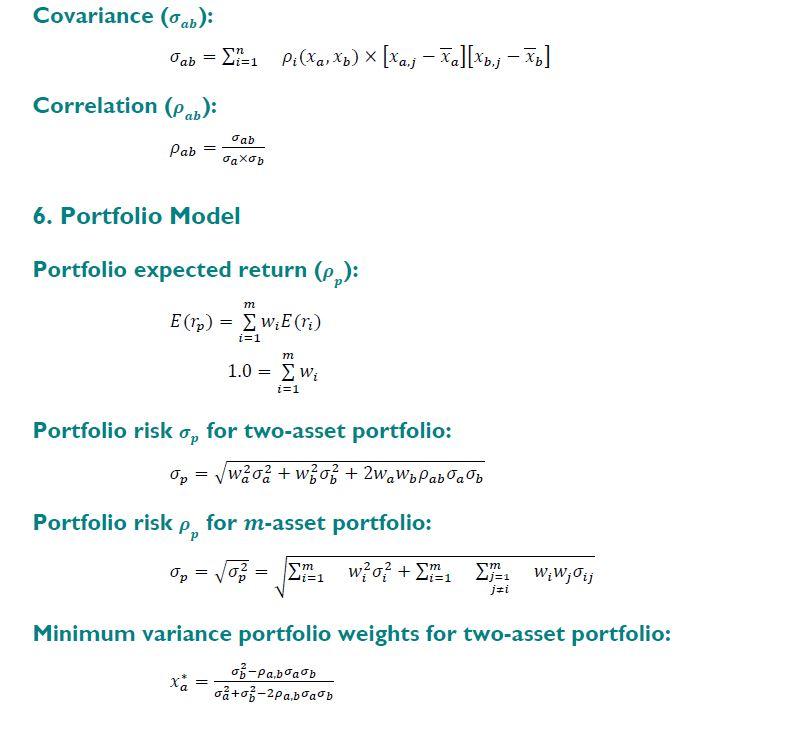
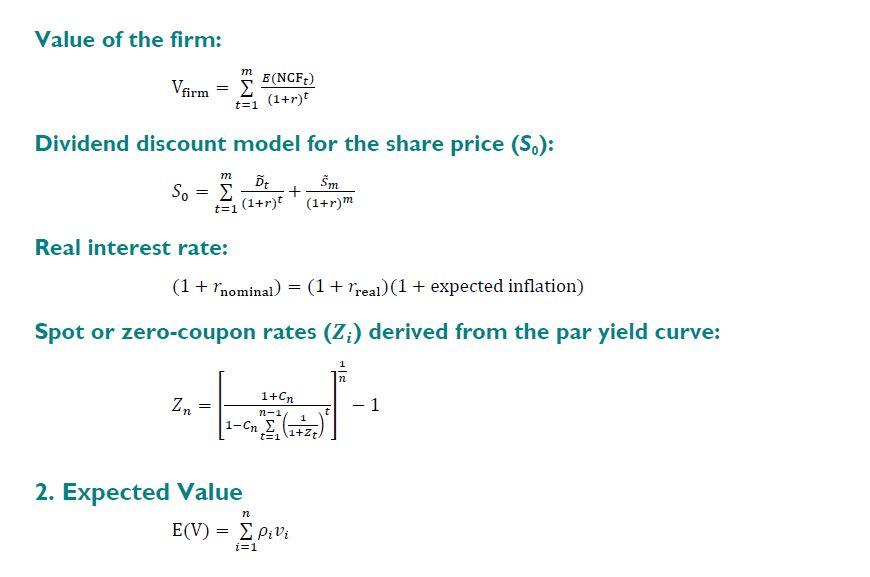
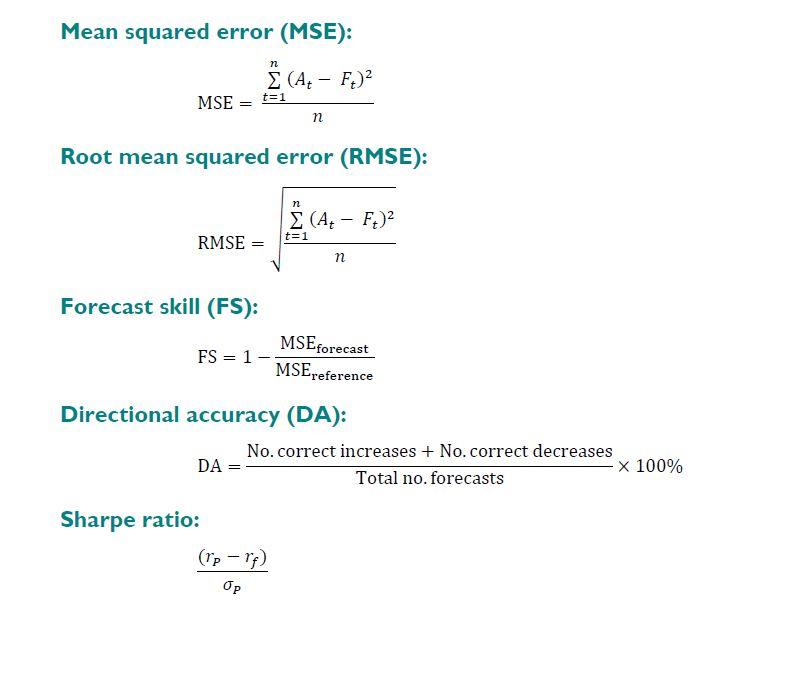
You wish to add a third commodity to this portfolio, and you plan to introduce one that you hope will reduce the risk of the portfolio. You invest 90m in coffee. Coffee has an annual volatility of 10%, and again the time horizon is six months and the confidence limit is 1.96. What is the coffee value at risk, and what is the value at risk for the whole portfolio, given that the correlation between coffee and gold is -0.40, and between coffee and silver is -0.30? (13 marks) 3. Capital Asset Pricing Model (CAPM) and Arbitrage Pricing Theory (APT) CAPM: E (ra) = rf + Ba(E('m) rp) Ba Pamam Capital structure on firm beta (): D E Bfirm = BDebt D+E + BEquity D+E Leveraged beta (B): Pshares Priro (1+(1-10) Total risk of shares: Shares Bhares Market + 02 (@shares) 4. Currency Relationships Interest rate parity: 1+rF(t) 1+r(t) fo/f(t) Sd/F Purchasing power parity: E(1+ip(t)) - E(SD/F(t)) E(1+ip(t)) SD/F Expectations theory: E(Sp/f(t)) FD/F(t) FD/F(t)-SD/F SD/F E(Sp/f(t))-SD/F SD/F = International Fisher effect: Sp/f(t)-SD/F re(t)-ro(t) 1+rp(t) SD/F Covariance (ab): Jab = =1 Pilxa, xb) * [X a.) [a][xb.j #b] Correlation (Pad): Pab Jaxob 6. Portfolio Model Portfolio expected return (Pm): E(rp) = w;E (ri) - Emera m 1.0 = w; i=1 Portfolio risk on for two-asset portfolio: Op = woz + wo + 2wqWbPablo Portfolio risk pp for m-asset portfolio: Op = Vo} = E. w?o? + STEI w? ? + , ww,; jui Minimum variance portfolio weights for two-asset portfolio: -Pa.beach - xa o+0%-2Paboado = Value of the firm: m E(NCF) Vfirm = 2 = (1+r) t=1 Dividend discount model for the share price (S.): m m So = BE (1+r) + (1+r)m t=1 Real interest rate: (1 + rnominal) = (1 + rreal) (1 + expected inflation) Spot or zero-coupon rates (2) derived from the par yield curve: 1+Cn Zn - 1 n-1 1-C tsi (1+2, 2. Expected Value n E(V) = Pivi i=1 Mean squared error (MSE): n EM- (, - F.)2 MSE = t=1 n Root mean squared error (RMSE): n (- (A4 - Ft)2 t=1 RMSE n Forecast skill (FS): MSE forecast FS = 1- MSE reference Directional accuracy (DA): DA No. correct increases + No. correct decreases X 100% Total no. forecasts Sharpe ratio: (rp-re) op You wish to add a third commodity to this portfolio, and you plan to introduce one that you hope will reduce the risk of the portfolio. You invest 90m in coffee. Coffee has an annual volatility of 10%, and again the time horizon is six months and the confidence limit is 1.96. What is the coffee value at risk, and what is the value at risk for the whole portfolio, given that the correlation between coffee and gold is -0.40, and between coffee and silver is -0.30? (13 marks) 3. Capital Asset Pricing Model (CAPM) and Arbitrage Pricing Theory (APT) CAPM: E (ra) = rf + Ba(E('m) rp) Ba Pamam Capital structure on firm beta (): D E Bfirm = BDebt D+E + BEquity D+E Leveraged beta (B): Pshares Priro (1+(1-10) Total risk of shares: Shares Bhares Market + 02 (@shares) 4. Currency Relationships Interest rate parity: 1+rF(t) 1+r(t) fo/f(t) Sd/F Purchasing power parity: E(1+ip(t)) - E(SD/F(t)) E(1+ip(t)) SD/F Expectations theory: E(Sp/f(t)) FD/F(t) FD/F(t)-SD/F SD/F E(Sp/f(t))-SD/F SD/F = International Fisher effect: Sp/f(t)-SD/F re(t)-ro(t) 1+rp(t) SD/F Covariance (ab): Jab = =1 Pilxa, xb) * [X a.) [a][xb.j #b] Correlation (Pad): Pab Jaxob 6. Portfolio Model Portfolio expected return (Pm): E(rp) = w;E (ri) - Emera m 1.0 = w; i=1 Portfolio risk on for two-asset portfolio: Op = woz + wo + 2wqWbPablo Portfolio risk pp for m-asset portfolio: Op = Vo} = E. w?o? + STEI w? ? + , ww,; jui Minimum variance portfolio weights for two-asset portfolio: -Pa.beach - xa o+0%-2Paboado = Value of the firm: m E(NCF) Vfirm = 2 = (1+r) t=1 Dividend discount model for the share price (S.): m m So = BE (1+r) + (1+r)m t=1 Real interest rate: (1 + rnominal) = (1 + rreal) (1 + expected inflation) Spot or zero-coupon rates (2) derived from the par yield curve: 1+Cn Zn - 1 n-1 1-C tsi (1+2, 2. Expected Value n E(V) = Pivi i=1 Mean squared error (MSE): n EM- (, - F.)2 MSE = t=1 n Root mean squared error (RMSE): n (- (A4 - Ft)2 t=1 RMSE n Forecast skill (FS): MSE forecast FS = 1- MSE reference Directional accuracy (DA): DA No. correct increases + No. correct decreases X 100% Total no. forecasts Sharpe ratio: (rp-re) op












