Question
Find the eigenvalues of A. Then determine the possible Jordan canonical forms of A (up to permutation of the blocks). ii) Compute the Jordan canonical
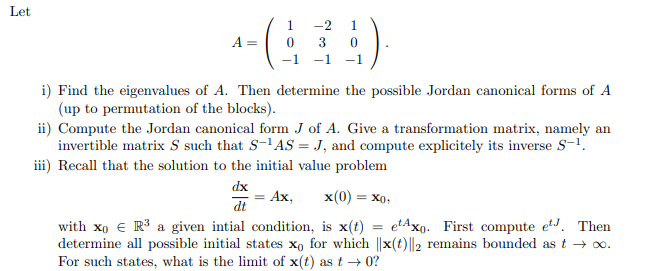
Find the eigenvalues of A. Then determine the possible Jordan canonical forms of A (up to permutation of the blocks). ii) Compute the Jordan canonical form J of A. Give a transformation matrix, namely an invertible matrix S such that S?1AS = J, and compute explicitely its inverse S?1. iii) Recall that the solution to the initial value problem dx = Ax, x(0) = x0, dt with x0 ? R3 a given intial condition, is x(t) = etA x0 . First compute etJ . Then determine all possible initial states x0 for which ?x(t)?2 remains bounded as t ? ?. For such states, what is the limit of x(t) as t ? 0?
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


