Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Find the general solution of (x y2 + 3x) y' = xy - 3y. Give the general solution of y + 6y + 18y
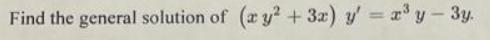
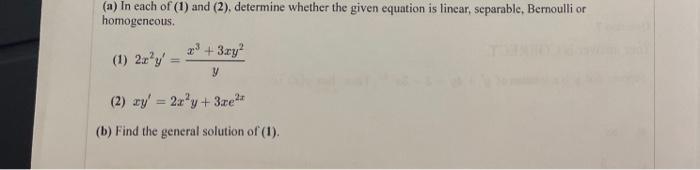
Find the general solution of (x y2 + 3x) y' = xy - 3y. Give the general solution of y" + 6y + 18y = 0. (a) In each of (1) and (2), determine whether the given equation is linear, separable, Bernoulli or homogeneous. (1) 2xy = x + 3xy y (2) ay = 2xy + 3re (b) Find the general solution of (1).
Step by Step Solution
★★★★★
3.48 Rating (151 Votes )
There are 3 Steps involved in it
Step: 1
To find the general solution of the given differential equations lets analyze each equation separately 1 The equation is 2x2y x3 3xy2y a To determine ...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Differential Equations and Linear Algebra
Authors: Jerry Farlow, James E. Hall, Jean Marie McDill, Beverly H. West
2nd edition
131860615, 978-0131860612
