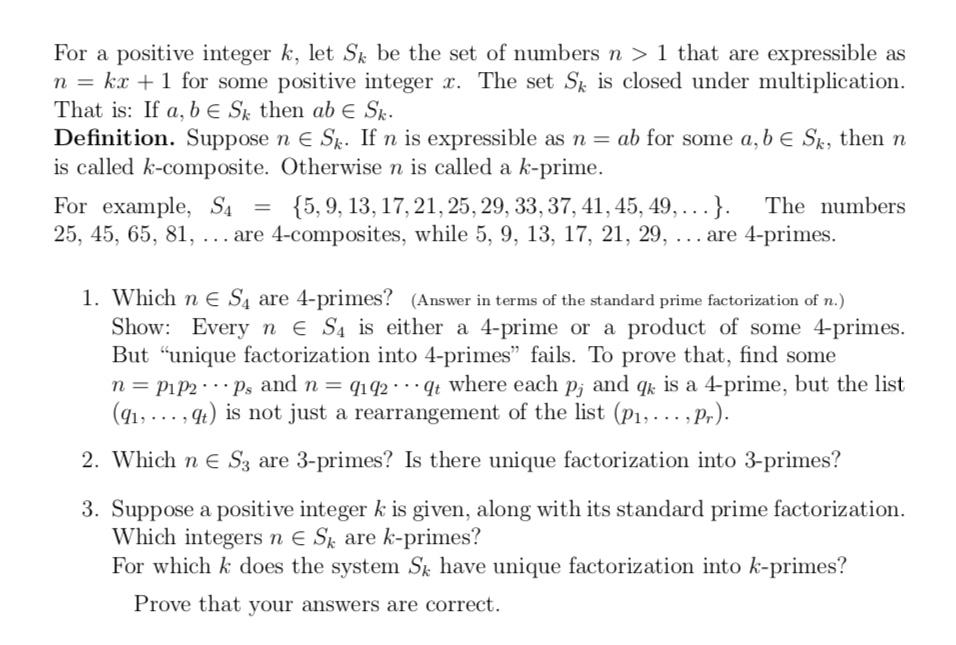
For a positive integer k, let Sk be the set of numbers n > 1 that are expressible as n = kx +1 for some positive integer r. The set S is closed under multiplication. That is: If a, bE Sk then ab E Sk. Definition. Suppose n E Sk. If n is expressible as n = ab for some a, b E Sk, then n is called k-composite. Otherwise n is called a k-prime. {5, 9, 13, 17, 21, 25, 29, 33, 37, 41,45, 49,...}. For example, SA 25, 45, 65, 81, ... are 4-composites, while 5, 9, 13, 17, 21, 29, ... are 4-primes. The numbers 1. Which n E S4 are 4-primes? (Answer in terms of the standard prime factorization of n.) Show: Every n e S4 is either a 4-prime or a product of some 4-primes. But "unique factorization into 4-primes" fails. To prove that, find some n = PiP2 Ps and n = q192 qt where each p; and q is a 4-prime, but the list (91, ... , 4t) is not just a rearrangement of the list (p1,... , Pr). 2. Which n E S3 are 3-primes? Is there unique factorization into 3-primes? 3. Suppose a positive integer k is given, along with its standard prime factorization. Which integersn e S are k-primes? For which k does the system Sk have unique factorization into k-primes? Prove that your answers are correct.