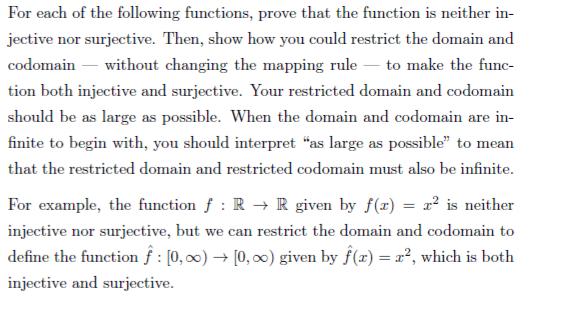
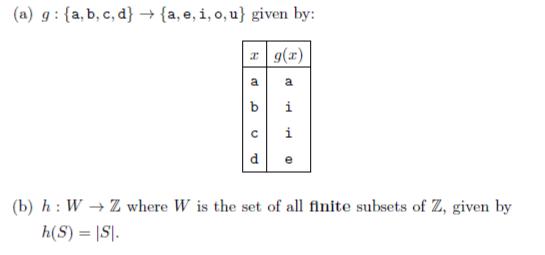
For each of the following functions, prove that the function is neither in- jective nor surjective. Then, show how you could restrict the domain and codomain without changing the mapping rule to make the func- tion both injective and surjective. Your restricted domain and codomain should be as large as possible. When the domain and codomain are in- finite to begin with, you should interpret "as large as possible" to mean that the restricted domain and restricted codomain must also be infinite. For example, the function f: R R given by f(r) = x? is neither injective nor surjective, but we can restrict the domain and codomain to define the function f : (0, 0) - [0, 0) given by f(z) = =', which is both injective and surjective. (a) g: {a, b, c, d} {a, e, i, o, u} given by: g(x) a a b i i d e (b) h: W Z where W is the set of all finite subsets of Z, given by h(S) = |S].