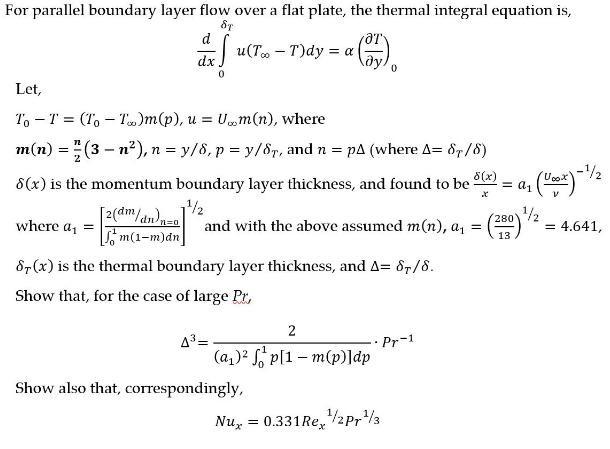
For parallel boundary layer flow over a flat plate, the thermal integral equation is, d |u(T - T)dy = a dx dy. Let, To - T = (To - T)m(p), u = U.m(n), where m(n) = (3 n), n = y/8, p = y/8r, and n = pA (where A= &r/8) 8(x) 8(x) is the momentum boundary layer thickness, and found to be = a1 [2(dm/dn)n So m(1-m)dn 1/2 = 4.641, 280 where a, = and with the above assumed m(n), a 13 87 (x) is the thermal boundary layer thickness, and A= 87/8. Show that, for the case of large Pr, 2 A3= Pr-1 (a,)? S, p[1 m(p)]dp Show also that, correspondingly, Nu, 0.331 Re, 2Pr/3