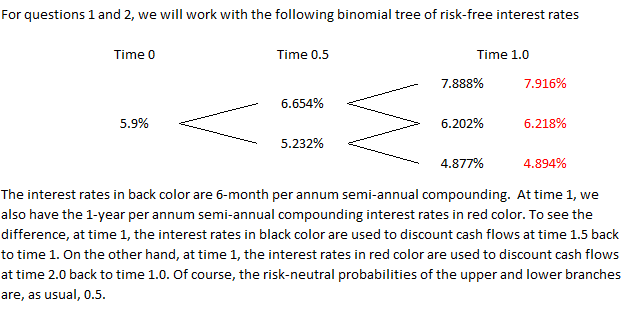

For questions 1 and 2, we will work with the following binomial tree of risk-free interest rates Time 0 Time 0.5 Time 1.0 7.888% 7.916% 6.654% 5.9% 6.202% 6.218% 5.232% 4.877% 4.894% The interest rates in back color are 6-month per annum semi-annual compounding. At time 1, we also have the 1-year per annum semi-annual compounding interest rates in red color. To see the difference, at time 1, the interest rates in black color are used to discount cash flows at time 1.5 back to time 1. On the other hand, at time 1, the interest rates in red color are used to discount cash flows at time 2.0 back to time 1.0. Of course, the risk-neutral probabilities of the upper and lower branches are, as usual, 0.5. Question 1 Please don't round up your answers at all. You can make Excel display a number to a certain number of decimal places for presentation purposes, but please don't round up. The best approach is to enter your formulas straight into the answer cells. a) Enter the 1-year interest rate at time 0 here: b) Consider the 1-year callable bond, face value $100, paying semi-annual coupon rate of 8%. The call price is par. The bond can only be called at time 0.5. The bond is currently selling for $99.61. Note that the bond is subject to credit risk and liquidity risk. Enter the static spread of the bond here: c) Consider the same callable bond in b) and the binomial interest rate tree: Enter the option-adjusted spread here: d) Consider the same callable bond in b) and the binomial interest rate tree: Enter the spread due to optionality here: e) Given the option-adjusted spread in c), what is the price of an identical bond except that it is non-callable? Enter your answer here: For questions 1 and 2, we will work with the following binomial tree of risk-free interest rates Time 0 Time 0.5 Time 1.0 7.888% 7.916% 6.654% 5.9% 6.202% 6.218% 5.232% 4.877% 4.894% The interest rates in back color are 6-month per annum semi-annual compounding. At time 1, we also have the 1-year per annum semi-annual compounding interest rates in red color. To see the difference, at time 1, the interest rates in black color are used to discount cash flows at time 1.5 back to time 1. On the other hand, at time 1, the interest rates in red color are used to discount cash flows at time 2.0 back to time 1.0. Of course, the risk-neutral probabilities of the upper and lower branches are, as usual, 0.5. Question 1 Please don't round up your answers at all. You can make Excel display a number to a certain number of decimal places for presentation purposes, but please don't round up. The best approach is to enter your formulas straight into the answer cells. a) Enter the 1-year interest rate at time 0 here: b) Consider the 1-year callable bond, face value $100, paying semi-annual coupon rate of 8%. The call price is par. The bond can only be called at time 0.5. The bond is currently selling for $99.61. Note that the bond is subject to credit risk and liquidity risk. Enter the static spread of the bond here: c) Consider the same callable bond in b) and the binomial interest rate tree: Enter the option-adjusted spread here: d) Consider the same callable bond in b) and the binomial interest rate tree: Enter the spread due to optionality here: e) Given the option-adjusted spread in c), what is the price of an identical bond except that it is non-callable? Enter your answer here








