For reference, here is Problem 5.

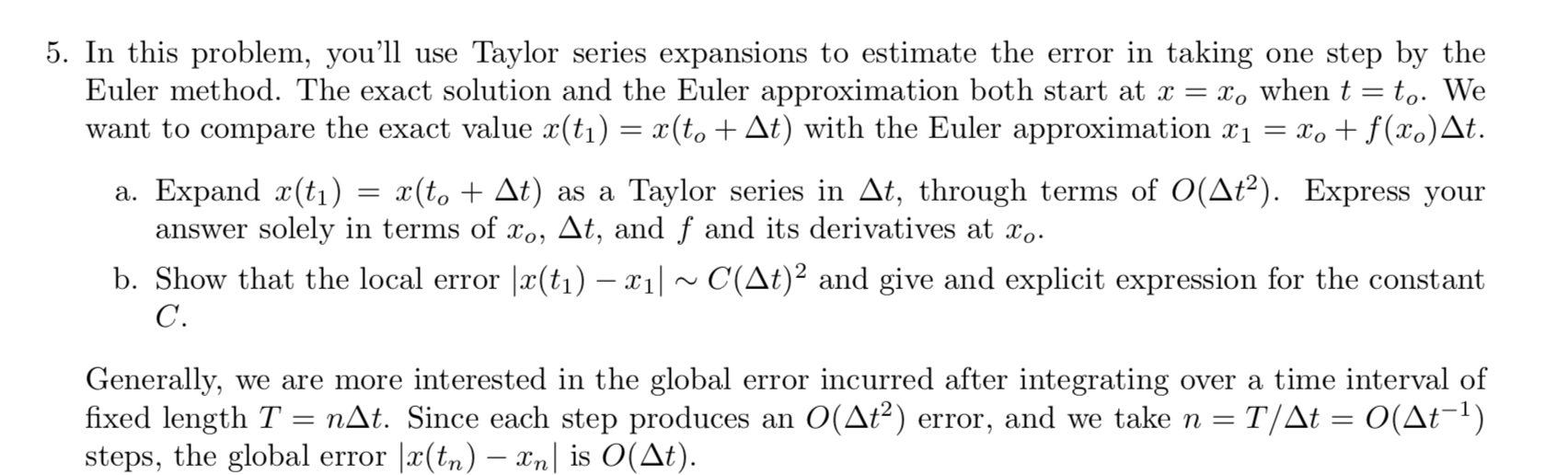
7. Bonus problem (it means you don't have to solve it): if you want to do a massive amount of algebra, try to redo problem 5 for the 4th-order Runge-Kutta method! It'll be a nightmare if you don't use Mathematica or some other symbolic manipulation language 5. In this problem, you'll use Taylor series expansions to estimate the error in taking one step by the Euler method. The exact solution and the Euler approximation both start at x = x, when t = to. We want to compare the exact value x(t) = x(to + At) with the Euler approximation x1 = xo + f(x.)At. a. Expand x(t) x(to + At) as a Taylor series in At, through terms of O(Ata). Express your answer solely in terms of X., At, and f and its derivatives at xo. b. Show that the local error (x(t) x1| ~ C(At)2 and give and explicit expression for the constant C. Generally, we are more interested in the global error incurred after integrating over a time interval of fixed length T nAt. Since each step produces an O(Ata) error, and we take n =T/At = O(At-1) steps, the global error |X(tn) xn| is O(At). 7. Bonus problem (it means you don't have to solve it): if you want to do a massive amount of algebra, try to redo problem 5 for the 4th-order Runge-Kutta method! It'll be a nightmare if you don't use Mathematica or some other symbolic manipulation language 5. In this problem, you'll use Taylor series expansions to estimate the error in taking one step by the Euler method. The exact solution and the Euler approximation both start at x = x, when t = to. We want to compare the exact value x(t) = x(to + At) with the Euler approximation x1 = xo + f(x.)At. a. Expand x(t) x(to + At) as a Taylor series in At, through terms of O(Ata). Express your answer solely in terms of X., At, and f and its derivatives at xo. b. Show that the local error (x(t) x1| ~ C(At)2 and give and explicit expression for the constant C. Generally, we are more interested in the global error incurred after integrating over a time interval of fixed length T nAt. Since each step produces an O(Ata) error, and we take n =T/At = O(At-1) steps, the global error |X(tn) xn| is O(At)