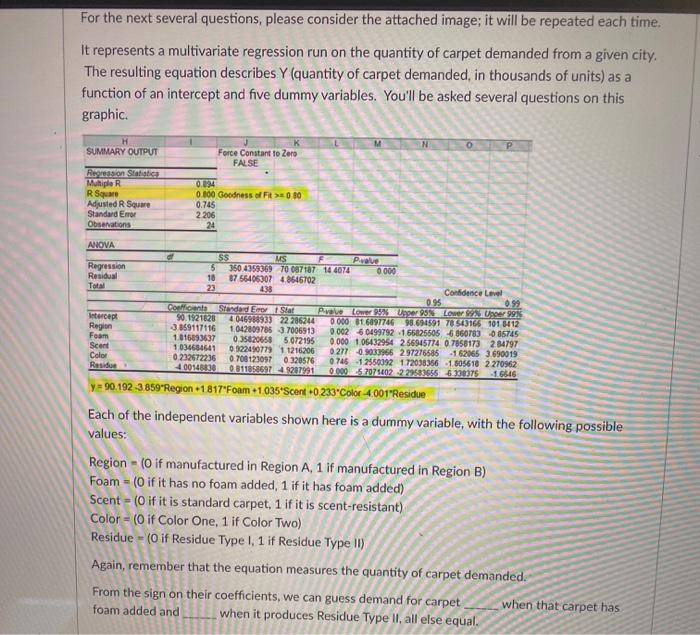
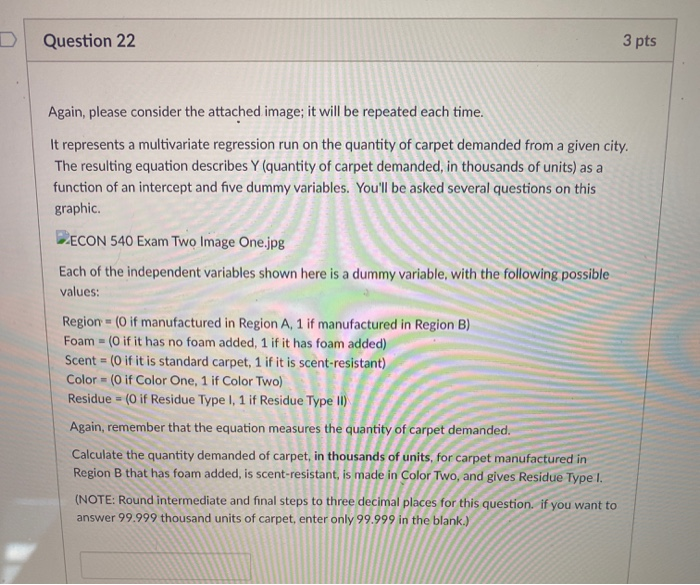
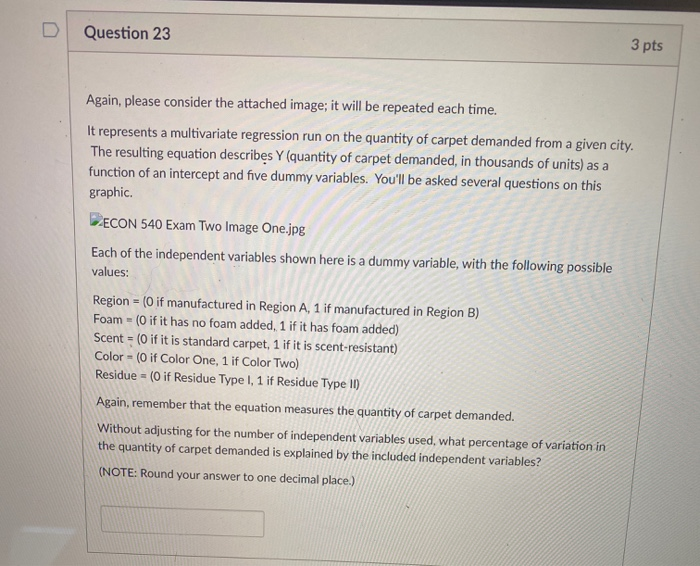
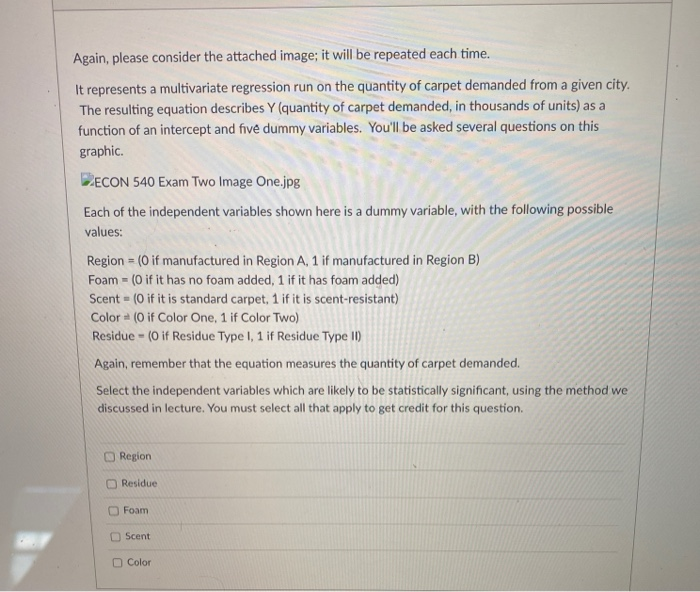
For the next several questions, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic. M N H SUMMARY OUTPUT 0 P Force Constant to Zero FALSE Regression Statistics Multiple R R Square Adjusted R Square Standard Error Observations 0.094 0.800 Goodness of Ft - 0 80 0.745 2 206 24 ANOVA SS MS F Pave Regression 5 350 4359369 70 087187 14 4074 0.000 Residual 10 87.56406307 4.8646702 Total 23 433 Confidence Level 0.95 0.99 Conflict Standard Emo Star Prove Lower 95% Upper 95% Lower Upper 99% Intercept 90 1921828 4046938933 22286244 0.000 1.6097746 98.694591 78.543166 101.8412 Region -3.859117116 1.042809786 3.7006913 0.0026 0499792.166825505 -6 860783 085745 Foam 1.816093637 0.35820658 5072195 0.000 1.06432954 2 56945774 0.7858173 284797 Scent 1.034684641 0.922490779 1.1216206 0277 0.9033966 297276585 -162065 3.690019 Color 0232672236 0.708123097 0.328576 0.746 -1 2550092 172038366 -1.805618 2 270962 Residue 400148838 001165869749287991 0.000 25.7071402 2 295836556 308375 -1.6545 y = 90.192 3.859"Region 1817'Foam 1.035'Scent +0 233Color4.001"Residue Each of the independent variables shown here is a dummy variable, with the following possible values: Region - (O if manufactured in Region A, 1 if manufactured in Region B) Foam = ( if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. From the sign on their coefficients, we can guess demand for carpet when that carpet has foam added and when it produces Residue Type II, all else equal. O is higher; is higher O is lower; is lower O is lower; is higher O is higher; is lower Question 22 3 pts Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region - ( if manufactured in Region A, 1 if manufactured in Region B) Foam - ( if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue = (if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Calculate the quantity demanded of carpet, in thousands of units, for carpet manufactured in Region B that has foam added, is scent-resistant, is made in Color Two, and gives Residue Type I. (NOTE: Round intermediate and final steps to three decimal places for this question. if you want to answer 99.999 thousand units of carpet, enter only 99.999 in the blank.) Question 23 3 pts Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic. DECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region = (0 if manufactured in Region A, 1 if manufactured in Region B) Foam - (O if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color - (O if Color One, 1 if Color Two) Residue = (0 if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Without adjusting for the number of independent variables used, what percentage of variation in the quantity of carpet demanded is explained by the included independent variables? (NOTE: Round your answer to one decimal place.) Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region = ( if manufactured in Region A. 1 if manufactured in Region B) Foam = ( if it has no foam added, 1 if it has foam added) Scent = 10 if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Select the independent variables which are likely to be statistically significant, using the method we discussed in lecture. You must select all that apply to get credit for this question. Region Residue Foam Scent Color D Question 25 3 pts Again, please consider the attached image; it will be repeated each time. This is the last time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region - ( if manufactured in Region A, 1 if manufactured in Region B) Foam - ( if it has no foam added, 1 if it has foam added) Scent - ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. What critical value will you use to perform an F-test on this calculation? Assume that we use a 95% confidence level (an alpha of 0.05). (NOTE: Enter only the critical value. Round to two decimal places.) For the next several questions, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic. M N H SUMMARY OUTPUT 0 P Force Constant to Zero FALSE Regression Statistics Multiple R R Square Adjusted R Square Standard Error Observations 0.094 0.800 Goodness of Ft - 0 80 0.745 2 206 24 ANOVA SS MS F Pave Regression 5 350 4359369 70 087187 14 4074 0.000 Residual 10 87.56406307 4.8646702 Total 23 433 Confidence Level 0.95 0.99 Conflict Standard Emo Star Prove Lower 95% Upper 95% Lower Upper 99% Intercept 90 1921828 4046938933 22286244 0.000 1.6097746 98.694591 78.543166 101.8412 Region -3.859117116 1.042809786 3.7006913 0.0026 0499792.166825505 -6 860783 085745 Foam 1.816093637 0.35820658 5072195 0.000 1.06432954 2 56945774 0.7858173 284797 Scent 1.034684641 0.922490779 1.1216206 0277 0.9033966 297276585 -162065 3.690019 Color 0232672236 0.708123097 0.328576 0.746 -1 2550092 172038366 -1.805618 2 270962 Residue 400148838 001165869749287991 0.000 25.7071402 2 295836556 308375 -1.6545 y = 90.192 3.859"Region 1817'Foam 1.035'Scent +0 233Color4.001"Residue Each of the independent variables shown here is a dummy variable, with the following possible values: Region - (O if manufactured in Region A, 1 if manufactured in Region B) Foam = ( if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. From the sign on their coefficients, we can guess demand for carpet when that carpet has foam added and when it produces Residue Type II, all else equal. O is higher; is higher O is lower; is lower O is lower; is higher O is higher; is lower Question 22 3 pts Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region - ( if manufactured in Region A, 1 if manufactured in Region B) Foam - ( if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue = (if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Calculate the quantity demanded of carpet, in thousands of units, for carpet manufactured in Region B that has foam added, is scent-resistant, is made in Color Two, and gives Residue Type I. (NOTE: Round intermediate and final steps to three decimal places for this question. if you want to answer 99.999 thousand units of carpet, enter only 99.999 in the blank.) Question 23 3 pts Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic. DECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region = (0 if manufactured in Region A, 1 if manufactured in Region B) Foam - (O if it has no foam added, 1 if it has foam added) Scent = ( if it is standard carpet, 1 if it is scent-resistant) Color - (O if Color One, 1 if Color Two) Residue = (0 if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Without adjusting for the number of independent variables used, what percentage of variation in the quantity of carpet demanded is explained by the included independent variables? (NOTE: Round your answer to one decimal place.) Again, please consider the attached image; it will be repeated each time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region = ( if manufactured in Region A. 1 if manufactured in Region B) Foam = ( if it has no foam added, 1 if it has foam added) Scent = 10 if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. Select the independent variables which are likely to be statistically significant, using the method we discussed in lecture. You must select all that apply to get credit for this question. Region Residue Foam Scent Color D Question 25 3 pts Again, please consider the attached image; it will be repeated each time. This is the last time. It represents a multivariate regression run on the quantity of carpet demanded from a given city. The resulting equation describes Y (quantity of carpet demanded, in thousands of units) as a function of an intercept and five dummy variables. You'll be asked several questions on this graphic ECON 540 Exam Two Image One.jpg Each of the independent variables shown here is a dummy variable, with the following possible values: Region - ( if manufactured in Region A, 1 if manufactured in Region B) Foam - ( if it has no foam added, 1 if it has foam added) Scent - ( if it is standard carpet, 1 if it is scent-resistant) Color = ( if Color One, 1 if Color Two) Residue - ( if Residue Type I, 1 if Residue Type II) Again, remember that the equation measures the quantity of carpet demanded. What critical value will you use to perform an F-test on this calculation? Assume that we use a 95% confidence level (an alpha of 0.05). (NOTE: Enter only the critical value. Round to two decimal places.)