For this question, we will assume that all graphs have a vertex-set that is non-empty and...

 

Transcribed Image Text:

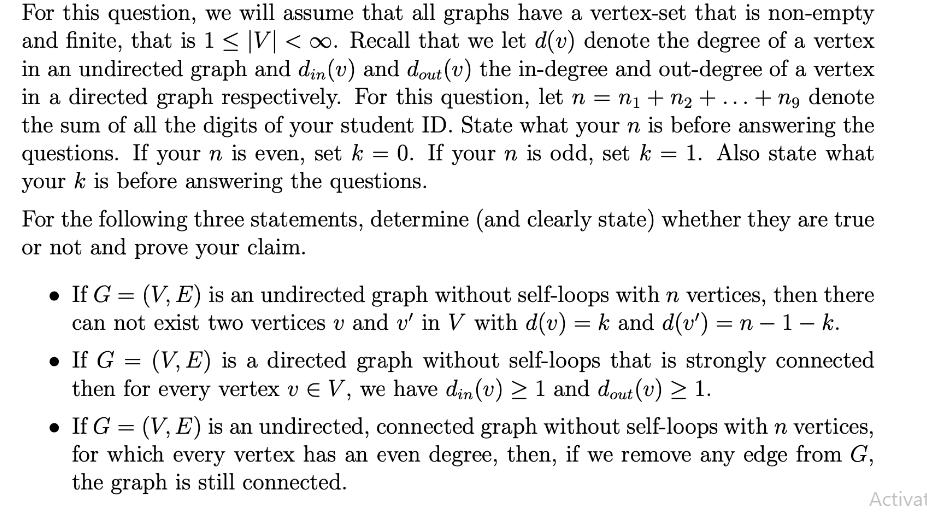
For this question, we will assume that all graphs have a vertex-set that is non-empty and finite, that is 1 < |V| < . Recall that we let d(v) denote the degree of a vertex in an undirected graph and din(v) and dout (v) the in-degree and out-degree of a vertex in a directed graph respectively. For this question, let n = n+n2 + ... + ng denote the sum of all the digits of your student ID. State what your n is before answering the questions. If your n is even, set k = 0. If your n is odd, set k = 1. Also state what your k is before answering the questions. For the following three statements, determine (and clearly state) whether they are true or not and prove your claim. If G = (V,E) is an undirected graph without self-loops with n vertices, then there can not exist two vertices v and v' in V with d(v) = k and d(v') = n 1 k. = (V,E) is a directed graph without self-loops that is strongly connected then for every vertex v V, we have din (v) 1 and dout (v) > 1. If G = (V,E) is an undirected, connected graph without self-loops with n vertices, for which every vertex has an even degree, then, if we remove any edge from G, the graph is still connected. Activat For this question, we will assume that all graphs have a vertex-set that is non-empty and finite, that is 1 < |V| < . Recall that we let d(v) denote the degree of a vertex in an undirected graph and din(v) and dout (v) the in-degree and out-degree of a vertex in a directed graph respectively. For this question, let n = n+n2 + ... + ng denote the sum of all the digits of your student ID. State what your n is before answering the questions. If your n is even, set k = 0. If your n is odd, set k = 1. Also state what your k is before answering the questions. For the following three statements, determine (and clearly state) whether they are true or not and prove your claim. If G = (V,E) is an undirected graph without self-loops with n vertices, then there can not exist two vertices v and v' in V with d(v) = k and d(v') = n 1 k. = (V,E) is a directed graph without self-loops that is strongly connected then for every vertex v V, we have din (v) 1 and dout (v) > 1. If G = (V,E) is an undirected, connected graph without self-loops with n vertices, for which every vertex has an even degree, then, if we remove any edge from G, the graph is still connected. Activat

Answer rating: 100% (QA)

To determine the values of n and k we need to calculate the sum of all the digits of the student ID ... View the full answer