Gather the data by following the instructions.

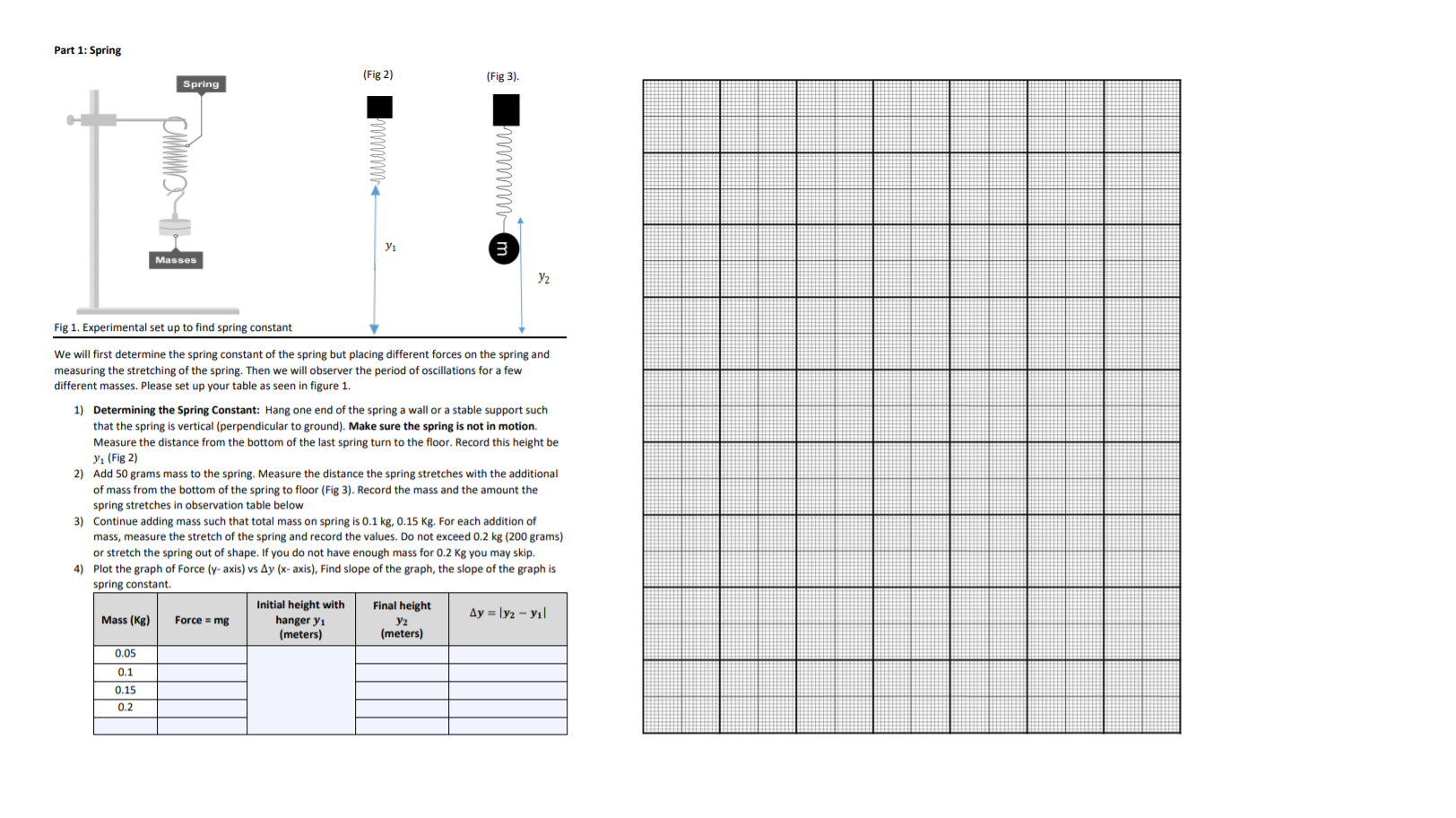
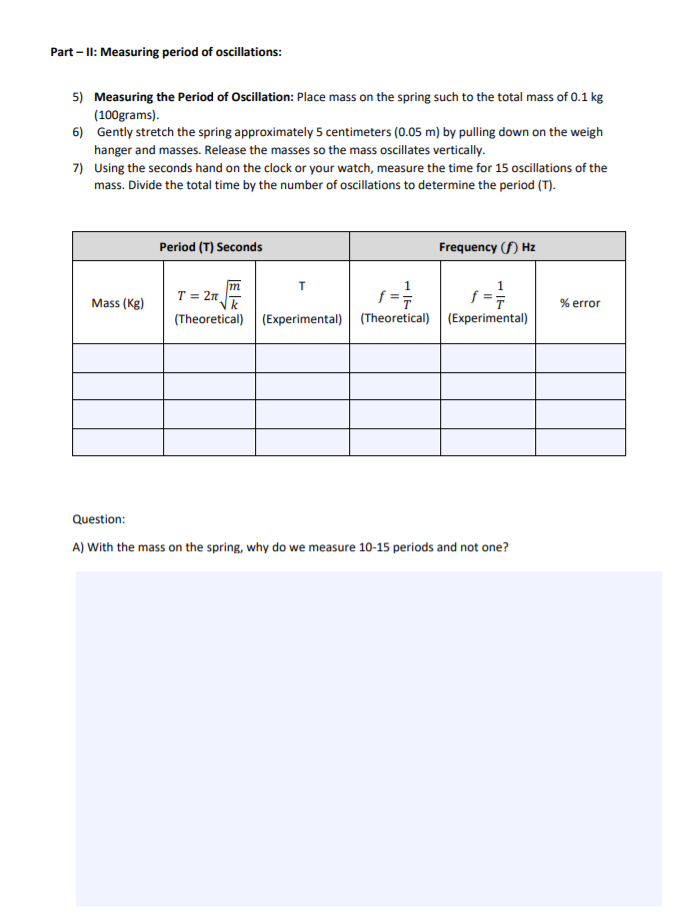
Part 1: Spring (Fig 2) Spring (Fig 3). V1 3 Masses y2 Fig 1. Experimental set up to find spring constant We will first determine the spring constant of the spring but placing different forces on the spring and measuring the stretching of the spring. Then we will observer the period of oscillations for a few different masses. Please set up your table as seen in figure 1 1) Determining the Spring Constant: Hang one end of the spring a wall or a stable support such that the spring is vertical (perpendicular to ground). Make sure the spring is not in motion. Measure the distance from the bottom of the last spring turn to the floor. Record this height be y1 (Fig 2) 2) Add 50 grams mass to the spring. Measure the distance the spring stretches with the additional of mass from the bottom of the spring to floor (Fig 3). Record the mass and the amount the spring stretches in observation table below 3) Continue adding mass such that total mass on spring is 0.1 kg, 0.15 Kg. For each addition of mass, measure the stretch of the spring and record the values. Do not exceed 0.2 kg (200 grams) or stretch the spring out of shape. If you do not have enough mass for 0.2 Kg you may skip. 4) Plot the graph of Force (y- axis) vs Ay (x- axis), Find slope of the graph, the slope of the graph is spring constant. Initial height with Final height Mass (Kg) Force = mg hanger y1 Ay = ly2 - yil V2 (meters) (meters) 0.05 0.1 0.15A periodic motion is one that repeats itself in a fairly regular way. Examples of periodic motion are a pendulum, a mass attached to a spring or even the orbit of the moon around the earth. The time it takes for one full motion, or cycle, is known as the period. For example, to complete one full cycle the moon requires approximately twenty-eight days. Thus the period of the moon is twenty-eight days. The period is normally represented by the letter (T). A quantity related to the period is the frequency (f). The frequency is the number of oscillations per second. The frequency and period are related by: f = Another quantity which describes the periodic motion is the amplitude. The amplitude is the maximum displacement from the equilibrium position of the motion. A particular type of periodic motion where the motion repeats in a sinusoidal way is called simple harmonic motion. Two examples of simple harmonic motion are the oscillations of a pendulum when the displacement is small and the oscillation of a mass on a spring. In this lab, simple harmonic motion will be examined using a mass on a spring and using pendulums. When a spring is stretched or compressed, the force required to stretch the spring is given by Hooke's Law: F = -kAx Where, k - spring constant, x - distance of the spring compressed or stretched. The spring constant determines the 'stiffness' of the spring and is measured in Newton/meter. The minus sign indicates the force points in the direction opposite to the displacement. The normal method of analyzing the motion of a mass on a spring using Newton's 2nd leads to a differential equation which is beyond the scope of this course. However, we can state the result for the period of a mass on a spring as: T = 2n Where, m - is the oscillating mass, k - Spring constant. A simple pendulum is a mass on a string or wire. The mass is assumed to be small in size. We assume the string or wire does not stretch. The period for a simple pendulum is given by: T = 2n Where, L - Length of the string, g - acceleration due to gravity It may be somewhat surprising that the mass does not enter into the equation for the period. We will verify this during this lab.Part - II: Measuring period at oscilhtions: 5} Measuring the Period of Oscillation: Place mass on the spring such to the total mass oi I11 kg anagrams]. Eu:- Gently.r stretch the spring approximately 5 centimeters [I105 m] by pulling down on the weigh hanger and masses. Release the masses so the mass oscillates vertical\"- ?} Using the seconds hand on the clock or your watch. measure the time for 1.5 oscillations of the mass. Divide the total time by the number of oscillations to determine the period (T). m T = Btu-J; 9:. error [Theoretical] Question: A} With the mass on the spring, why do we measure 10-15 periods and not one