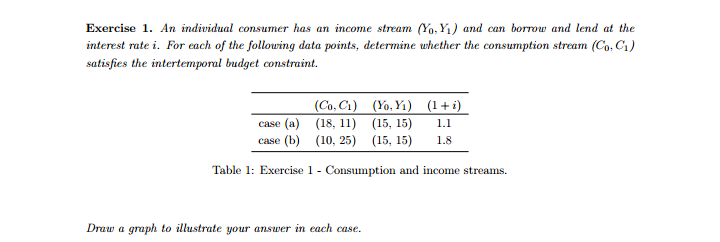
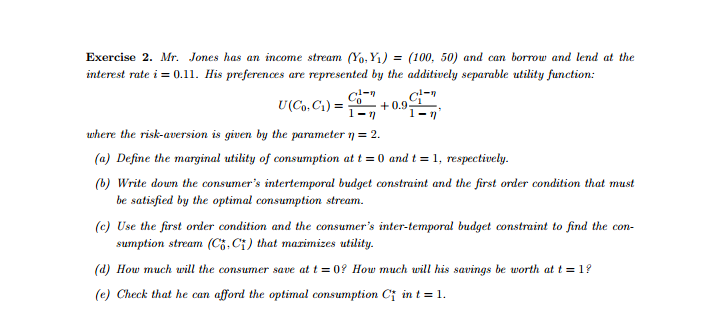
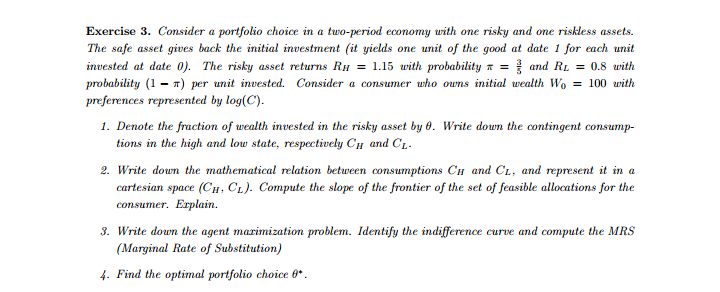
Get it done well in parts
1 Positive vs. Normative Statements (16 points) Identify whether each of the following statements is positive or normative. Briefly justify your answer. 1. (4 points) The government has a duty to provide basic healthcare and education to every citizen. 2. (4 points) The cost of health insurance is too high. 3. (4 points) The median earnings of a full-time worker with a college degree are almost twice as high as those of a high-school graduate with no college education. 4. (4 points) The current unemployment rate is 3.9%, the lowest it has been since December 2000.2 True or False (20 points) For each of the following statements, indicate if they are True or False. Justify your answer. 1. Bill is a football coach. He evaluates his players based on three criteria: height, strength, and speed. Bill prefers one player over another if he is better in at least two of these criteria. Assume that there are no players with the exact same height, nor the exact same strength, nor the exact same speed. (a) (4 points) Bill's preferences are complete. (b) (4 points) Bill's preferences are transitive. 2. (4 points) Ann and Bob are utility maximizing consumers. Given their income and market prices, Ann chooses a bundle that gives her a utility UAnn = 100, while Bob chooses a bundle that gives him a utility UBot = 110. Therefore, we know that Bob is happier than Ann. 3. (4 points) John's utility function for food (f) and clothes (c) is given by U (f, c) = (fo + co)=, with a > 0. John's preferences satisfy the principle of diminishing marginal rate of substitution for any value of a. 4. (4 points) Ava has preferences over two goods that satisfy completeness, transitive ity, non-satiation and the indifference curves have a strictly diminishing marginal rate of substitution. Suppose that the price of one of these goods increases (and the price of the other one remains the same). Claim: Ava's utlity must be strictly lower after the price increase. How does your answer depend on the composition of the initial consumption bundle?3 Demand for Video Games (16 points) We have the following weekly demand data for the video game Grand Theft Auto in a US town. We also have the price data for a Playstation at the same time. Price of Playstation (in $) |Price of Grand Theft Auto (in $) | Quantity of games demanded 300 10 100 310 10 95 320 10 90 300 11 99 310 96 1. (6 points) Write down the equation for the demand for Grand Theft Auto in the following form: QD =a+BP, + BpPp where Q2 and P, are quantity demanded and price of Grand Theft Auto, Pp is the price of Playstation, and o, 3., 8, are constants.4 Indifference Curves (18 points) In each of the following examples, the consumer consumes only two goods, a and y. Based on the information given in each statement, sketch a plausible set of indifference curves (draw at least two curves on a set of labeled axes and indicate the direction of higher utility). Then, write down a possible form of the utility function u(r, y) that is consistent with your graph. 1. (6 points) Alan likes wearing both right shoes (x) and left shoes (y). He always needs to wear them as a pair, having a right shoe is useless without the left one and viceversa.Exercise 1. An individual consumer has an income stream (Yo, Y1) and can borrow and lend at the interest rate i. For each of the following data points, determine whether the consumption stream (Co. Cl) satisfies the intertemporal budget constraint. (Co. C) (Yo,Yi) (1+i) CASE (A) (18, 11) (15, 15) 1.1 case (b) (10, 25) (15, 15) 1.8 Table 1: Exercise 1 - Consumption and income streams. Draw a graph to illustrate your answer in each case.Exercise 2. Mr. Jones has an income stream (Yo, Yi) = (100, 50) and can borrow and lend at the interest rate i = 0.11. His preferences are represented by the additively separable utility function: 1-77 U(Go. CI) = 1 - 1 + 0.9 where the risk-aversion is given by the parameter , = 2. (a) Define the marginal utility of consumption at t = 0 and t = 1, respectively. (b) Write down the consumer's intertemporal budget constraint and the first order condition that must be satisfied by the optimal consumption stream. (c) Use the first order condition and the consumer's inter-temporal budget constraint to find the con- sumption stream (C;. Ci) that maximizes utility. (d) How much will the consumer save at { = 0? How much will his savings be worth at t = 1? (e) Check that he can afford the optimal consumption Of int= 1.Exercise 3. Consider a portfolio choice in a two-period economy with one risky and one riskless assets. The safe asset gives back the initial investment (it yields one unit of the good at date I for each unit invested at date O). The risky asset returns Ry = 1.15 with probability a = _ and RL = 0.8 with probability (1 - x) per unit invested. Consider a consumer who owns initial wealth Wo = 100 with preferences represented by log(C). 1. Denote the fraction of wealth invested in the risky asset by 8. Write down the contingent consump- tions in the high and low state, respectively Cu and Cr- 2. Write down the mathematical relation between consumptions Cu and CL, and represent it in a cartesian space (Cu, CL). Compute the slope of the frontier of the set of feasible allocations for the consumer. Explain. 3. Write down the agent marimization problem. Identify the indifference curve and compute the MRS (Marginal Rate of Substitution) 4. Find the optimal portfolio choice 0*