Answered step by step
Verified Expert Solution
Question
1 Approved Answer
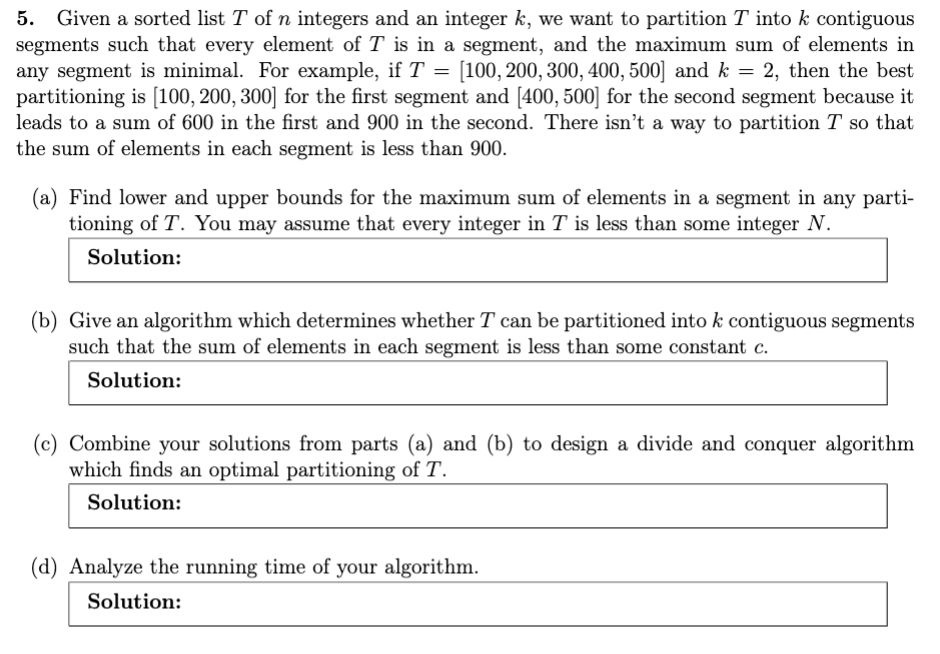
Given a sorted list T of n integers and an integer k , we want to partition T into k contiguous segments such that every
Given a sorted list of integers and an integer we want to partition into contiguous segments such that every element of is in a segment, and the maximum sum of elements in any segment is minimal. For example, if and then the best partitioning is for the first segment and for the second segment because it leads to a sum of in the first and in the second. There isn't a way to partition so that the sum of elements in each segment is less than
a Find lower and upper bounds for the maximum sum of elements in a segment in any partitioning of You may assume that every integer in is less than some integer
b Give an algorithm which determines whether can be partitioned into contiguous segments such that the sum of elements in each segment is less than some constant
c Combine your solutions from parts a and b to design a divide and conquer algorithm which finds an optimal partitioning of
d Analyze the running time of your algorithm.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


