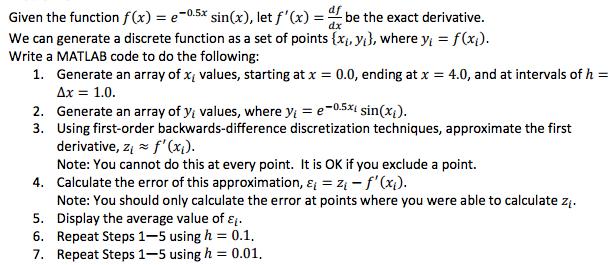
Given the function f(x) = e0.5x sin(x), let f'(x) = d be the exact derivative. dx We can generate a discrete function as a set of points (x, y), where y = f(x). Write a MATLAB code to do the following: 1. Generate an array of x, values, starting at x = 0.0, ending at x = 4.0, and at intervals of h = Ax = 1.0. 2. Generate an array of y values, where y = e0.5x+ sin(x). 3. Using first-order backwards-difference discretization techniques, approximate the first derivative, z f'(x). Note: You cannot do this at every point. It is OK if you exclude a point. 4. Calculate the error of this approximation, & = z = f'(x). Note: You should only calculate the error at points where you were able to calculate zi. 5. Display the average value of . 6. Repeat Steps 1-5 using h= 0.1. 7. Repeat Steps 1-5 using h= 0.01.