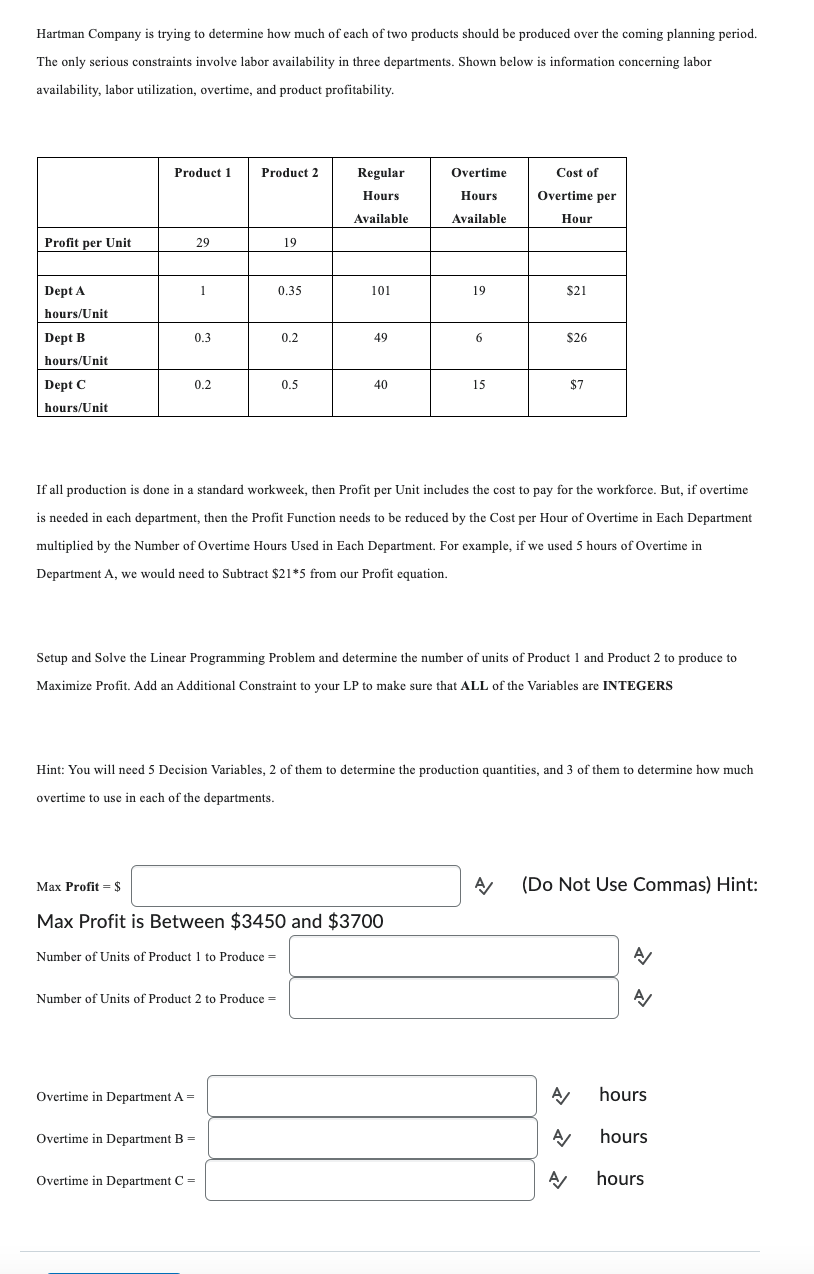
Hartman Company is trying to determine how much of each of two products should be produced over the coming planning period. The only serious constraints involve labor availability in three departments. Shown below is information concerning labor availability, labor utilization, overtime, and product profitability. If all production is done in a standard workweek, then Profit per Unit includes the cost to pay for the workforce. But, if overtime is needed in each department, then the Profit Function needs to be reduced by the Cost per Hour of Overtime in Each Department multiplied by the Number of Overtime Hours Used in Each Department. For example, if we used 5 hours of Overtime in Department A, we would need to Subtract $215 from our Profit equation. Setup and Solve the Linear Programming Problem and determine the number of units of Product 1 and Product 2 to produce to Maximize Profit. Add an Additional Constraint to your LP to make sure that ALL of the Variables are INTEGERS Hint: You will need 5 Decision Variables, 2 of them to determine the production quantities, and 3 of them to determine how much overtime to use in each of the departments. Max Profit =$ A (Do Not Use Commas) Hint: Max Profit is Between $3450 and $3700 Number of Units of Product 1 to Produce = Number of Units of Product 2 to Produce = Overtime in Department A= Overtime in Department B= Overtime in Department C= A. hours Hartman Company is trying to determine how much of each of two products should be produced over the coming planning period. The only serious constraints involve labor availability in three departments. Shown below is information concerning labor availability, labor utilization, overtime, and product profitability. If all production is done in a standard workweek, then Profit per Unit includes the cost to pay for the workforce. But, if overtime is needed in each department, then the Profit Function needs to be reduced by the Cost per Hour of Overtime in Each Department multiplied by the Number of Overtime Hours Used in Each Department. For example, if we used 5 hours of Overtime in Department A, we would need to Subtract $215 from our Profit equation. Setup and Solve the Linear Programming Problem and determine the number of units of Product 1 and Product 2 to produce to Maximize Profit. Add an Additional Constraint to your LP to make sure that ALL of the Variables are INTEGERS Hint: You will need 5 Decision Variables, 2 of them to determine the production quantities, and 3 of them to determine how much overtime to use in each of the departments. Max Profit =$ A (Do Not Use Commas) Hint: Max Profit is Between $3450 and $3700 Number of Units of Product 1 to Produce = Number of Units of Product 2 to Produce = Overtime in Department A= Overtime in Department B= Overtime in Department C= A. hours