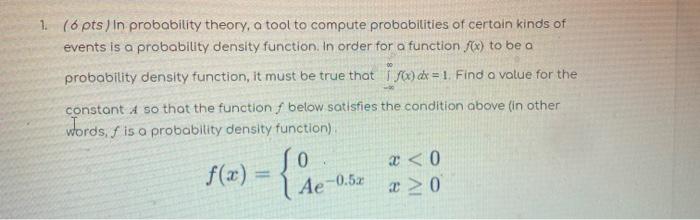
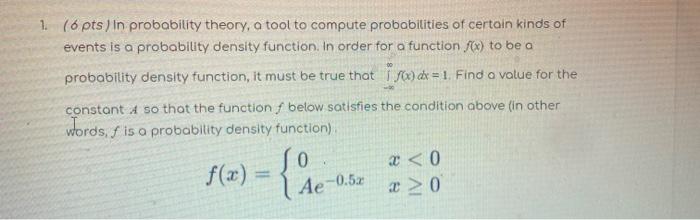
Question
he gathering of particles in a suspension is 50 for every mL. A 5 mL volume of the suspension is taken out. a. What is

he gathering of particles in a suspension is 50 for every mL. A 5 mL volume of the
suspension is taken out.
a. What is the probability that the amount of particles eliminated will be among 235 and
265?
b. What is the probability that the typical number of particles per mL in the draw out model
is some place in the scope of 48 and 52?
c. If a 10 mL test is taken out, what is the probability that the typical number per mL
of particles in the eliminated model is some place in the scope of 48 and 52?
d. How gigantic a model ought to be taken out with the objective that the ordinary number of particles per mL in
the model is some place in the scope of 48 and 52 with probability 95%?
In a self-assertive illustration of 100 batteries made by a particular procedure, the typical
lifetime was 150 hours and the standard deviation was 25 hours.
(I) Find a 95% conviction length for the mean lifetime of batteries made by this
model.
(ii) Find a 99% conviction length for the mean lifetime of batteries made by this
model.
(iii) An originator states that the mean lifetime is some place in the scope of 147 and 153 hours. With what
level of conviction can this statement is made?
(iv) Approximately the quantity of batteries ought to be examined with the objective that a 95% assurance
stretch will demonstrate the expect to inside 2 hours?
(v) Approximately the quantity of batteries ought to be analyzed so a 99% assurance
stretch will decide the plan to inside 2 hours
A Company researcher has been given the task of social affair information practically all agents
inside the association to all the more promptly appreciate the sufficiency of their high level training pathway
program offered to all delegates. The probability that a specialist has been at the association
at any rate five years is 0.74, the probability that a delegate has a Master's level testament or
higher is 0.34, and the probability that a subjectively picked specialist has been at the association
at any rate five years and has a Master's authentication is 0.12.
F. Of the people who have an advanced education 35.29% have been at the association at any rate 5 years
while those with lower degrees 93.94 % have been at the association at any rate 5 years. Make a probability tree depicting the current condition.
Much thanks to you benevolent for reacting to me. Would you have the option to compassionately answer the (iv) and (v) sub parts.
In an unpredictable illustration of 100 batteries conveyed by a particular methodology, the ordinary
lifetime was 150 hours and the standard deviation was 25 hours.
(I) Find a 95% assurance range for the mean lifetime of batteries made by this
model.
(ii) Find a 99% assurance range for the mean lifetime of batteries made by this
model.
i21i
(iii) A planner ensures that the mean lifetime is some place in the scope of 147 and 153 hours. With what
level of assurance can this affirmation is made?
(iv) Approximately the quantity of batteries ought to be tried with the objective that a 95% conviction
length will decide the expect to inside 2 hours?
(v) Approximately the quantity of batteries ought to be tried with the objective that a 99% conviction
length will decide the plan to inside 2 hours?


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


