Hello,

Appreciate if you can help me to find out the solution for the following problem.

Denote by V(t)V(t) the replicating portfolio of the payoff in problem number 4 (in this unit's practice problems and problem set) that does replication by investing the domestic currency in the foreign stock, the foreign bank account and the domestic bank account. Can you say, in terms of V(t)V(t), how much the replicating portfolio invests in each of these three assets, in each of the above two problems?

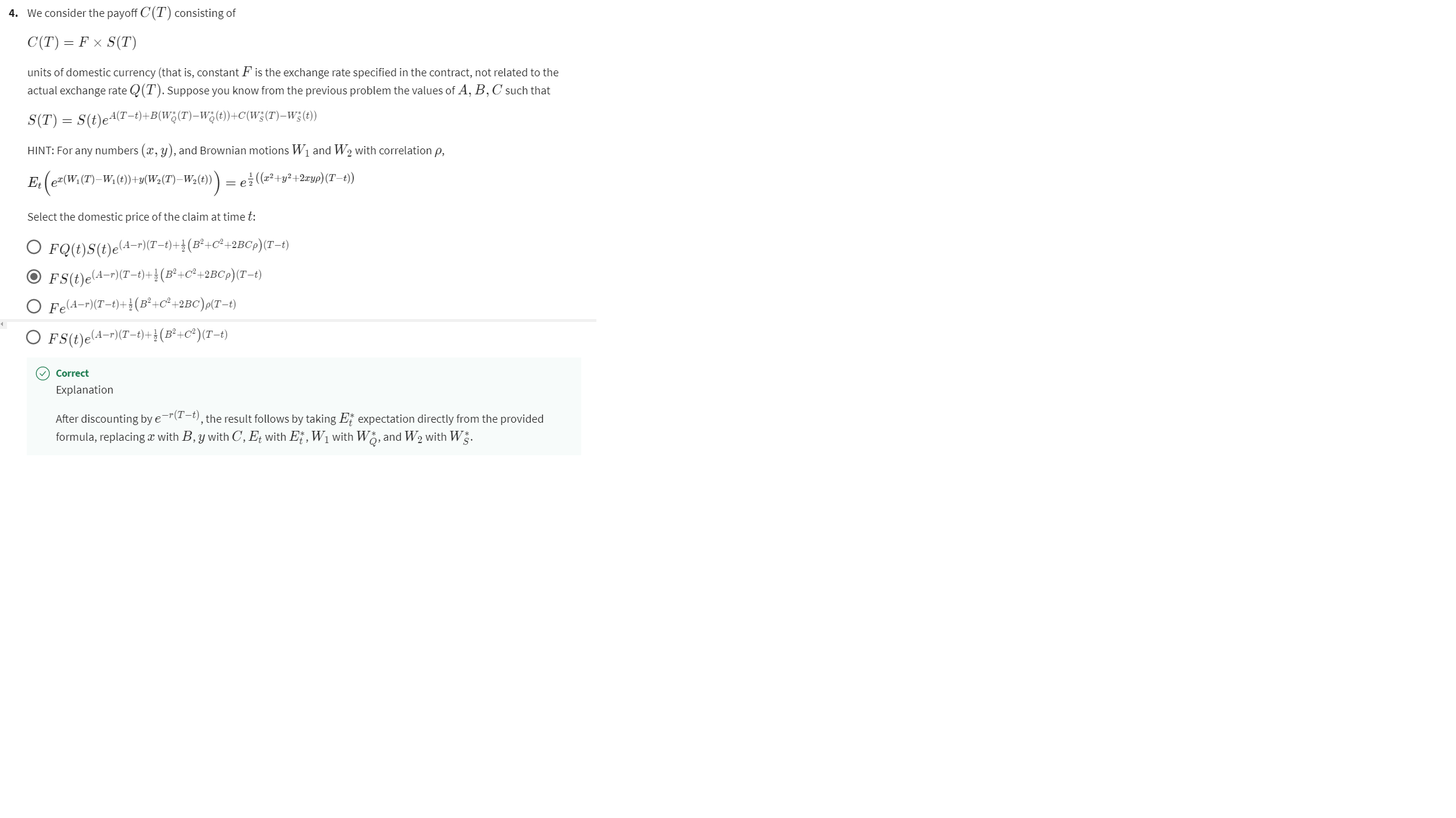
I am attaching the problem 4 of unit's practice problemsand its solution in UnitPracticeProblemAndSol.png

4. We consider the payoff C(T) in dollars in the amount C(T) = log (VZ(T) ) , where Z (t) is the price in dollars of the European stock at time t. Suppose you know from the previous problem the values of A, B, C such that Z (T) = Q(T)S(T) = Q(t)S(t)ed(T-t)+B(Wi (T)-Wi (t))+C(W; (T)-W; (t)) The price of the claim C(T) is equal to: O e-r( T-t) Q (t) S(t) N(d]) O e-r(T-t) (; log Q (t) S(t ) + } A(T - t) + } (B2 + C2) (T -t) ) O e-7(I-1) } log Q(t) S(t) O e-T(T-t) (; log Q (t) S(t) + ; A(T -t) ) Correct Explanation The price should be computed under the probabilities found in the previous problem, because under those probabilities the dollar values of the discounted foreign bank account and the discounted foreign stock are martingales. We have log VZ(T) = log Q (t) S(t ) + 3 (A(T - t) + B(Wi (T) - Wi (t)) + C(W2 (T) - W2 (t)) ) The price is the Et expectation of the discounted value of this, thus equal to e-r(I-t) ($ log Q(t) S(t) + ; A(T -t))4. We consider the payoff C(T') consisting of C(T) = F X S(T) units of domestic currency (that is, constant F is the exchange rate specified in the contract, not related to the actual exchange rate Q (1'). Suppose you know from the previous problem the values of A, B, C such that S(T) = S(t)e4(T-t)+B(W.(T)-Wa(t))+C(W;(T)-W's(t)) HINT: For any numbers (x, y), and Brownian motions W1 and W2 with correlation p, Et ( ex( W. (T) -W. (t)+u( W2 (T)-W=(t)) ) = et((22+32+ 2xup) (T-1)) Select the domestic price of the claim at time t: O FQ(t)S(t) e(4-r) (I-t) +4(B2+02+2BCP) (I-t) O FS(t)e(4-r)(T-t)+}(B'+02+2BCP)(I-t) O Fe(A-r)(T-t)+1 (B'+02+2BC)p(T-t) O FS(t) e (4-r)(T-t) + 1 (B' + C? ) (I-t) Correct Explanation After discounting by er(1-t), the result follows by taking Et expectation directly from the provided formula, replacing a with B, y with C, Et with Et, Wi with Wa, and W2 with Ws