Hello, please help me with this I cannot figure It out. I attached some files that may help you solve it.

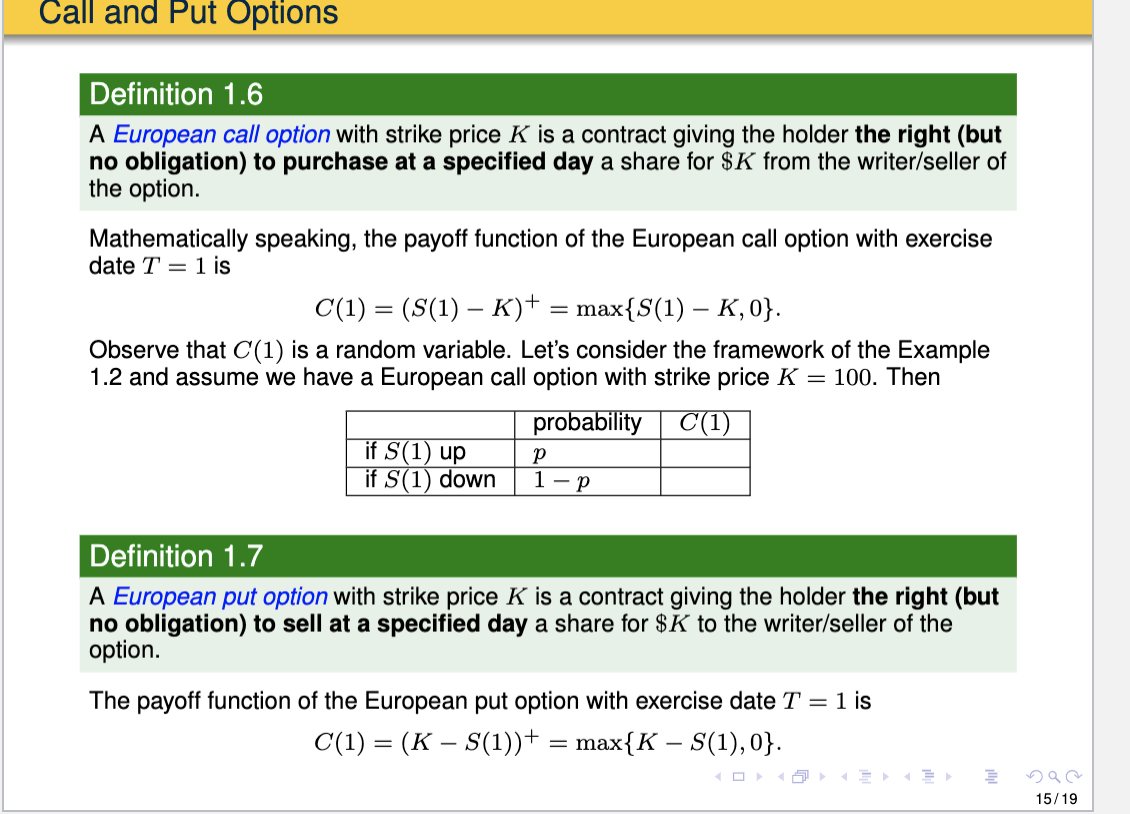
Problem 1. (10 Points) Assume that the price of the stock S follows the one-step binomial model with S(0) = $100 and S(1) = Su $105, if S goes up = $98, if S goes down sd Moreover, we have P({S(1) = SU}) 1 3 The bond prices are A(0) = $100 and A(1) = $103. We are interested in pricing a European call and a European put with strike price K = 103 and exercise time is T = 1. (a) (2 Points) Verify that there are no arbitrage opportunities in the market {S, B}. (b) (8 Points) Determine the replicating portfolios Vc and Vp, where Vc, resp. Vp, is the replicating portfolio of the European call, resp. put. Proposition 1.1 Under the framework of the One-Step Binomial model, assume (for simplicity) that S(0) = A(O). Then, there is No Arbitrage opportunity only if sd S4. In other words, we prefer to hold at time 1 the bond. Then, at time t = 0: (i) short sell 1 share; i.e., receive $S(0) and (ii) buy 1 bond. The total outcome is $0. One-Step Binomial Model Proof. Wait until time t=1 when (i) you collect $A(1) from the bond, (ii) buy one share for S(1) and return the share to the lender. The total outcome is, based on our initial assumption, A(1) S(1) > 0. In other words, we have a certain strictly positive profit with zero initial investment Consider the case A(1) 0 7, -50+ 51) =-5d+s4 >0 Vlo) = 0, V(1) 20 with probalxility tB20 - A.O 410) ): I share stock and S60) Case 2: If you 1 sl bands. The time o value of this portfolio is Alo) vo) = 1.500) Slo) 410) Alo)=0. At time I, ,5" A) the value will become: Slo) Ao slo), when stock pink V(i) = 1.5(1) - 46) A1 = A. 510), when stock price & -> VO) = 5"- 40-560) with Probability P, 830 075" Sto) Slo) - sursd 70. v (o)=0, V(1) 70 with probability pro 4.0. 410) ): I share stock and S60) Case 2: If you 1 sl bands. The time o value of this portfolio is Alo) vo) = 1.500) Slo) 410) Alo)=0. At time I, ,5" A) the value will become: Slo) Ao slo), when stock pink V(i) = 1.5(1) - 46) A1 = A. 510), when stock price & -> VO) = 5"- 40-560) with Probability P, 830 075" Sto) Slo) - sursd 70. v (o)=0, V(1) 70 with probability pro 4.0. : Put option pricing Recall: By definition, the put option at time I has value stock price 1. PC1) = (k~50) - los stock price d. 10 Stefi Replicate this put option with x shares and 4 bands - X.511)+ Y AC1)=P(1) poxtlloy = 0 90x+lloy = 10 - Ty: 4 :- 4 es 2: Pricing the option. PO) = 0.560) + 4.460) = 5:100+ #. 6023.03 Call and Put Options Definition 1.6 A European call option with strike price K is a contract giving the holder the right (but no obligation) to purchase at a specified day a share for $K from the writer/seller of the option. Mathematically speaking, the payoff function of the European call option with exercise date T = 1 is C(1) = (S(1) K)+ = max{S(1) K,0}. Observe that C(1) is a random variable. Let's consider the framework of the Example 1.2 and assume we have a European call option with strike price K = 100. Then probability C(1) if S(1) up if S(1) down 1-P Definition 1.7 A European put option with strike price K is a contract giving the holder the right (but no obligation) to sell at a specified day a share for $K to the writer/seller of the option. The payoff function of the European put option with exercise date T = 1 is C(1) = (K S(1))+ = max{K S(1),0}. nae 15/19 Problem 1. (10 Points) Assume that the price of the stock S follows the one-step binomial model with S(0) = $100 and S(1) = Su $105, if S goes up = $98, if S goes down sd Moreover, we have P({S(1) = SU}) 1 3 The bond prices are A(0) = $100 and A(1) = $103. We are interested in pricing a European call and a European put with strike price K = 103 and exercise time is T = 1. (a) (2 Points) Verify that there are no arbitrage opportunities in the market {S, B}. (b) (8 Points) Determine the replicating portfolios Vc and Vp, where Vc, resp. Vp, is the replicating portfolio of the European call, resp. put. Proposition 1.1 Under the framework of the One-Step Binomial model, assume (for simplicity) that S(0) = A(O). Then, there is No Arbitrage opportunity only if sd S4. In other words, we prefer to hold at time 1 the bond. Then, at time t = 0: (i) short sell 1 share; i.e., receive $S(0) and (ii) buy 1 bond. The total outcome is $0. One-Step Binomial Model Proof. Wait until time t=1 when (i) you collect $A(1) from the bond, (ii) buy one share for S(1) and return the share to the lender. The total outcome is, based on our initial assumption, A(1) S(1) > 0. In other words, we have a certain strictly positive profit with zero initial investment Consider the case A(1) 0 7, -50+ 51) =-5d+s4 >0 Vlo) = 0, V(1) 20 with probalxility tB20 - A.O 410) ): I share stock and S60) Case 2: If you 1 sl bands. The time o value of this portfolio is Alo) vo) = 1.500) Slo) 410) Alo)=0. At time I, ,5" A) the value will become: Slo) Ao slo), when stock pink V(i) = 1.5(1) - 46) A1 = A. 510), when stock price & -> VO) = 5"- 40-560) with Probability P, 830 075" Sto) Slo) - sursd 70. v (o)=0, V(1) 70 with probability pro 4.0. 410) ): I share stock and S60) Case 2: If you 1 sl bands. The time o value of this portfolio is Alo) vo) = 1.500) Slo) 410) Alo)=0. At time I, ,5" A) the value will become: Slo) Ao slo), when stock pink V(i) = 1.5(1) - 46) A1 = A. 510), when stock price & -> VO) = 5"- 40-560) with Probability P, 830 075" Sto) Slo) - sursd 70. v (o)=0, V(1) 70 with probability pro 4.0. : Put option pricing Recall: By definition, the put option at time I has value stock price 1. PC1) = (k~50) - los stock price d. 10 Stefi Replicate this put option with x shares and 4 bands - X.511)+ Y AC1)=P(1) poxtlloy = 0 90x+lloy = 10 - Ty: 4 :- 4 es 2: Pricing the option. PO) = 0.560) + 4.460) = 5:100+ #. 6023.03 Call and Put Options Definition 1.6 A European call option with strike price K is a contract giving the holder the right (but no obligation) to purchase at a specified day a share for $K from the writer/seller of the option. Mathematically speaking, the payoff function of the European call option with exercise date T = 1 is C(1) = (S(1) K)+ = max{S(1) K,0}. Observe that C(1) is a random variable. Let's consider the framework of the Example 1.2 and assume we have a European call option with strike price K = 100. Then probability C(1) if S(1) up if S(1) down 1-P Definition 1.7 A European put option with strike price K is a contract giving the holder the right (but no obligation) to sell at a specified day a share for $K to the writer/seller of the option. The payoff function of the European put option with exercise date T = 1 is C(1) = (K S(1))+ = max{K S(1),0}. nae 15/19