Hello tutors..

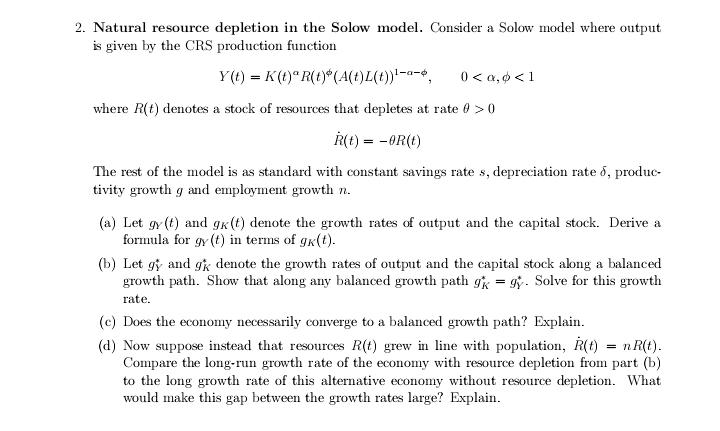
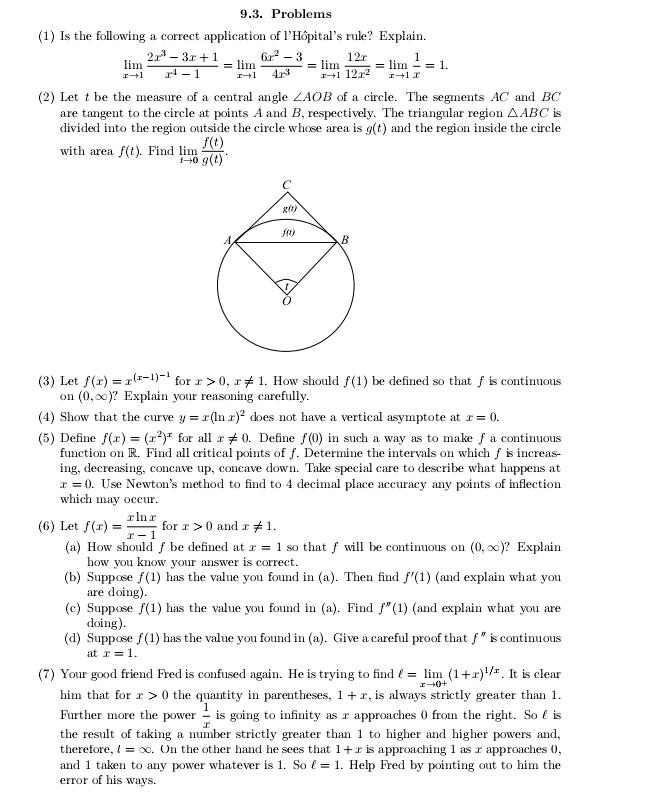
1.2. Exercises (1) The inequality |r - 2| () R(t) = -OR(t) The rest of the model is as standard with constant savings rate s, depreciation rate 6, produc- tivity growth g and employment growth n. (a) Let gy (t) and ge(t) denote the growth rates of output and the capital stock. Derive a formula for gy (t) in terms of gx(t). (b) Let of and g denote the growth rates of output and the capital stock along a balanced growth path. Show that along any balanced growth path g; = g. Solve for this growth rate. (c) Does the economy necessarily converge to a balanced growth path? Explain. (d) Now suppose instead that resources R(t) grew in line with population, R(() = n R(t). Compare the long-run growth rate of the economy with resource depletion from part (b) to the long growth rate of this alternative economy without resource depletion. What would make this gap between the growth rates large? Explain.9.3. Problems (1) Is the following a correct application of l'Hopital's rule? Explain. lim 2x - 3r+ 1 6r- - 3 12r = lim = lim 1-+1 r - = lim - = 1. I-+1 473 r +1 1212 (2) Let t be the measure of a central angle ZAOB of a circle. The segments AC and BC are tangent to the circle at points A and B, respectively. The triangular region AABC is divided into the region outside the circle whose area is g(t) and the region inside the circle with area f(t). Find lim /(t) fog(t) (3) Let f(r) = p(-1)" for r > 0, r# 1. How should f(1) be defined so that f is continuous on (0,co)? Explain your reasoning carefully. (4) Show that the curve y = r(In r) does not have a vertical asymptote at r = 0). (5) Define f(x) = (r')" for all r # 0. Define f(0) in such a way as to make f a continuous function on R. Find all critical points of f. Determine the intervals on which f is increase ing, decreasing, concave up, concave down. Take special care to describe what happens at r =0. Use Newton's method to find to 4 decimal place accuracy any points of inflection which may occur. (6) Let f (r) = = r- 1 for r > 0 and r # 1. (a) How should f be defined at r = 1 so that f will be continuous on (0, co)? Explain how you know your answer is correct. (b) Suppose f(1) has the value you found in (a). Then find f'(1) (and explain what you are doing). (c) Suppose f(1) has the value you found in (a). Find f*(1) (and explain what you are doing). (d) Suppose f (1) has the value you found in (a). Give a careful proof that f * is continuous at r =1. (7) Your good friend Fred is confused again. He is trying to find / = lim (1 +r) /3. It is clear I-+0+ him that for r > 0 the quantity in parentheses, 1 + r, is always strictly greater than 1. Further more the power - 1 r _ is going to infinity as r approaches 0 from the right. So / is the result of taking a number strictly greater than 1 to higher and higher powers and, therefore, 1 = co. On the other hand he sees that 1 + r is approaching 1 as r approaches (, and 1 taken to any power whatever is 1. So / = 1. Help Fred by pointing out to him the error of his ways.11.2. Exercises (1) Let f(x) = p + 3x3 + x - 10. Then Df-1(48) = = where a = (2) Let /(x) = _ my for r 2 1. Then /-1(243) = -a 3" where a =_ and p = (3) Let /(x) = In(x - 2) +es for r > 2. 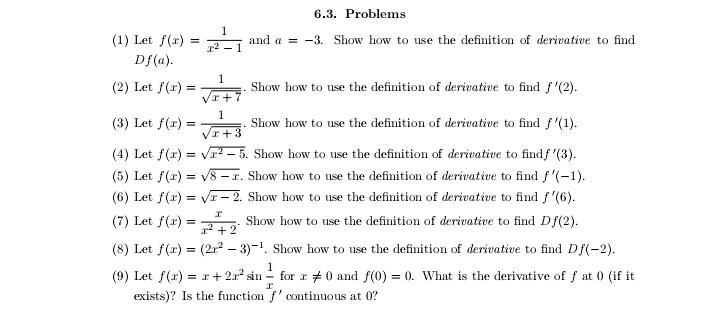Then Dy-1(e") = (1 + acb)-1 where a = - and b = (4) Let f (1) = 10 1 - 1 + for -1 1. Then Df-1(8) = = where a = (11) Let /(x) = In = +1 for x > 1. Then Df-!(In 5 - In3) = - where a = (12) What is the area of the largest rectangle that has one corner at the origin, one corner on the negative y-axis, one corner on the positive r-axis, and one corner on the curve y = Ina? Answer: the area is (13) What is the area of the largest rectangle that has one corner at the origin, one corner on the negative r-axis, one corner on the positive y-axis, and one corner on the curvey = et? Answer: the area is (14) Solve the equation: 1 + log,,(r - 4) = log,(2 + 5). Answer: 1= (15) Let /(x) = log,(log, r). Then Df(e) = where a = (16) Suppose p, q > 0 and logy(p) = log12(9) = logis(p + q). Find 2. Express your answer in a form that involves neither exponentials nor logarithms. Answer: ! _ + 4wh 2 where a = (17) A triangle is bounded by the r-axis, the y-axis, and the tangent line to the curve y = 2" at r = 0. The area of this triangle is - where a = (18) lim - = a lab where a = and b = 11.2. EXERCISES 71 (19) lim logs (t + 0.04) + 2 In 6 where a = and b = (20) If y = arcsin ( ), then dy dr Vi- H where a = and b = (21) A tapestry 30 feet high is hung so that its lower edge is 24 feet above the eye of an observer. How far from the tapestry should the observer stand in order to maximize the visual angle subtended by the tapestry? Answer: ft. (22) Let f(x) = arctan 17 7). Then DS(1) = = where a = (23) Let f(x) = arctan (). Then s () - " andr ()- -; where a - _ and b= (24) Let /(x) = parxing. Then f(1) = _ and f'(1) = (25) A solution to the equation arcsing - arccos r =0 is r = = where o = (20) Let /(x) = arctan 2r - arctan r for r 2 0. (a) The function / is increasing on the interval ( (b) The function / has a local maximum at s = (c) The function f has a local minimum at 1 = (27) Let / (x) = In(arctan vr- -1). Then S'(2) = V" where a = _ and b = (28) The expression e final is a complicated way of writing the integer (29) The expression In 81 (In 27) In 3v3 is a complicated way of writing the fraction s where (30) The solution to the differential equation y' = (2r- 1)y which satisfies the initial condition y(0) = 3 is y = el(?) where a = _and f(r) =_ (31) The solution to the differential equation y' = 4ry which satisfies the initial condition y(0) = 7 is y = ac/(") where a = _ and f(r) = _ (32) Let f(x) = er'timr for r > 0. Then Of (e) = - where a = (33) The equation of the tangent line at the point (1,0) to the curve whose equation is6.3. Problems 1 (1) Let f(r) and a = -3. Show how to use the definition of derivative to find Df (a). (2) Let f (r) = 1 Show how to use the definition of derivative to find f '(2). vr+7 (3) Let f(r): 1 Show how to use the definition of derivative to find f'(1). vr+3 (4) Let f(x) = vr- -5. Show how to use the definition of derivative to findf '(3). (5) Let f(r) = v8 - r. Show how to use the definition of derivative to find f'(-1). (6) Let f(r) = vr -2. Show how to use the definition of derivative to find f'(6). (7) Let f (x) = 2+ 2 Show how to use the definition of derivative to find Df(2). (8) Let f(r) = (2r' - 3) . Show how to use the definition of derivative to find Df(-2). (9) Let f(x) = r+2r- sin - for r # 0 and f(0) = 0. What is the derivative of f at 0 (if it exists)? Is the function f'continuous at (