Help me out. Provide clear solutions
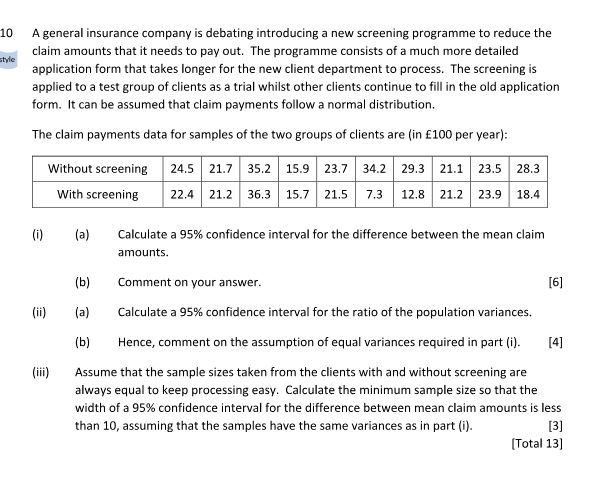
6 Heights of males with classic congenital adrenal hyperplasia (CAH) are assumed to be normally distributed. Determine the minimum sample size to ensure that a 95% confidence interval for the mean height has a maximum width of 10cm, if: a previous sample had a standard deviation of 8.4 cm the population standard deviation is 8.4 cm. 7 A sample value of 2 is obtained from a Poisson distribution with mean #. Calculate an exact two-sided 90% confidence interval for /- (ii) A sample of 30 values from the same Poisson distribution has a mean of 2. Use these data values to construct an approximate 90% confidence interval for / . 8 An office manager wants to analyse the variability in the time taken for her typists to complete a given task. She has given seven typists the task and the results are as follows (in minutes): 15, 17.2, 13.7, 11.2, 18, 15.1, 14 The manager wants a 95% confidence interval for the true standard deviation of time taken of the form (k, ). Calculate the value of k. 9 The amounts of individual claims arising under a certain type of general insurance policy are known from past experience to conform to a lognormal distribution in which the standard style deviation equals 1.8 times the mean. An actuary has found that the lower and upper limits of a 95% confidence interval for the mean claim amount are f4,250 and $4,750. Evaluate the lower and upper limits of a 95% confidence interval for the lognormal parameter //. [3]10 A general insurance company is debating introducing a new screening programme to reduce the claim amounts that it needs to pay out. The programme consists of a much more detailed tyle application form that takes longer for the new client department to process. The screening is applied to a test group of clients as a trial whilst other clients continue to fill in the old application form. It can be assumed that claim payments follow a normal distribution. The claim payments data for samples of the two groups of clients are (in (100 per year): Without screening 24.5 21.7 35.2 15.9 23.7 34.2 29.3 21.1 23.5 28.3 With screening 22.4 21.2 36.3 15.7 21.5 7.3 12.8 21.2 23.9 18.4 (a) Calculate a 95% confidence interval for the difference between the mean claim amounts. (b) Comment on your answer. [6] (ii) (a) Calculate a 95% confidence interval for the ratio of the population variances. (b) Hence, comment on the assumption of equal variances required in part (i). [4] (iii) Assume that the sample sizes taken from the clients with and without screening are always equal to keep processing easy. Calculate the minimum sample size so that the width of a 95% confidence interval for the difference between mean claim amounts is less than 10, assuming that the samples have the same variances as in part (i). [3] [Total 13]