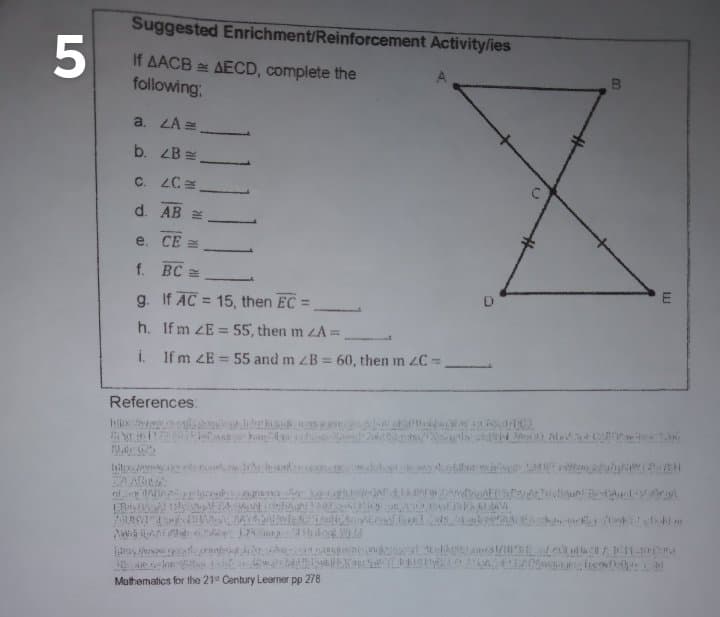
help me with this.
Geometry without the study of congruent triangles is like reading a book
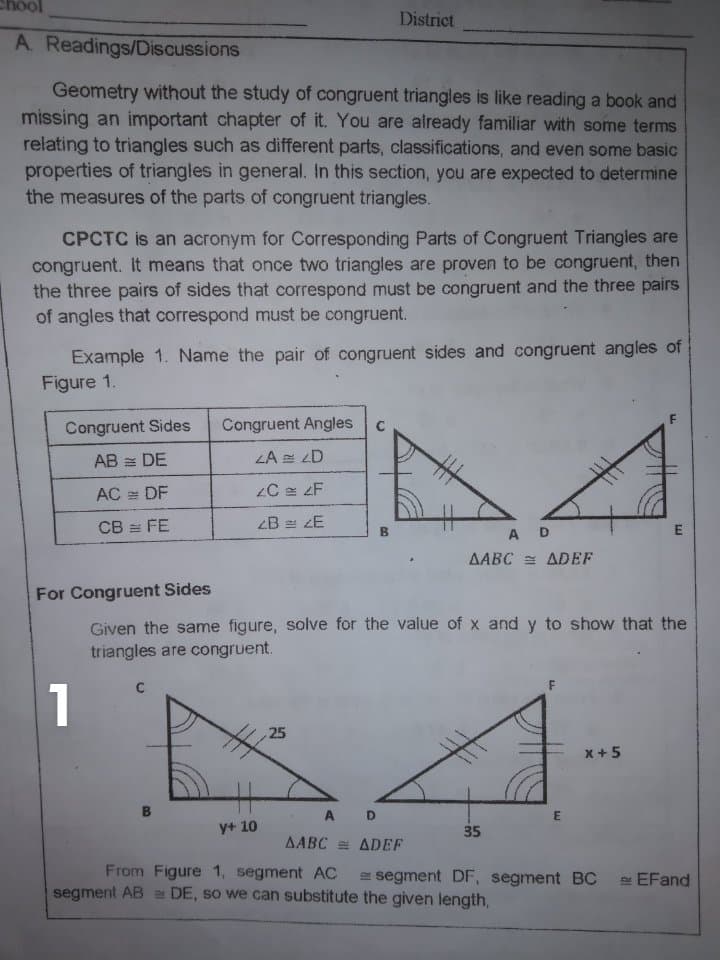
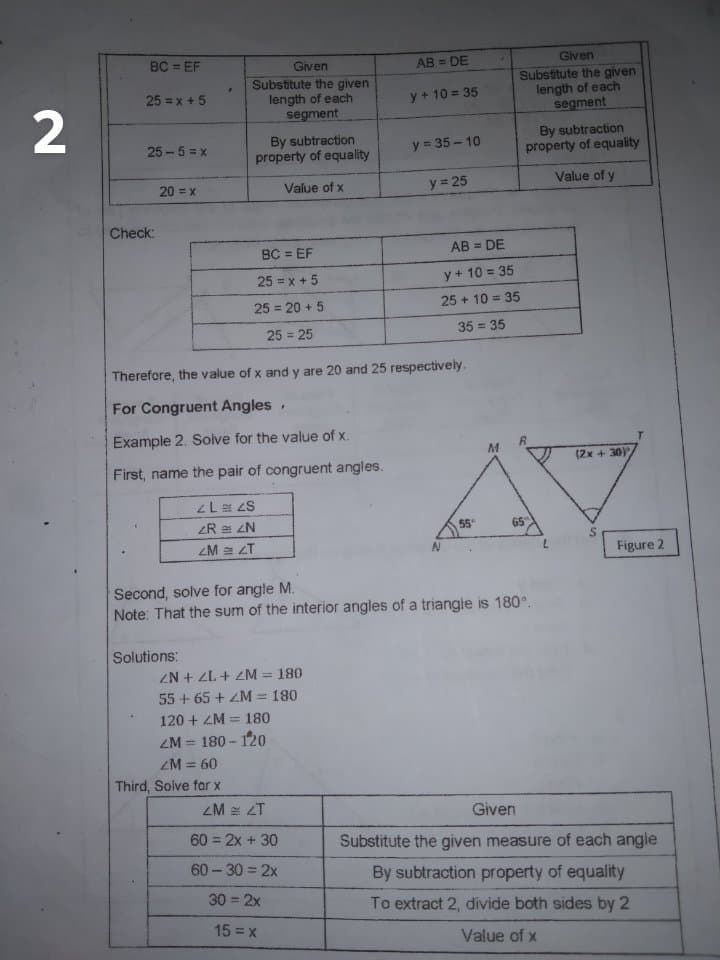
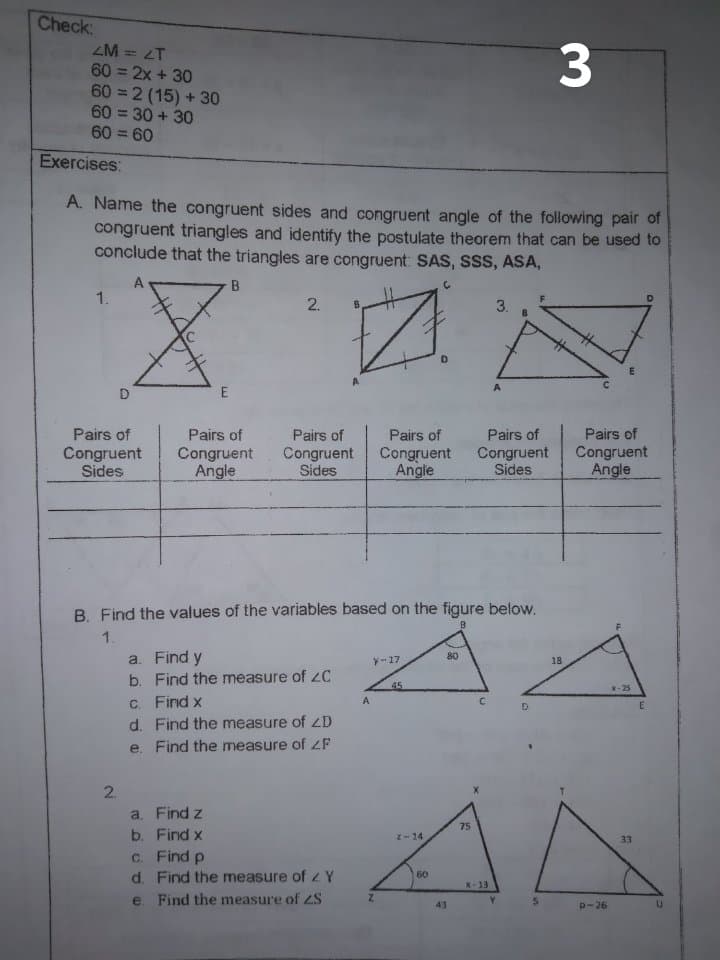
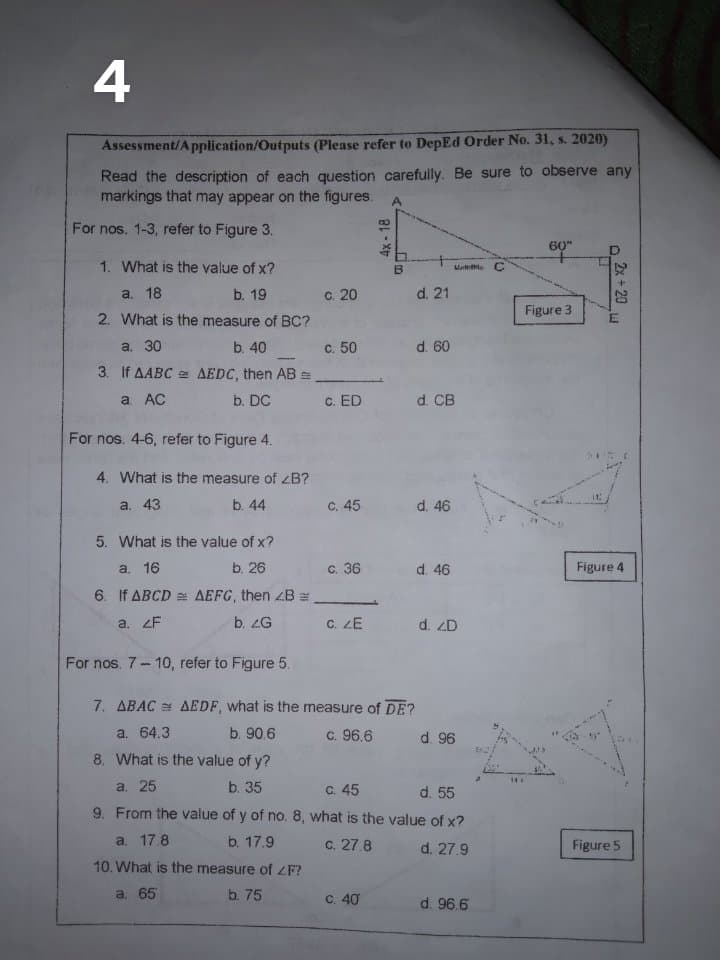
District A. Readings/Discussions Geometry without the study of congruent triangles is like reading a book and missing an important chapter of it. You are already familiar with some terms relating to triangles such as different parts, classifications, and even some basic properties of triangles in general, In this section, you are expected to determine the measures of the parts of congruent triangles. CPCTC is an acronym for Corresponding Parts of Congruent Triangles are congruent. It means that once two triangles are proven to be congruent, then the three pairs of sides that correspond must be congruent and the three pairs of angles that correspond must be congruent. Example 1. Name the pair of congruent sides and congruent angles of Figure 1. Congruent Sides Congruent Angles AB = DE LA = LD AC = DF CB = FE 4B = LE B A D E AABC = ADEF For Congruent Sides Given the same figure, solve for the value of x and y to show that the triangles are congruent. C x + 5 B y+ 10 E 35 AABC = ADEF From Figure 1, segment AC = segment OF, segment BC - EFand segment AB = DE, so we can substitute the given length,BC = EF Given AB = DE Given Substitute the given Substitute the given 25 = x +5 length of each y + 10 = 35 length of each 2 segment segment By subtraction 25 - 5 =x By subtraction y = 35 - 10 property of equality property of equality 20 = x Value of x y = 25 Value of y Check: BC = EF AB = DE 25 = x + 5 y + 10 = 35 25 = 20 + 5 25 + 10 = 35 25 = 25 35 = 35 Therefore, the value of x and y are 20 and 25 respectively For Congruent Angles . Example 2. Solve for the value of x. First, name the pair of congruent angles. (3x + 30) 55 65 S Figure 2 Second, solve for angle M. Note: That the sum of the interior angles of a triangle is 180 Solutions: ZN + 2L+ ZM = 180 55 + 65 + ZM = 180 120 + 4M = 180 ZM = 180 - 120 ZM = 60 Third, Solve for x ZM = CT Given 60 = 2x + 30 Substitute the given measure of each angle 60 -30 = 2x By subtraction property of equality 30 = 2x To extract 2, divide both sides by 2 15 = x Value of xCheck: LM = 2T 60 = 2x + 30 3 60 = 2 (15) + 30 60 = 30 + 30 60 = 60 Exercises: A. Name the congruent sides and congruent angle of the following pair of congruent triangles and identify the postulate theorem that can be used to conclude that the triangles are congruent SAS, SSS, ASA, 1 2. 3 D E Pairs of Pairs of Pairs of Pairs of Pairs of Pairs of Congruent Congruent Congruent Congruent Congruent Congruent Sides Angle Sides Angle Sides Angle B. Find the values of the variables based on the figure below. a. Find y *- 17 18 b. Find the measure of zC 45 c. Find x E d. Find the measure of LD e. Find the measure of ZF 2 a. Find z 75 b. Find x c. Find p d. Find the measure of z Y 60 e. Find the measure of 2S 41 p-764 Assessment/Application/Outputs (Please refer to DepEd Order No. 31, s. 2020) Read the description of each question carefully. Be sure to observe any markings that may appear on the figures. For nos. 1-3, refer to Figure 3. 4x - 18 D 1. What is the value of x? 07 + XZ a. 18 b. 19 c. 20 d. 21 Figure 3 2. What is the measure of BC? a. 30 b. 40 c. 50 d. 60 3. If AABC = AEDC, then AB = a AC b. DC C. ED d. CB For nos. 4-6, refer to Figure 4. 4. What is the measure of z B? a. 43 b. 44 C. 45 d. 46 5. What is the value of x? a. 16 b. 26 c. 36 d. 46 Figure 4 6. If ABCD = AEFG, then ZB = a.