Help needed for question 2c.

Problem setup

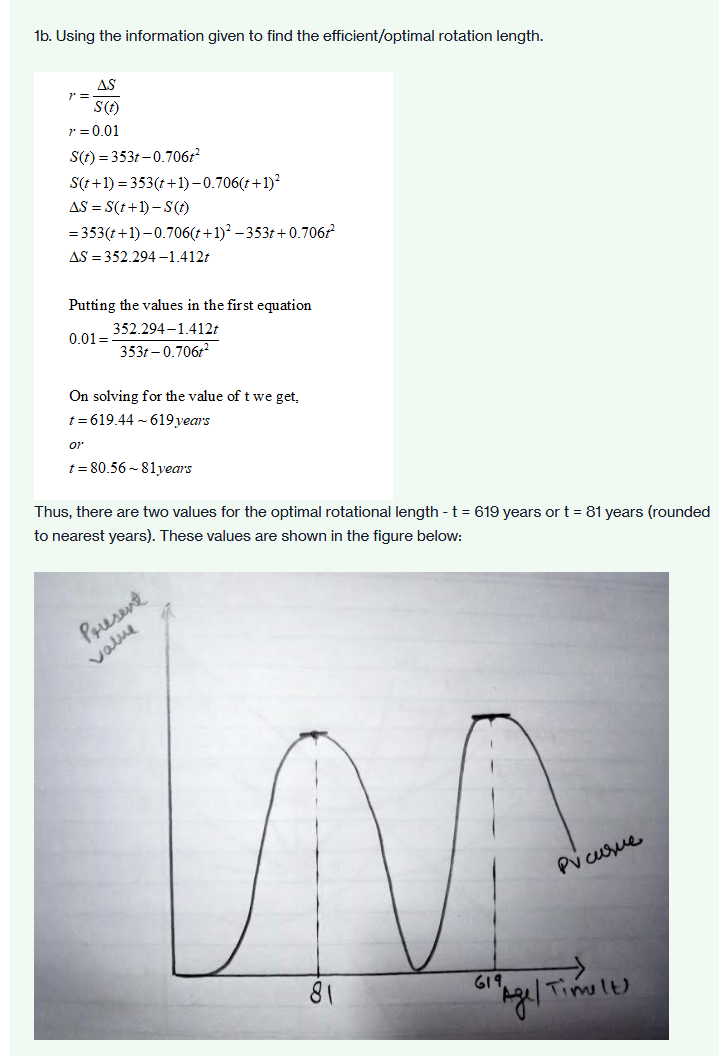
Question The owner of one acre of ponderosa pine [Pinus ponderosa] needs to plan a forest rotation length. Unless the owner chooses to sell the forest land, it will be kept in forestry and the chosen rotation will be maintained indenitely. Assume that the stumpage price is p = $2.53 per cubic feet and the fixed cost of harvest is C = $4,350.9D. The volume [in cubic feet} of the stand at any point in time is given by Wit} = at - bt'2 = 141.2t - 0.2824t 2 , where t is measured in years. Assume that the forest owner uses an annual discount rate of r = 0.01 and that the present value of bare land is S 2 $1?,912.46 per acre. 1.a. Write down a condition {ie., a mathematical relationship) the forest owner may use to choose a rotation length that maximizes the net present value of profits from timber harvest. In a few sentences, interpret the condition. 1.b What is the forest owner's efficient rotation length, rounded to the nearest year? Show your work. and plot your solution using a figure 1b. Using the information given to find the efficient/optimal rotation length. AS S (t) 7 =0.01 S(t) =353t -0.706t S(t+1) =353(t+1)-0.706(t+ 1)? AS = S(t+1)-S(1) =353(t+1) -0.706(t+1)2 -353 + 0.7067 AS = 352.294-1.412t Putting the values in the first equation 0.01 = 352.294-1.412t 353t - 0.706+2 On solving for the value of t we get, t=619.44 - 619 years or t =80.56 ~ 81 years Thus, there are two values for the optimal rotational length - t = 619 years or t = 81 years (rounded to nearest years). These values are shown in the figure below: Present value Pv curve 81 " Age / Timult )2.b. Given the forest owner's fire risk parameter is A = 0.02637, the above equation becomes: PQ (t) 6+2 [pQ(t)-c] (1-elo-) 2.50(a-2bt) 0.01+0.02637 [2.50(at-bt-)-4350.90] 2.50(141.2-2(0.2824)t) 0.03637 [2.50(141.2t-0.2824t-)-1432.98] 353-1.412t 0.03637 (1-20 03637ty 353t-0.706t--1432.98 1-2010363 76 (353 - 1.412t)(1-e0.0565/) = 0.03637(353t - 0.706t2-1432.98) (353 - 1.412t)(1-e0.03637t) = 12.83861t - 0.02567722+2-52.1175 353 -1.412t-3530.03637+1.412te0.036371= 12.83861t - 0.02567722t--52.1175 0.03637t(-351.588) =-0.02567722t-+14.25061t - 405.1175 351.588-0.03637t= 0.02567722t2-14.25061t + 405.1175 0.03637t= 0.0000730t--0.04053t + 1.15225[t = 30, 525] e 0.03637t= 30ore0.03637t= 525 TakingInonbothsides 0.03637tine = In30 t = 3.4011/0.03637 = 93.51 or 0.03637t= 525 0.03637t = In525 t = 6.263/0.03637 = 17.221 Since, average occurrence of fire is roughly 38 years, efficient rotation length given the risk of fire will be approximately 94 years2. Return to the set-up of problem #1. Now assume that the forest owner faces the risk of severe fire that requires replanting of the stand. Such a fire occurs on average once roughly every 38 years. Specifically, assume that the forest owner's fire risk parameter is A = 0.02637. This risk is reflected in the value of bare forest land in the area, which in this scenario is S = $2,917.92 per acre. Assume all other parameters from problem #1 remain the same. 2.a. Write down a condition the forest owner may use to choose a rotation length that maximizes the (expected) net present value of profits from timber harvest in this scenario. In a few sentences, interpret the condition. 2.b. What is the forest owner's efficient rotation length given the risk of fire, rounded to the nearest year? 2.c. Using a figure, compare your solution from problem #2.b with your solution from problem #1.b. In a few sentences, interpret your results