Help with my chapter 13 assignment please

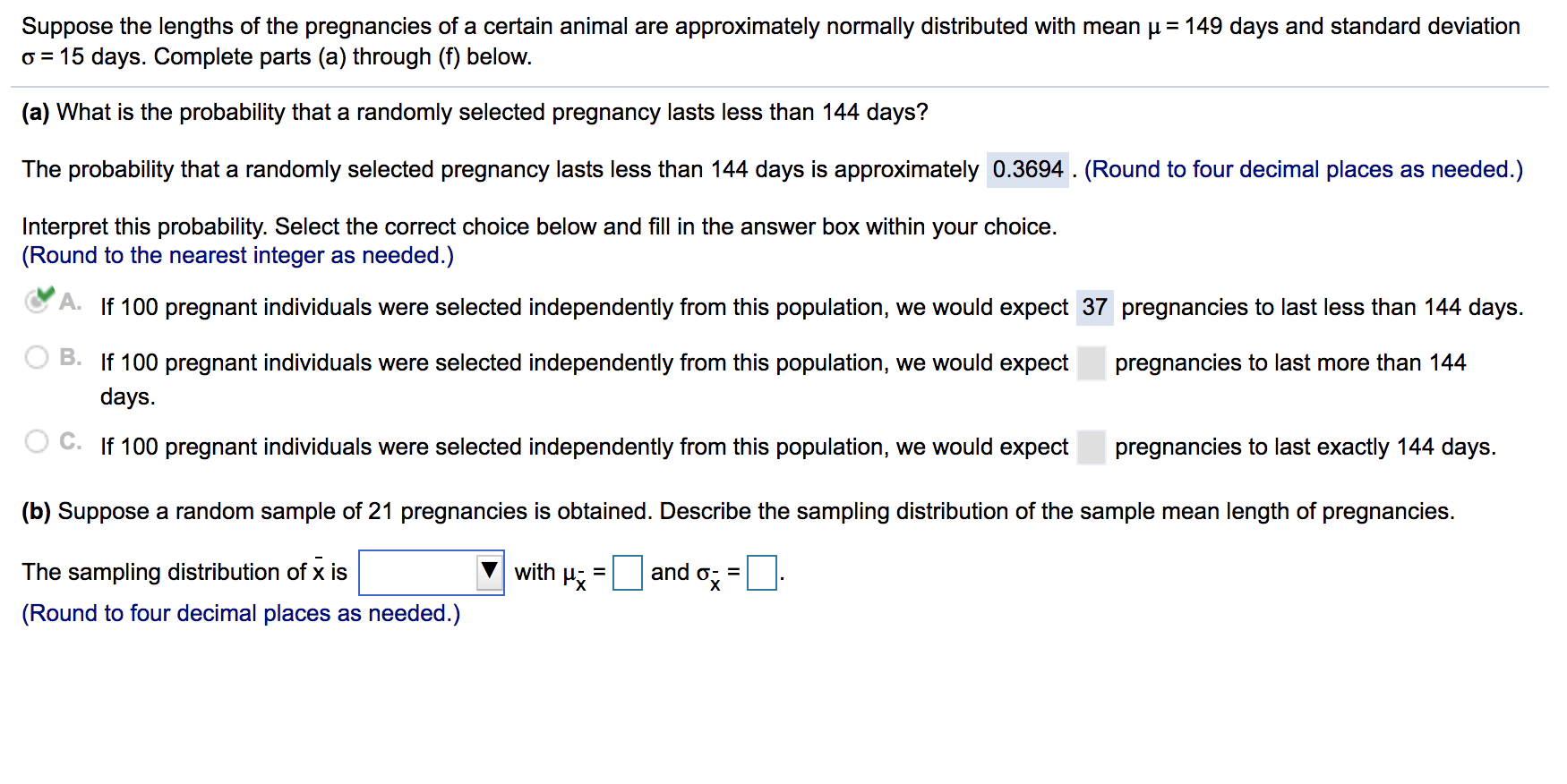
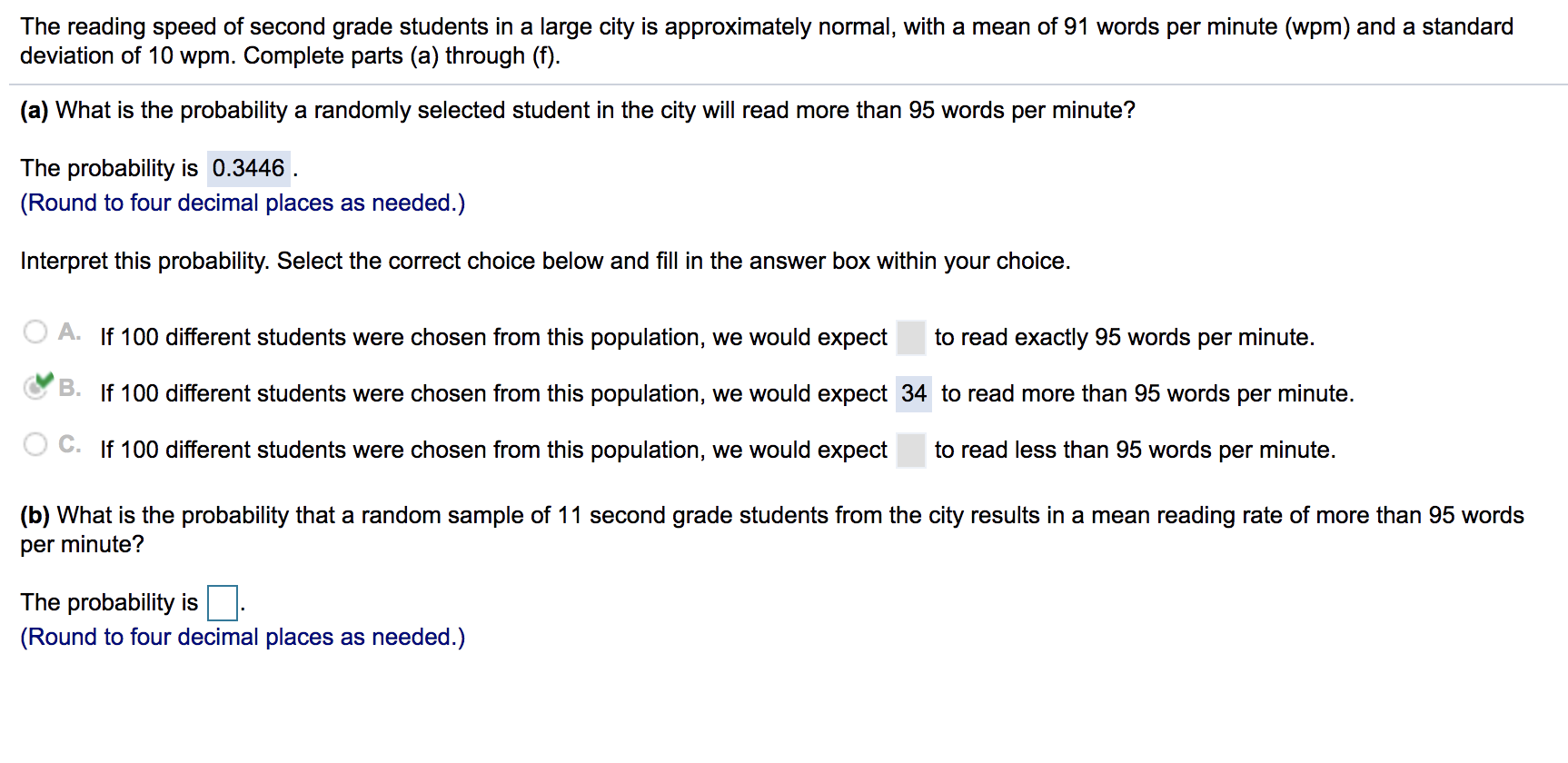
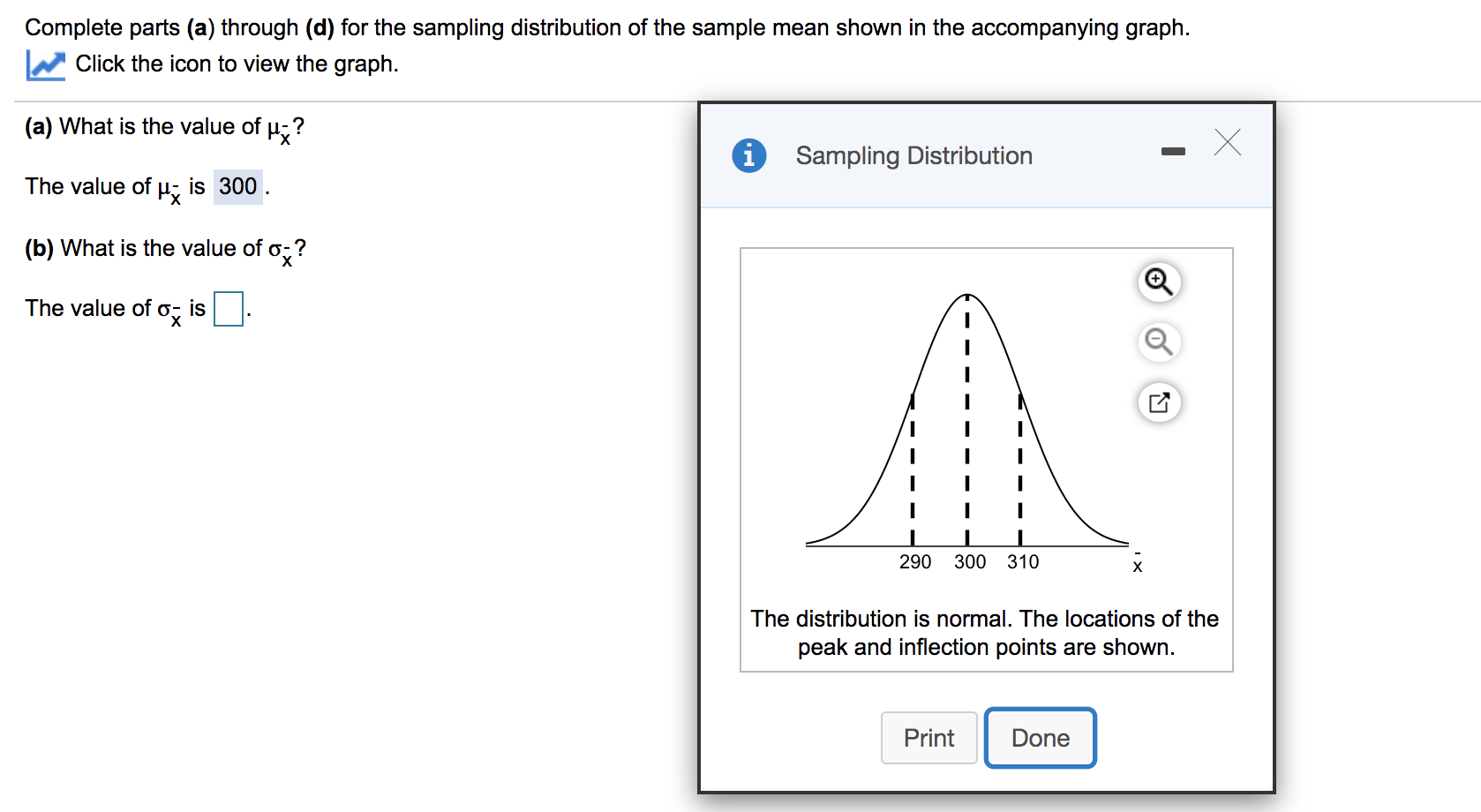
Suppose the lengths of the pregnancies of a certain animal are approximately normally distributed with mean u = 149 days and standard deviation 0 = 15 days. Complete parts (a) through (f) below. (a) What is the probability that a randomly selected pregnancy lasts less than 144 days? The probability that a randomly selected pregnancy lasts less than 144 days is approximately 0.3694 . (Round to four decimal places as needed.) Interpret this probability. Select the correct choice below and ll in the answer box within your choice. (Round to the nearest integer as needed.) "v A- If 100 pregnant individuals were selected independently from this population, we would expect 37 pregnancies to last less than 144 days. 3- If 100 pregnant individuals were selected independently from this population, we would expect pregnancies to last more than 144 days. (3- If 100 pregnant individuals were selected independently from this population, we would expect pregnancies to last exactly 144 days. (b) Suppose a random sample of 21 pregnancies is obtained. Describe the sampling distribution of the sample mean length of pregnancies. The sampling distribution ofx is E! with \"i = D and a)? = D. (Round to four decimal places as needed.) The reading speed of second grade students in a large city is approximately normal, with a mean of 91 words per minute (wpm) and a standard deviation of 10 wpm. Complete parts (a) through (f). (a) What is the probability a randomly selected student in the city will read more than 95 words per minute? The probability is 0.3446 . (Round to four decimal places as needed.) Interpret this probability. Select the correct choice below and ll in the answer box within your choice. A- If 100 different students were chosen from this population, we would expect to read exactly 95 words per minute. 'v 3- If 100 different students were chosen from this population, we would expect 34 to read more than 95 words per minute. C. If 100 different students were chosen from this population, we would expect to read less than 95 words per minute. (b) What is the probability that a random sample of 11 second grade students from the city results in a mean reading rate of more than 95 words per minute? The probability is D. (Round to four decimal places as needed.) Suppose a geyser has a mean time between eruptions of 74 minutes. Let the interval of time between the eruptions be normally distributed with standard deviation 21 minutes. Complete parts (a) through (e) below. (a) What is the probability that a randomly selected time interval between eruptions is longer than 84 minutes? The probability that a randomly selected time interval is longer than 84 minutes is approximately 0.3170 . (Round to four decimal places as needed.) (b) What is the probability that a random sample of 7 time intervals between eruptions has a mean longer than 84 minutes? The probability that the mean of a random sample of 7 time intervals is more than 84 minutes is approximately 0.1039'. (Round to four decimal places as needed.) (:2) What is the probability that a random sample of 25 time intervals between eruptions has a mean longer than 84 minutes? The probability that the mean of a random sample of 25 time intervals is more than 84 minutes is approximately '1. (Round to four decimal places as needed.) Complete parts (a) through (d) for the sampling distribution of the sample mean shown in the accompanying graph. K Click the icon to view the graph. (a) What is the value of \"i? 0 Sampling Distribution The value of \"i is 300 . (b) What is the value of \"i? The value of a; is El. 290 300 310 The distribution is normal. The locations of the peak and inection points are shown