Help with my project, please!

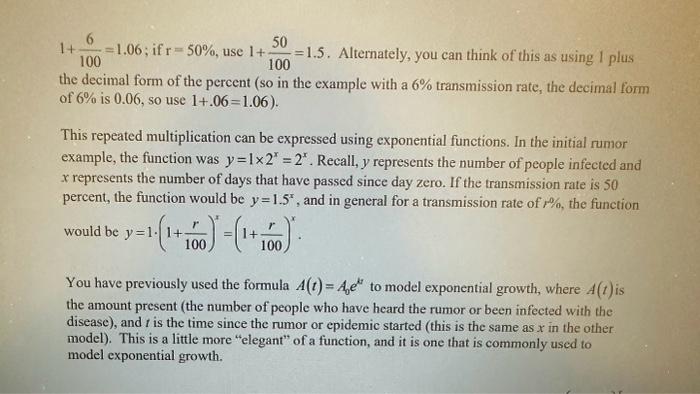
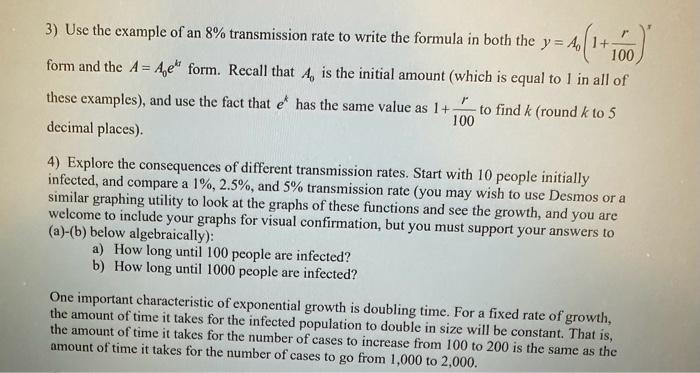
In this project, you will explore the behavior of exponential functions by using them to model the spread of disease. It is important to note that, while meaningfill in this context, the expociential and logistic growth models offer a very simplified view of a very complex phenomenon. You will be asked to consider some of the limitations of this model at the end of the project. 1) Consider how a rumor might spread among a population. Suppose on Day 1 a single person telis someone else a rumor, and suppose that on every subsequent day, each person who knows the rumor tells exactly one other person the rumor. a) How many days until 50 people have heard the rumor? b) How many days until 100 people have heard the rumor? In the situation with the rumor, the number of people who have heard the rumor doubles every day; this is because, each day, every person who knows the rumor tells it to a new person. In other words, there is a 100 percent transmission rate: 100 percent of those who know the rumor spread it to someone else. A transmission rate this high means that the number of people who know the rumor will grow very quickly. One way to explore the exponential model is through simple multiplication. In the rumor example, the number of people who know the rumor doubles every day, and so repeated multiplication by 2 is used to calculate how many people know the rumor. For example, afier four days, the total number of people who know the rumor has doubled four times, and so is 12222=16. This can be rewritten more concisely as 124. This pattern leads to a generalized formula, y=12x, where y represents the number of people who have heard the rumor on day x, and x is the number of days since the rumor started. 2) Use this generalired formula to calculate the following: a) How many days until the whole city of Roanoke (approximately 100,000 people) has heard the rumor? b) How many days until the whole country (approximately 330,000,000 people) has heard the runor? Mathematically, the spread of discase can be modeled in a manner similar to the spread of a rumor. Although a number of simplifying asstimptions must be made, the simple exponential model captures the basic impact of transmistion rates on the dispersion of a disense among a population. If the transmission rate were, say, 50 pereent instead of 100 percent, then repeated multiplication by 15 would be used. This would mean that, each day, 50% of those infected would infeet someone else. In general, if the rate of transmission as a percent is 2%, then the number used in repeated multiplication would be 1+100r. For example, if the transmission rate is 6 percent, use 1+1006=1.06; if r=50%, use 1+10050=1.5. Alternately, you can think of this as using 1 plus the decimal form of the percent (so in the example with a 6% transmission rate, the decimal form of 6% is 0.06 , so use 1+.06=1.06 ). This repeated multiplication can be expressed using exponential functions. In the initial rumor example, the function was y=12x=2x. Recall, y represents the number of people infected and x represents the number of days that have passed since day zero. If the transmission rate is 50 percent, the function would be y=1.5x, and in general for a transmission rate of r%, the function would be y=1(1+100r)x=(1+100r)x. You have previously used the formula A(t)=A0ett to model exponential growth, where A(t) is the amount present (the number of people who have heard the rumor or been infected with the disease), and t is the time since the rumor or epidemic started (this is the same as x in the other model). This is a little more "elegant" of a function, and it is one that is commonly used to model exponential growth. 3) Use the example of an 8% transmission rate to write the formula in both the y=A0(1+100r)x form and the A=A0ekt form. Recall that A0 is the initial amount (which is equal to J in all of these examples), and use the fact that ek has the same value as 1+100r to find k (round k to 5 decimal places). 4) Explore the consequences of different transmission rates. Start with 10 people initially infected, and compare a 1%,2.5%, and 5% transmission rate (you may wish to use Desmos or a similar graphing utility to look at the graphs of these functions and see the growth, and you are welcome to include your graphs for visual confirmation, but you must support your answers to (a)-(b) below algebraically): a) How long until 100 people are infected? b) How long until 1000 people are infected? One important characteristic of exponential growth is doubling time. For a fixed rate of growth, the amount of time it takes for the infected population to double in size will be constant. That is, the amount of time it takes for the number of cases to increase from 100 to 200 is the same as the amount of time it takes for the number of cases to go from 1,000 to 2,000 . In this project, you will explore the behavior of exponential functions by using them to model the spread of disease. It is important to note that, while meaningfill in this context, the expociential and logistic growth models offer a very simplified view of a very complex phenomenon. You will be asked to consider some of the limitations of this model at the end of the project. 1) Consider how a rumor might spread among a population. Suppose on Day 1 a single person telis someone else a rumor, and suppose that on every subsequent day, each person who knows the rumor tells exactly one other person the rumor. a) How many days until 50 people have heard the rumor? b) How many days until 100 people have heard the rumor? In the situation with the rumor, the number of people who have heard the rumor doubles every day; this is because, each day, every person who knows the rumor tells it to a new person. In other words, there is a 100 percent transmission rate: 100 percent of those who know the rumor spread it to someone else. A transmission rate this high means that the number of people who know the rumor will grow very quickly. One way to explore the exponential model is through simple multiplication. In the rumor example, the number of people who know the rumor doubles every day, and so repeated multiplication by 2 is used to calculate how many people know the rumor. For example, afier four days, the total number of people who know the rumor has doubled four times, and so is 12222=16. This can be rewritten more concisely as 124. This pattern leads to a generalized formula, y=12x, where y represents the number of people who have heard the rumor on day x, and x is the number of days since the rumor started. 2) Use this generalired formula to calculate the following: a) How many days until the whole city of Roanoke (approximately 100,000 people) has heard the rumor? b) How many days until the whole country (approximately 330,000,000 people) has heard the runor? Mathematically, the spread of discase can be modeled in a manner similar to the spread of a rumor. Although a number of simplifying asstimptions must be made, the simple exponential model captures the basic impact of transmistion rates on the dispersion of a disense among a population. If the transmission rate were, say, 50 pereent instead of 100 percent, then repeated multiplication by 15 would be used. This would mean that, each day, 50% of those infected would infeet someone else. In general, if the rate of transmission as a percent is 2%, then the number used in repeated multiplication would be 1+100r. For example, if the transmission rate is 6 percent, use 1+1006=1.06; if r=50%, use 1+10050=1.5. Alternately, you can think of this as using 1 plus the decimal form of the percent (so in the example with a 6% transmission rate, the decimal form of 6% is 0.06 , so use 1+.06=1.06 ). This repeated multiplication can be expressed using exponential functions. In the initial rumor example, the function was y=12x=2x. Recall, y represents the number of people infected and x represents the number of days that have passed since day zero. If the transmission rate is 50 percent, the function would be y=1.5x, and in general for a transmission rate of r%, the function would be y=1(1+100r)x=(1+100r)x. You have previously used the formula A(t)=A0ett to model exponential growth, where A(t) is the amount present (the number of people who have heard the rumor or been infected with the disease), and t is the time since the rumor or epidemic started (this is the same as x in the other model). This is a little more "elegant" of a function, and it is one that is commonly used to model exponential growth. 3) Use the example of an 8% transmission rate to write the formula in both the y=A0(1+100r)x form and the A=A0ekt form. Recall that A0 is the initial amount (which is equal to J in all of these examples), and use the fact that ek has the same value as 1+100r to find k (round k to 5 decimal places). 4) Explore the consequences of different transmission rates. Start with 10 people initially infected, and compare a 1%,2.5%, and 5% transmission rate (you may wish to use Desmos or a similar graphing utility to look at the graphs of these functions and see the growth, and you are welcome to include your graphs for visual confirmation, but you must support your answers to (a)-(b) below algebraically): a) How long until 100 people are infected? b) How long until 1000 people are infected? One important characteristic of exponential growth is doubling time. For a fixed rate of growth, the amount of time it takes for the infected population to double in size will be constant. That is, the amount of time it takes for the number of cases to increase from 100 to 200 is the same as the amount of time it takes for the number of cases to go from 1,000 to 2,000