Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Hi, can anyone solve this Lagrange and Kuhntucker question, the highlighted question 5, I have also given the solution for this. Can you solve the


Hi, can anyone solve this Lagrange and Kuhntucker question, the highlighted question 5, I have also given the solution for this. Can you solve the question in a simplified way. Thanks
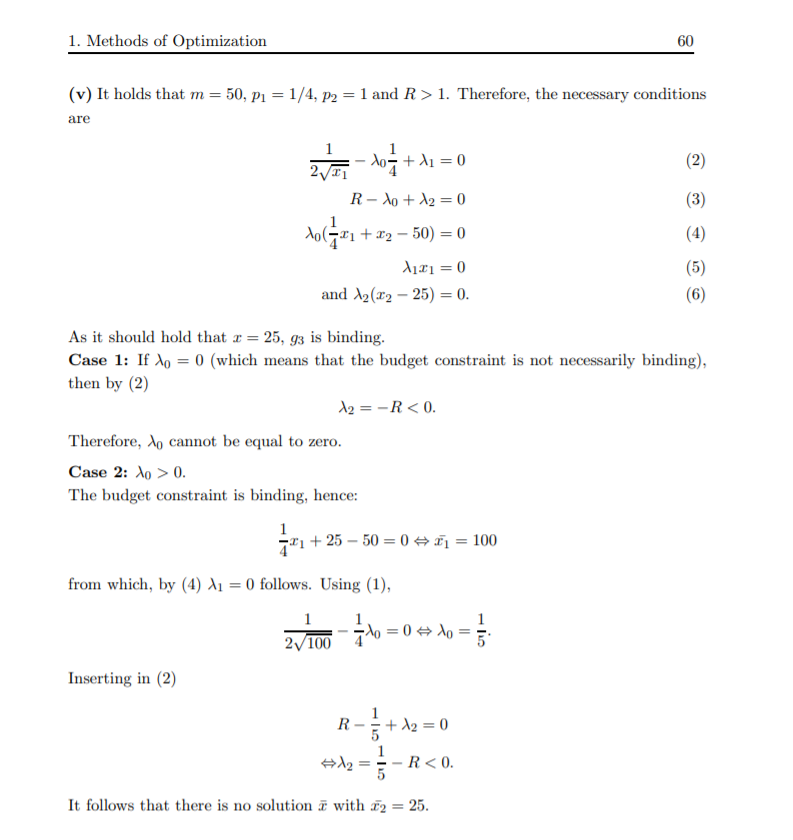
Exercise 25. Maria wants to spend part of her income on vacation (consumption of good 1) and save the rest (consumption of good 2). Her utility function is given by u(11, 12) := V11 + R 82, where R > 1 reflects the interest rate. Maria's income is denoted by m >0. The price of good 2 is normalized to p2 = 1. To be able to cover certain future costs, Maria has to consume a minimum amount of good 2 of 72 = 25. For good 1, there is only the usual non-negativity condition in place, i.e. 210. In the following, we analyze Marias utility maximization problem using the Kuhn-Tucker method. You may use that the utility function is monotonically increasing and (quasi-) concave. Furthermore, all constraints are convex. Hence, the necessary conditions are also sufficient. (1) Write down the Lagrangian function. Please denote the multipliers by 10, 11, 12. (ii) Write down the necessary Kuhn-Tucker conditions, that a local maximizer has to satisfy. (iii) Describe in keywords, how a global maximizer can be determined (NO calculation). (iv) How does the fact that the utility function is concave simplifies finding a maximizer? (1) Suppose m = 50, P1 = 1/4, P2 = 1 and R > 1 holds. Will Maria demand only the minimal amount of good 2? More precisely: Is with T2 = 25 a utility maximizer? Explain in keywords, how you proceed. (Hint: Think about, which constraints are binding and consider the Lagrangian multipliers in the first order conditions.) Solution of exercise 25. (i) The maximization problem is defined by max u(x1, x2) = VT1+ Rx2 (R > 1) s.t. P1.2 + P2.22Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


