Hi, I have a classwork to do, the classwork are the ones that have classwork written above the task.

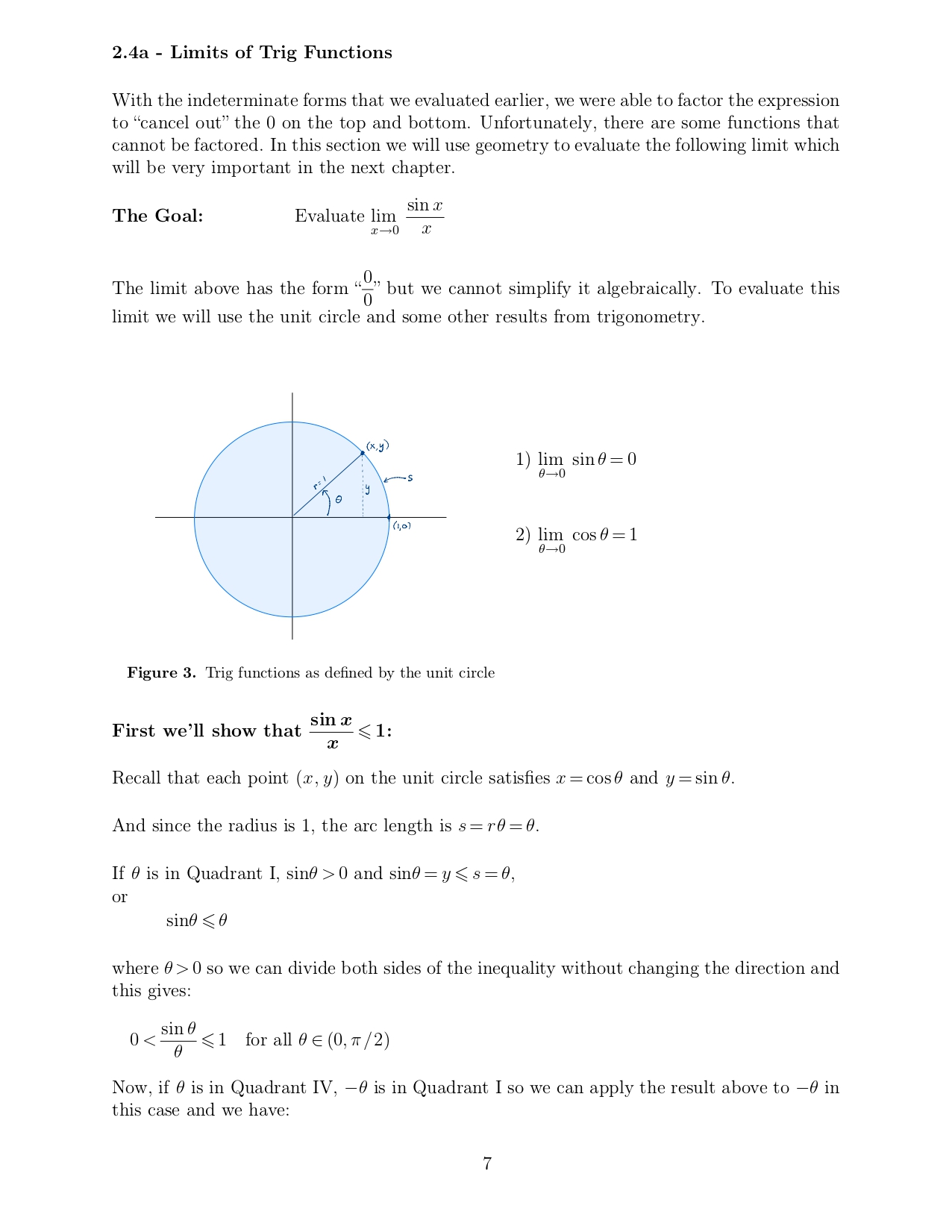
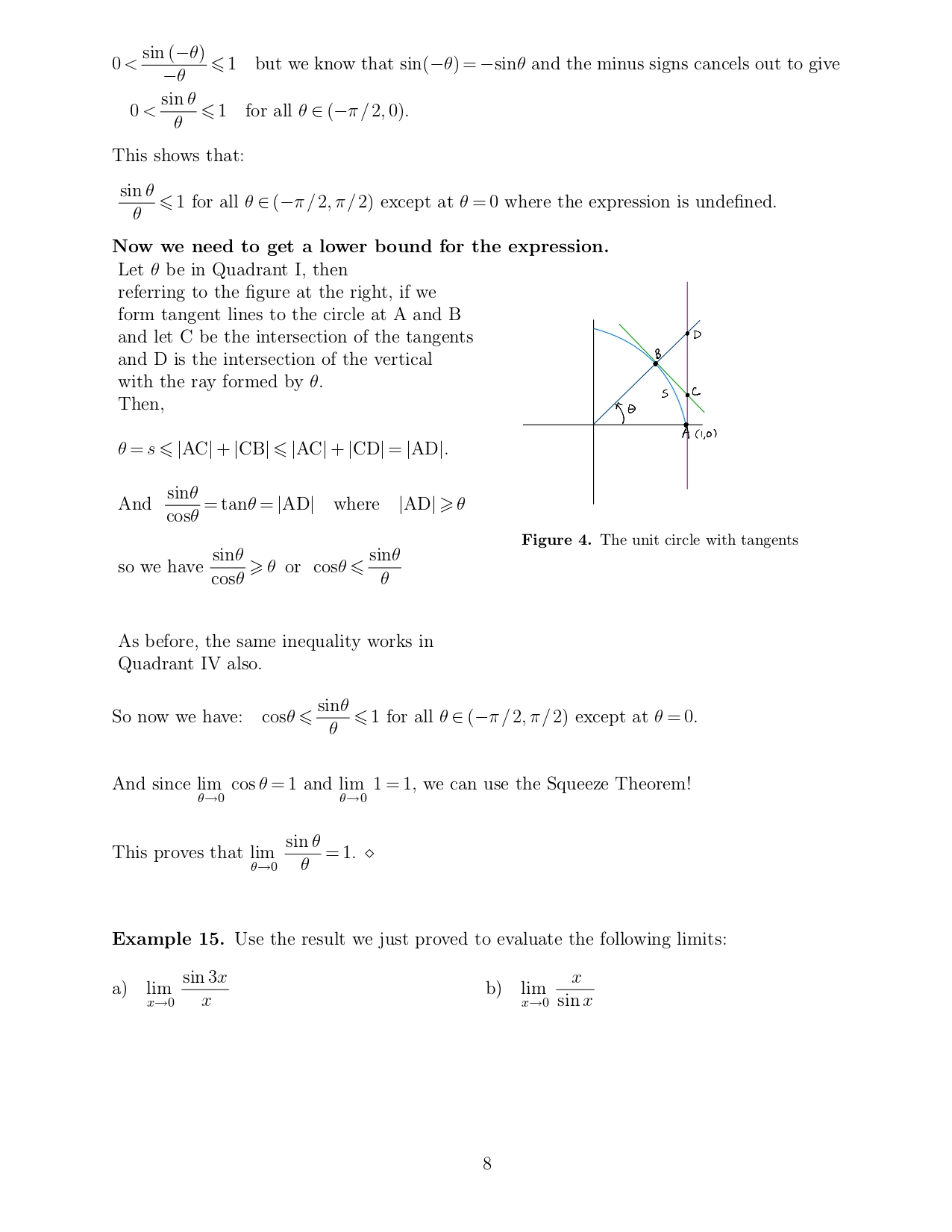
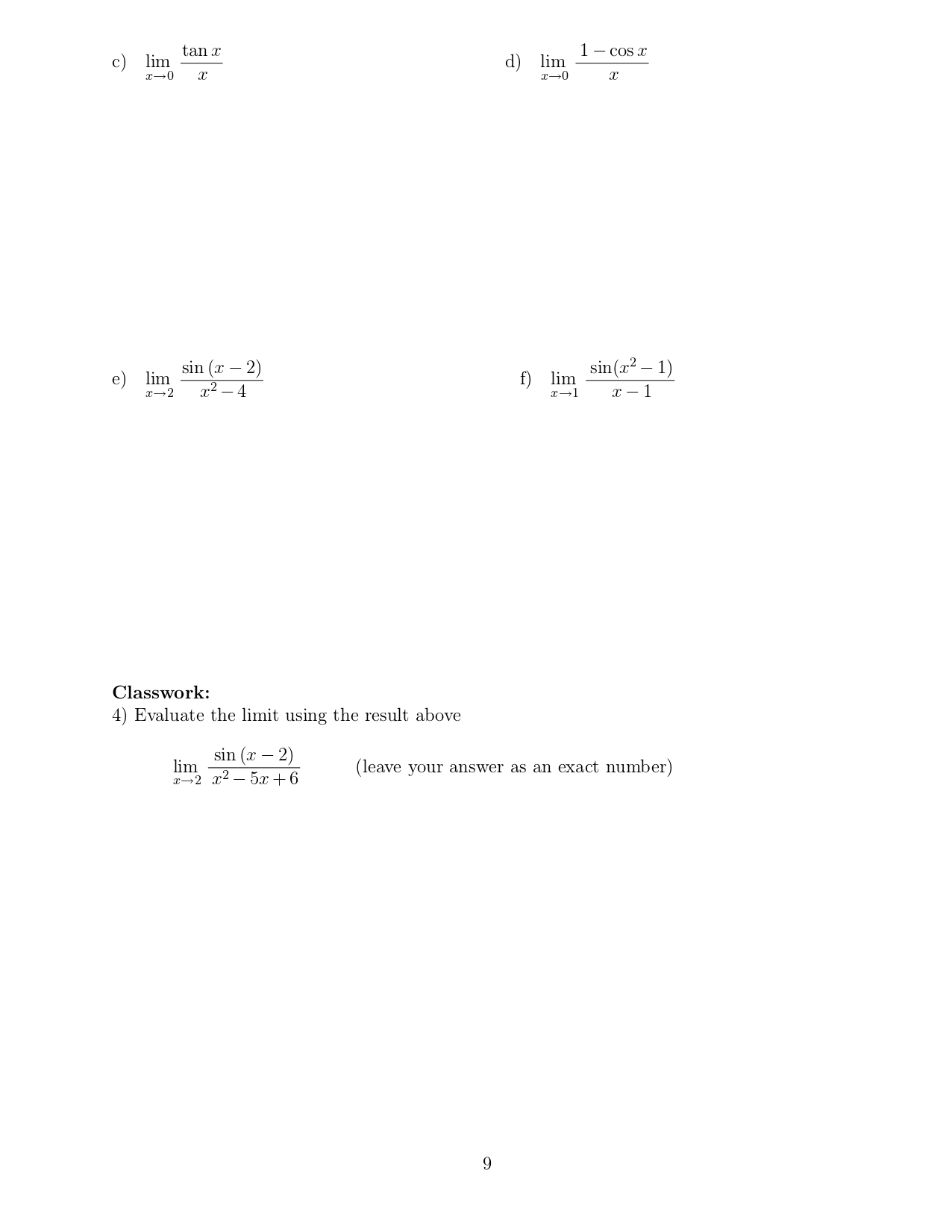
Math 5A - Lecture Notes for Monday, 8/30 Last week we used the graph of a function to determine limits at various values of a and we saw how to identify and calculate infinite limits. Example 1. Which of the statements below (if any) is true? a) x2 + x-6 -=x+3 b) lim xz+x-6 - =5 x - 2 x-2 x - 2 Example 2. Sketch the graph of f(x) = 1 2x +4 ifx7-1 ifx 0 if n is even Example 4. Evaluate lim x More Rules for Evaluating Limits Suppose that lim f(x) = L and lim g(x) = K, where L and K are both finite numbers, x-a" I-a then a) lim (f (x) + g(x)) = L + K b) limef(x) =cL for any constant c x-a Example 5. Combining i), ii), iii),a) and b) we can evaluate: lim x3 + 3x2 + 4x + 2=8 + 12 + 8+ 2=30 2- 2 2lim f(x)g(x) = L . K x-a Example 6. lim (x + 2) va+1=5V/4-5 .2=10 d) lim J (x) L if K # 0 c-ag(x) K Example 7. lim (2+ 2) 4 x-2(x -4) -2 -2 Example 8. lim (x - 2) 0 2-2 (x + 2) -4 = 0 Example 9. lim (2 + 2) _..4,, x-2(x -2) 0 and this limit does not exist. Notice that we do not have to evaluate an infinite limit here because the function would go to too on one side and to -co on the other side. We normally only write infinity as a limit when working with a single sided limit that is unbounded. Otherwise, we use the term does not exist for limits that are going to different infinities. lim (f(x))" = In Example 10. lim (cos ac)2 = (-1)2 =1 E) lim g( f (x)) = lim g(y) y - L' Example 11. lim (x + sinx)? = ( lim (x + sin a) )2 = (7 + 0)2-72 3Indeterminate Form of a Limit: . . . . . 0 We cannot use the llmlt laws for evaluatlng 11m1ts of the form: \"\" or \"00 00\". 0 If lim f(.') has one of these forms when the value of a is \"plugged in\. 1 2 (3)331: (2711721) . $3+8 d) $13112 $4- 16 e) lirn $3+x275m72 3322 332*4: 1' 4 Q 1132 Mila: Classwork: Show that each limit is an indeterminate form then rewrite the function so that the limit can be evaluated: 2 . 2: +3x4 1) gel[1,11 5921 3:279 21' M313 m3+$730 Some Theorems About Limits Theorem 1: If a E (b, c) and f(a:) g(:r) for all I E (b, :3) except possibly at 17:03 and if limf(a:) 2L and limg(a:)=K, then LgK. 370. xr. Figure 1. The limit preserves inequalities Theorem 2: The Squeeze Theorem If a E (b, c) and f(a:) g(:r) h(.'17) for all I E (b, :3) except possibly at 17:03 and if lim f(a:) :L and limh(:r:) :L, then limg(:r) :L mag. 34\"}. $420. Figure 2. The Squeeze or Sandwich Theorem Example 14. Use the Squeeze Theorem to show that: lim 3:200s (g) : 0 $42 Classwork: 3) Suppose that .r2 | 2m + 1 g f(:r) g 1'2 235' + 3. Use the Squeeze Theorem to determine the limit: lim1f(:r). 2.4a - Limits of Trig Functions With the indeterminate forms that we evaluated earlier, we were able to factor the expression to \"cancel ou \" the 0 on the top and bottom. Unfortunately, there are some functions that cannot be factored. In this section we will use geometry to evaluate the following limit which will be very important in the next chapter. sin a: The Goal: Evaluate lim 3210 {E O _11 0 limit we will use the unit circle and some other results from trigonometry. The limit above has the form \" but we cannot simplify it algebraically. To evaluate this (>253 . . 1) 11m 5111 I9 = 0 921] ("01 2] lim cos I? = 1 921] Figure 3. Trig functions as dened by the unit circle sin :12 First we'll show that g 1: 3'3 Recall that each point (at, 3;) on the unit circle satises a: : 0056' and y : sin 6'. And since the radius is 1, the arc length is s: r6:6'. If :9 is in Quadrant I, sinfil > O and sing : y g s : :9, or sin' S 6 where 6 > 0 so we can divide both sides of the inequality without changing the direction and this gives: sin 9 6 U 9:0 9 Example 15. Use the result we just proved to evaluate the following lin1its: . sin 32: . a: a] 11m b) lnn , :E>D {E ma 311153 C) lim tan x lim 1 - cos x x-0 d) e) lim sin (x - 2) f) lim sin (a2 - 1) 22 - 4 x-1 x- 1 Classwork: 4) Evaluate the limit using the result above lim - sin (x - 2) x-2 x2 - 5x+6 (leave your answer as an exact number) 9