Hi tutors can you guide and help me with this. The lesson is already in the picture use this as a guide thanks for helping

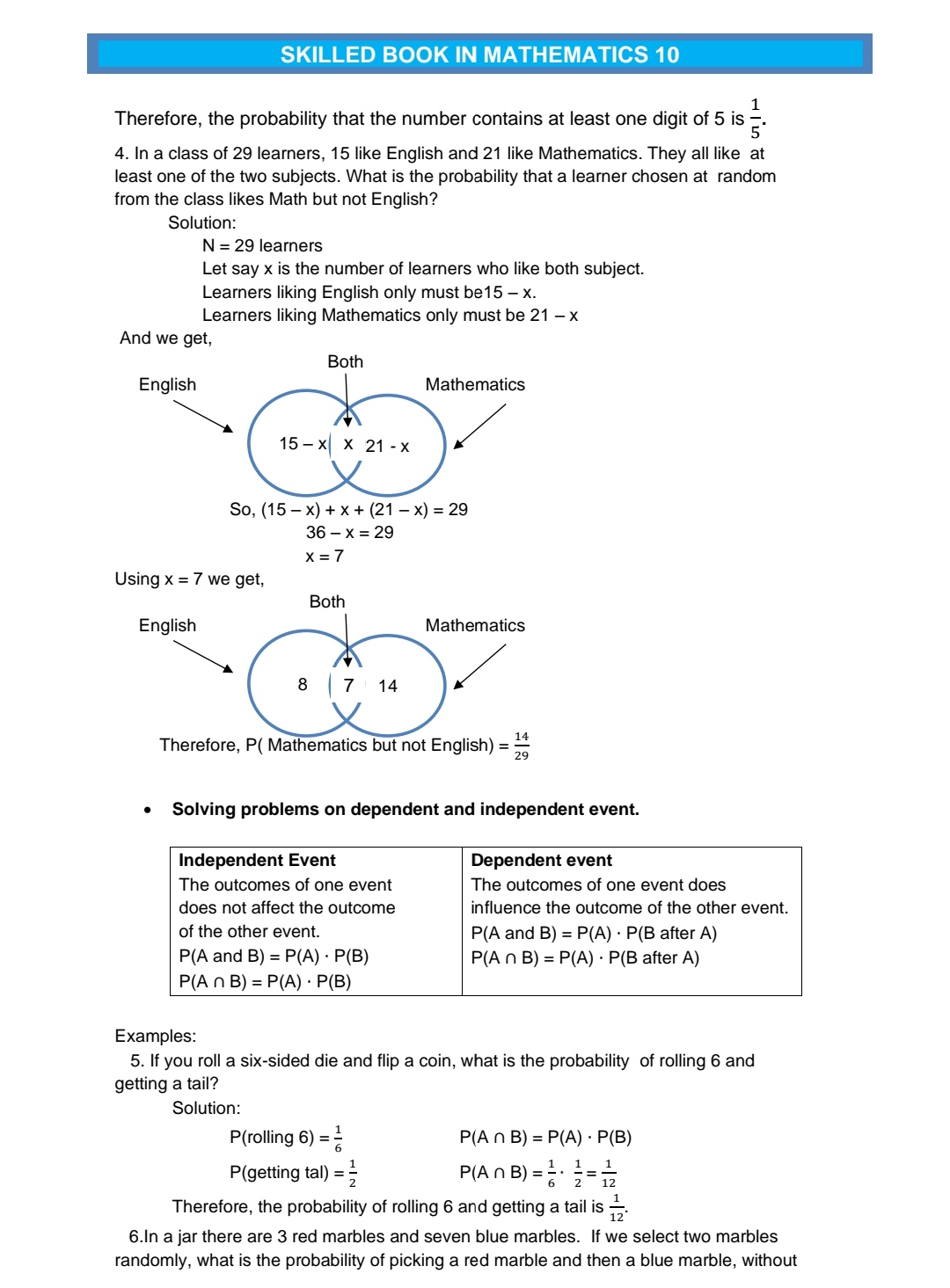
Illustrating Mutually Exclusive Events and Solve Week 8 Problems Involving Probability In this module, you are expected to illustrate mutually exclusive events and solve problems on probability. Mutually exclusive event is an event that cannot occur at the same time. Look at the illustration below: Mutually Exclusive Events OO P(A or B) = P(A) + P(B) In this illustration it shows that mutually exclusive events are those events which do not have anything in common. In other words, these events do not have an intersection. These are characterized by the word "or". It is the sum of individual probability written as, P(A or B) = P(A) + P(B) P(A U B) = P(A) + P(B) Examples: 1. Turning left and right (you cannot do this both at the same time. 2. Getting a head and tail in tossing a coin (you can't do this both at the same time. Let us illustrate the probability of mutually exclusive events. Examples: 1. The probability of getting an ace or a king from a deck of cards. Aces Kings The figure illustrates mutually exclusive events. Aces and Kings cannot occur K at the same time. In symbol. AV Ads KY P(Ace or King) = P(Ace) + P(King) K P(Ace U King) = P(Ace) + P(King) There are 52 cards, so N = 52 The event of getting an Ace is 4. Aces and Kings are The event of getting a King is 4. Mutually Exclusive (can't be both) 2. The probability of choosing online distance learning (ODL) and modular distance learning (MDL) among Grade 10 students in Dasmarinas West National High School.SKILLED BOOK IN MATHEMATICS 10 The gure illustrates mutually exclusive events. ODL and MDL cannot occur at the same time. In symbol. P(ODL or MDL) = P(ODL) + P(MDL) P(ODL U MDL) = P(ODL) + P(MDL) There 973 students. so N = 973 The event of online learners is 109. The event of modular learners is 864. After illustrating mutually exclusive event, let us proceed in solving problems involving probability. - Solve problems Involving mutually exclusive events. Use the formula: P(A u B) = P(A) + P(B) Examples: 1. What is the probability of getting a head or a tail in tossing a coin? Solution: The total number of outcomes: N = (head. tail) =2 The probability of getting a head: P(head) =21, The probability of getting a tail: P(tail) =3 Using the formula: P(head or tail): P(A) + P(B) P(A n B) HAUB)=%+%=1 Therefore, the probability of getting a head or a tail in tossing a coin is 1 . 2. The probability of picking a face card or an Ace from a deck of 52 playing cards. Solution: The total number of outcomes: N = 52 The probability of picking a face card: P(face card) = E: 2: = 133 The probability of picking an Ace: P(Ace) = g = = % Using the formula: P(face card or an ace): P(A) + P(B) P(Au3)=i+i=i 13 13 13 Therefore, the probability of getting a face card or an ace is 143. o In solving non-mutually exclusive events use the formula: P(A u B) = P(A) + P(B) P(A n B) 3. A number is chosen at random from a set of two-digit numbers from 10 to 99 inclusive. What is the probability that the number contains at least one digit 5? Solution: From 10 - 99 the total number of outcomes: N = 90 HA) = {15. 25. 35, 45. 55, 65, 75. 85. 95} HM==$ P(B) = (50. 51, 52, 53. 54, 55. 56, 57, 58, 59} 1 P(A) ==3 P(A and B) =% Using the formula: P(A u B) = P(A) + P(B) P(A n B) 9 10 1 18 = + - = = 90 90 90 90 U'lIH SKILLED BOOK IN MATHEMATICS 10 1 Therefore, the probability that the number contains at least one digit of 5 is 5' 4. In a class of 29 learners, 15 like English and 21 like Mathematics. They all like at least one of the two subjects. What is the probability that a leamer chosen at random from the class likes Math but not English? Solution: N = 29 learners Let say it is the number of learners who like both subiect. Learners liking English only must be15 x. Learners liking Mathematics only must be 21 x And we get, Both English Mathematics \\ ./ So,(15x)+x+(21x)=29 36x=29 X=7 Using it = 7 we get, - Solving problems on dependent and independent event. Independent Event Dependent event The outcomes of one event The outcomes of one event does does not affect the outcome inuence the outcome of the other event. of the other event. P(A and B) = P(A) - P(B after A) P(A and B) = P(A) - P(B) P(A n B) = P(A) - P(B after A) P(A n B): P(A) - P(B) Examples: 5. if you roll a six-sided die and ip a coin, what is the probability of rolling 6 and getting a tail? Solution: P(rolling 6) = P(A n B) = P(A) - P(B) P(getting tal)=% pm\" 3):? %=% Therefore, the probability of rolling 6 and getting a tail is i. 6.ln a iar there are 3 red marbles and seven blue marbles. If we select two marbles randomly, what is the probability of picking a red marble and then a blue marble, without replacement? P(red) = 110 P(blue) =5 P(A n B): P(A} - P(B) P(AnEl)=13- 3:3 7 ea _ 5 Therefore, the probability of picking a red marble and then a blue marble, without replacement A. Tell whether the following event is a mutually exclusive or NOT. Write an answer on the space provided. 1. Moving backwards and towards. 2. Getting an Ace or a Spade from a deck of cards. 3. Getting a face card or a club from a deck of cards. 4. Going to East and West. 5. Dancing and singing. B. Illustrate the probability of mutually exclusive event. 1. The probability of getting odd numbers or even numbers in casting a die. 2. The probability of picking a red card or spade from a deck of cards. 3. A ticket numbered 1 to 20 was placed in a box. illustrate the probability of choosing a factor of 8 and multiple of 5. 4.The probability of getting an\" I\" or a \"P\" in the word PHILIPPINES? 5. In a box, there are 5 red balls, 4 green bail, and 6 blue balls. Illustrate the probability of picking a red ball or a green ball? C. Solve problems involving mutually and not mutually exclusive events. 1. Two pair of dice are thrown. What is the probability that the score of the first die is 6 or the score of the second die is 5? 2. A basket contains 8 mangoes, 6 star apples. and 10 dragon fruits. What is the probability of picking mangoes or dragon fruit? 3. An octahedron die has 8 equal faces marked with numbers 1 to 8. If the die is thrown once, what is the probability that it lands a prime number or composite number? 4. What is the probability of randomly picking a number from 1 to 10, that is even or randomly picking a number from 1 to 100, that is odd or even. 5. In a group of 25 boys, 20 play basketball and 17 play volleyball. They all play at least one of the games. What is the probability that a boy chosen plays basketball but not volleyball? D. Solve problems involving independent and dependent event. 1. A box contains 5 green balls, 6 white balls, and 9 red balls. Two balls are drawn at random with replacement. Find the probability of getting red ball on the first draw, then white on the second. 2. There are 6 oranges, 5 apples and 5 mangoes on the basket. Paige got two fruits at random without replacement. Find the probability that it was an orange and apple. 3. Markson has 4 black shirts, 3 blue shirts and 2 red shirts in the cabinet. He took two 25 SKILLED BOOK IN MATHEMATICS 10 shirts randomly without replacement. Find the probability of getting two black shins. 4. Two pair of dice are thrown, one colored red and one colored blue. Find the probability that the score of red die is 1 and blue die is even. 5. A card is pulled from a deck of cards and noted. Then the card replaced and shuffled. The second card is pulled and noted. What is the probability that both cards are red