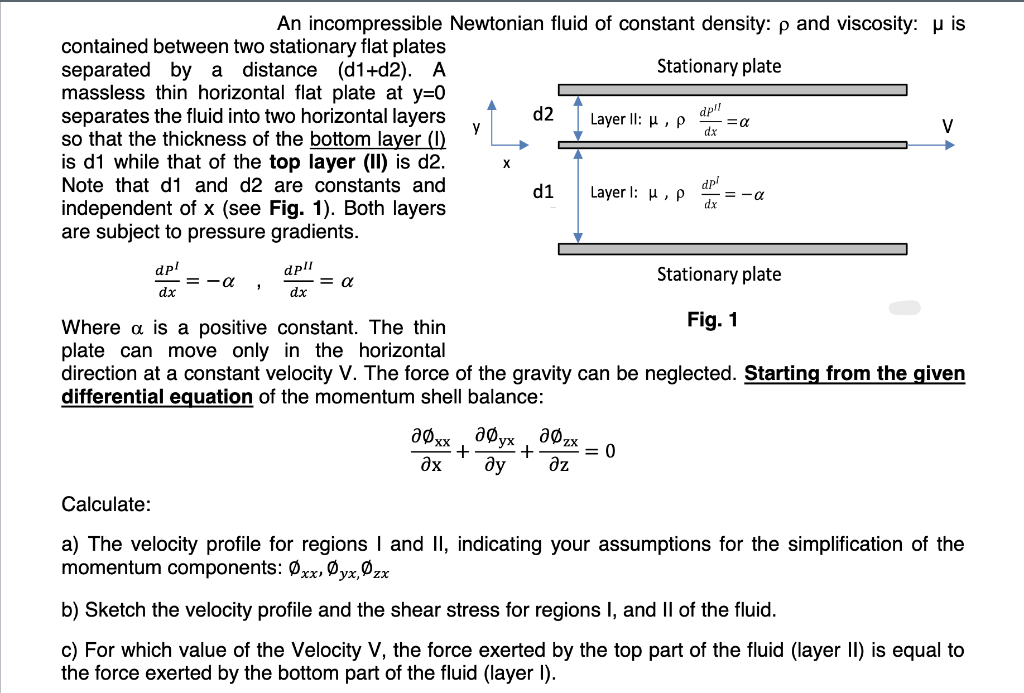
How do we go about this?

An incompressible Newtonian fluid of constant density: and viscosity: is contained between two stationary flat plates separated by a distance (d1+d2). A massless thin horizontal flat plate at y=0 separates the fluid into two horizontal layers so that the thickness of the bottom layer (I) is d1 while that of the top layer (II) is d2. Note that d1 and d2 are constants and independent of x (see Fig. 1). Both layers are subject to pressure gradients. dxdPI=,dxdPII= Where is a positive constant. The thin Fig. 1 plate can move only in the horizontal direction at a constant velocity V. The force of the gravity can be neglected. Starting from the given differential equation of the momentum shell balance: xxx+yyx+zzx=0 Calculate: a) The velocity profile for regions I and II, indicating your assumptions for the simplification of the momentum components: xx,yx,zx b) Sketch the velocity profile and the shear stress for regions I, and II of the fluid. c) For which value of the Velocity V, the force exerted by the top part of the fluid (layer II) is equal to the force exerted by the bottom part of the fluid (layer I). An incompressible Newtonian fluid of constant density: and viscosity: is contained between two stationary flat plates separated by a distance (d1+d2). A massless thin horizontal flat plate at y=0 separates the fluid into two horizontal layers so that the thickness of the bottom layer (I) is d1 while that of the top layer (II) is d2. Note that d1 and d2 are constants and independent of x (see Fig. 1). Both layers are subject to pressure gradients. dxdPI=,dxdPII= Where is a positive constant. The thin Fig. 1 plate can move only in the horizontal direction at a constant velocity V. The force of the gravity can be neglected. Starting from the given differential equation of the momentum shell balance: xxx+yyx+zzx=0 Calculate: a) The velocity profile for regions I and II, indicating your assumptions for the simplification of the momentum components: xx,yx,zx b) Sketch the velocity profile and the shear stress for regions I, and II of the fluid. c) For which value of the Velocity V, the force exerted by the top part of the fluid (layer II) is equal to the force exerted by the bottom part of the fluid (layer I)