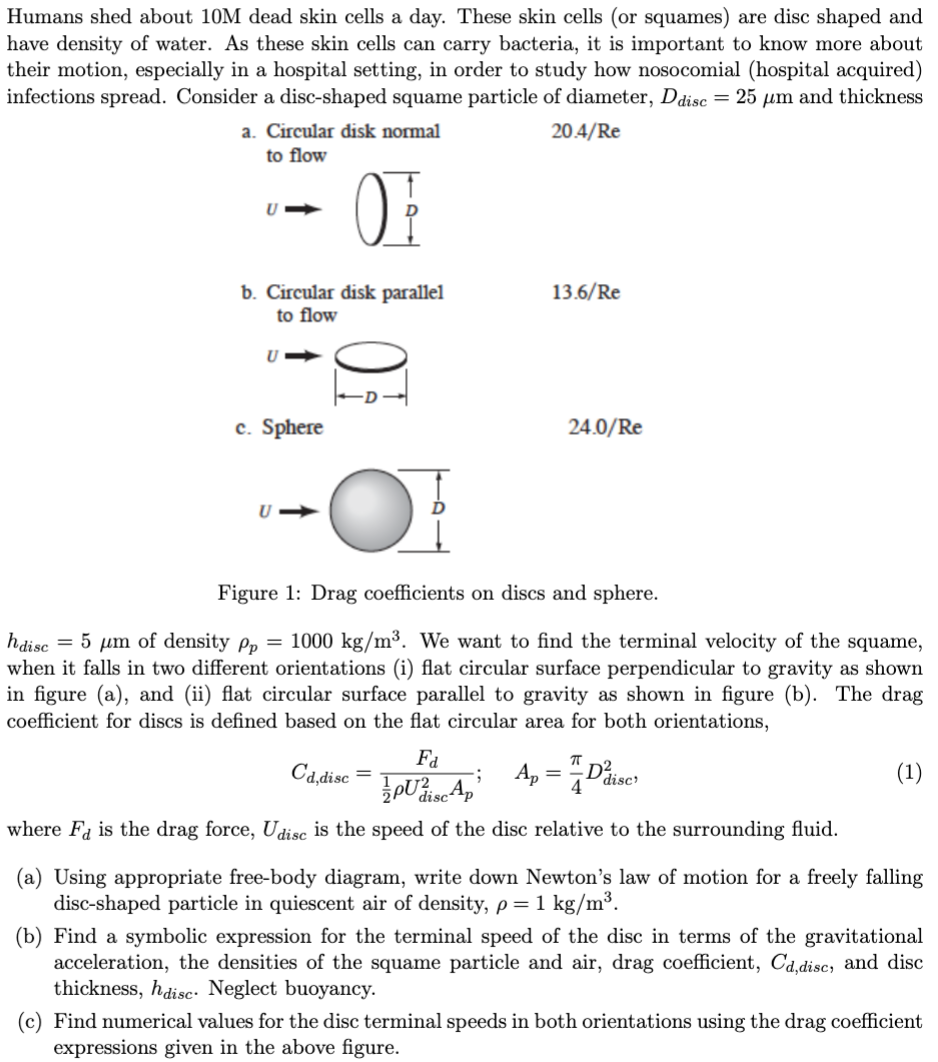
Humans shed about 10M dead skin cells a day. These skin cells (or squames) are disc shaped and have density of water. As these skin cells can carry bacteria, it is important to know more about their motion, especially in a hospital setting, in order to study how nosocomial (hospital acquired) infections spread. Consider a disc-shaped squame particle of diameter, Ddisc = 25 m and thickness a. Circular disk normal to flow 20.4/Re b. Circular disk parallel to flow 13.6/Re U c. Sphere 24.0/Re Figure 1: Drag coefficients on discs and sphere. hdisc = 5 m of density pp = 1000 kg/m. We want to find the terminal velocity of the squame, when it falls in two different orientations (i) flat circular surface perpendicular to gravity as shown in figure (a), and (ii) flat circular surface parallel to gravity as shown in figure (b). The drag coefficient for discs is defined based on the flat circular area for both orientations, Fd Cd,disc = disc ; Ap= Ap = 4 D disc where Fd is the drag force, Udisc is the speed of the disc relative to the surrounding fluid. (1) (a) Using appropriate free-body diagram, write down Newton's law of motion for a freely falling disc-shaped particle in quiescent air of density, p = 1 kg/m. (b) Find a symbolic expression for the terminal speed of the disc in terms of the gravitational acceleration, the densities of the squame particle and air, drag coefficient, Cd,disc, and disc thickness, hdisc. Neglect buoyancy. (c) Find numerical values for the disc terminal speeds in both orientations using the drag coefficient expressions given in the above figure. (d) Find a symbolic expression for the terminal velocity of a spherical particle (of same density as squame) and drag coefficient, Cd,sphere, based on the frontal area of sphere of diameter Dsphere. Find numerical values for the spherical particle diameter that will result in the same terminal speed as that of the disc in, i. orientation (a). ii. orientation (b).