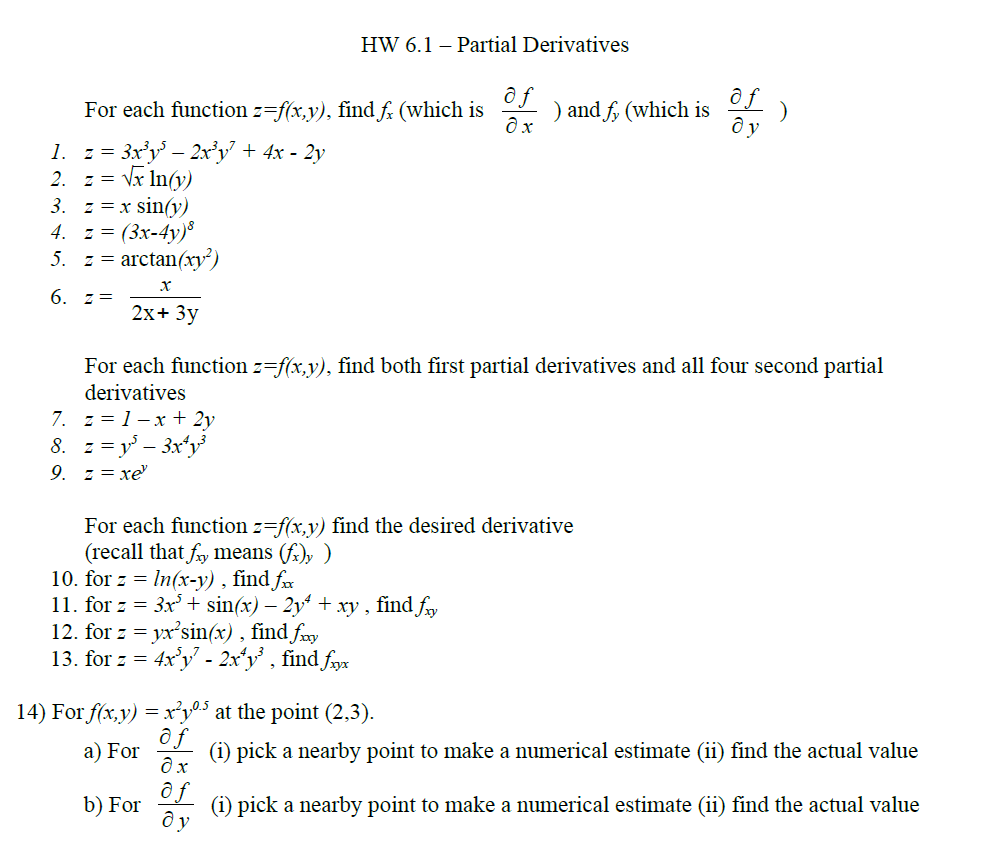
HW 6.1 - Partial Derivatives

For each function z=f(x,y), find fx (which is ? f

? x ) and fy (which is ? f

? y

)

1. z = 3x3y5 - 2x3y7 + 4x - 2y

2. z = ?x ln(y)

3. z = x sin(y)

4. z = (3x-4y)8

5. z = arctan(xy2)

6. z = x

2x+ 3y

For each function z=f(x,y), find both first partial derivatives and all four second partial

derivatives

7. z = 1 - x + 2y

8. z = y5 - 3x4y3

9. z = xey

For each function z=f(x,y) find the desired derivative

(recall that fxy means (fx)y )

10. for z = ln(x-y) , find fxx

11. for z = 3x5 + sin(x) - 2y4 + xy , find fxy

12. for z = yx2sin(x) , find fxxy

13. for z = 4x5y7 - 2x4y3 , find fxyx

14) For f(x,y) = x2y0.5 at the point (2,3).

a) For ? f

? x (i) pick a nearby point to make a numerical estimate (ii) find the actual value

b) For ? f

? y

(i) pick a nearby point to make a numerical estimate (ii) find the actual value

HW 6.1 - Partial Derivatives For each function z=f(x,y), find fx (which is of ) and fy (which is of ) ax dy 1. = = 3xy' - 2xBy' + 4x - 2y 2. = = Vx In(y) 3. z = x sin(y) 4. z = (3x-4)8 5. z = arctan(xy') X 6. 7= 2x + 3y For each function z=f(x,y), find both first partial derivatives and all four second partial derivatives 7. = = 1-x+2y 8. z=y - 3x4y 9. z = xe" For each function z=f(x,y) find the desired derivative (recall that fry means (fx)y ) 10. for = = In(x-y) , find fox 11. for = = 3x + sin(x) - 2y* + xy , find fry 12. for = = yx sin(x) , find fay 13. for z = 4x y - 2x y , find fryx 14) For f(x,y) = xy's at the point (2,3). a) For of a x (i) pick a nearby point to make a numerical estimate (ii) find the actual value b) For of (i) pick a nearby point to make a numerical estimate (ii) find the actual value