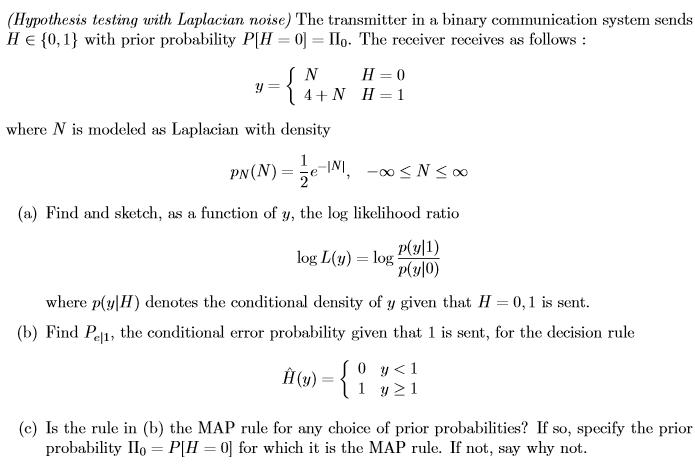
•  

(Hypothesis testing with Laplacian noise) The transmitter in a binary communication system sends H = {0,1} with prior probability P[H=0] = II. The receiver receives as follows: [ N ={ y = H=0 4+N H 1 where N is modeled as Laplacian with density PN(N) = 1 INI, - N e (a) Find and sketch, as a function of y, the log likelihood ratio log L(y) log = P(y|1) p(y|0) where p(y|H) denotes the conditional density of y given that H = 0,1 is sent. (b) Find Pe1, the conditional error probability given that 1 is sent, for the decision rule (y) = {{ 0 y < 1 1 y 1 (c) Is the rule in (b) the MAP rule for any choice of prior probabilities? If so, specify the prior probability II P[H=0] for which it is the MAP rule. If not, say why not. =