I am stuck on question 1F I am a economics major and this is an advanced macroeconomics course

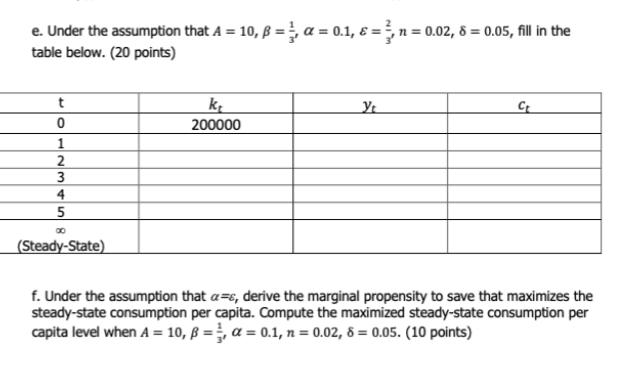
I. A Solow Growth Model with Heterogenous Income Sources (65 Points) Let us consider a Solow economy endowed at every time t with an aggregate population of size Lt growing at a constant rate ne (0,+20) per period: Lt+1 = (1+ n)Lt As everyone is employed, L. also corresponds to the size of the aggregate labour input which is used along with the aggregate stock of physical capital denoted by K; to produce Yt unit(s) of the aggregate output/income according to the following aggregate production function: Yt = AK-BLA where A>0, BE (0,1) are parameters. Production is carried out by a single profit-maximizing firm renting at every time & both physical capital and labour at the rental rates Wt, It respectively. In equilibrium at time & Wt = MPLt, It = MPK, where MPL, and MPKt denote the marginal product of labour and the marginal product of physical capital, respectively. The change in the physical capital from time & to time t+1 is governed by the following law of motion equation: Kt+1 - Ke = It - 8Kt where de (0,1) represents the physical capital depreciation rate parameter and /, denotes the aggregate investment in physical capital at time t which is equal in equilibrium to the aggregate saving S, which can be written as: St = aw,Let + er. K. where wtLt denotes the aggregate labour income, 7:K, represents the aggregate capital income and a,SE (0,1) are marginal propensity to save parameters. Aggregate consumption: Ct at time & is equal to the difference between aggregate output/income and aggregate saving: Ct = Yt - St Let yr denote the output/income per capita at time t: Yt yt= Let k: stand for the physical capital per capita at time & Kt kt =Ne a. Write-down the production function in per capita units. (5 points) b. Show that both the aggregate labour income Wt L, and the aggregate physical capital income r:Kt are fractions of the aggregate income Yr. (10 points) c. Show that in equilibrium, the physical capital per capita at time t+1: kt+1 can be written as a function of its lagged value: kt. (5 points) d. Derive the steady-state physical capital per capita: kss, the steady-state output/income per capita: yes, and the steady-state consumption per capita: Css. (15 points) e. Under the assumption that A = 10, B = ;, a = 0.1, & = =, n = 0.02, 8 = 0.05, fill in the table below. (20 points)e. Under the assumption that A = 10, 8 = _, a = 0.1, & = =, n = 0.02, 6 = 0.05, fill in the table below. (20 points) Ft yt Ct O 200000 AWNH 5 (Steady-State) f. Under the assumption that a=6, derive the marginal propensity to save that maximizes the steady-state consumption per capita. Compute the maximized steady-state consumption per capita level when A = 10, 8 = ;, a = 0.1, n = 0.02, 6 = 0.05. (10 points)