I don't know how to calculate the answer to all blank. Picture 2 is the reference.

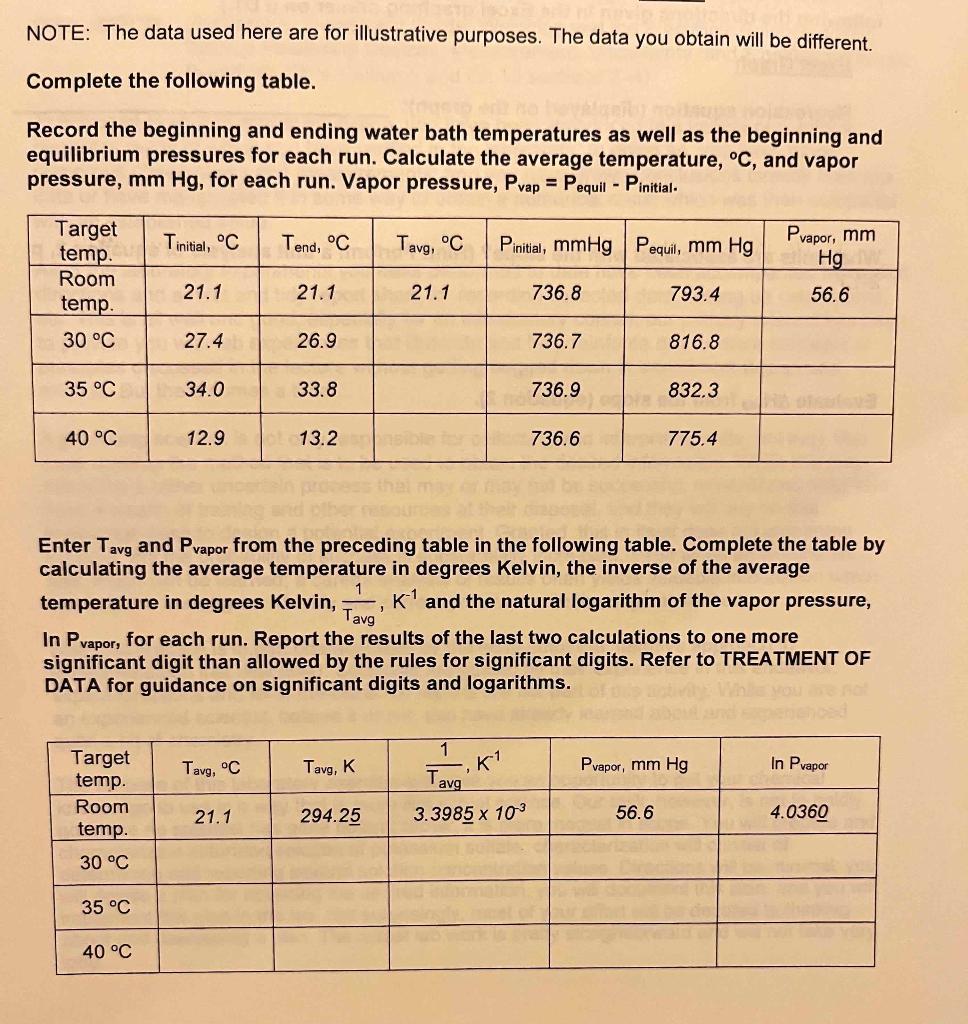
NOTE: The data used here are for illustrative purposes. The data you obtain will be different. Complete the following table. Record the beginning and ending water bath temperatures as well as the beginning and equilibrium pressures for each run. Calculate the average temperature, C, and vapor pressure, mmHg, for each run. Vapor pressure, Pvap=PequilPinitial.. Enter Tavg and Pvapor from the preceding table in the following table. Complete the table by calculating the average temperature in degrees Kelvin, the inverse of the average temperature in degrees Kelvin, Tavg1,K1 and the natural logarithm of the vapor pressure, In Pvapor,foreachrun.Reporttheresultsofthelasttwocalculationstoonemore significant digit than allowed by the rules for significant digits. Refer to TREATMENT OF DATA for guidance on significant digits and logarithms. VAPOR PRESSURE AND HEAT OF VAPORIZATION DISCUSSION (Reference: Measurement, Uncertainty, and Significant Figures; Graphing Basics; Excel Graphing Primer. BLBMWS, Ch 11 sections 4-5 and Ch 10 sections 3-6.) The vapor pressure of a liquid is the pressure exerted by its vapor in equilibrium with its liquid phase. Equilibrium is achieved when the rates of the opposing processes, vaporization, and condensation, become equal. When this occurs, the vapor pressure becomes constant (if the temperature stays constant), and if the temperature of the liquid increases the vapor pressure also increases. This situation is more completely described as a state of dynamic equilibrium two opposing processes occurring at the same rate. When the vapor pressure becomes equal to the opposing (applied) pressure, the liquid boils. When the opposing pressure is one atmosphere (760mmHg), this temperature is called the normal boiling point of the liquid. Many organic liquids are volatile and have measurable vapor pressures at or near room temperature, 25C. The heat (enthalpy) of vaporization of a liquid can be figured out from such measurements using the Clausius-Clapeyron equation: (1) lnP=(RTHvap)+C=(RHvapT1)+C where: PHvapRTC=vaporpressure,mmHg=molarheatofvaporization,kJ/mol=gasconstant=8.314J/molK=8.314103kJ/molK=Kelvintemperature,K=aconstant According to equation 1 , a plot of lnPvsT1 is linear. And the slope, m, of this line equals: (2) m=RHvap Hence, Hvap is readily obtained from such a graph by finding the slope and substituting its value into equation 2 and solving. An estimate of the normal boiling point of the liquid can be found by solving the regression equation (trendline) for T at a vapor pressure of 760mmHg. In this experiment, you will determine the vapor pressure of isopropyl alcohol, C3H8O, at four different temperatures. When this data is graphed as described above the Hvap is determined from the slope (equation 2) and the normal boiling point is found using the regression equation (trendline) by solving it for T at 760mmHg. The experimental setup consists of a 125-mL Erlenmeyer flask connected to a gas pressure sensor which in turn is connected to a LabQuest 2 (or LabQuest) handheld device that displays the measured pressure. The flask is immersed in a constant temperature water bath to bring it to the desired temperature and to allow for pressure equilibration. It is then sealed, and a small volume of isopropyl alcohol is introduced into it without allowing air to enter or leave the flask. The pressure inside the flask will begin to increase as some of the alcohol vaporizes. The pressure change will be detected by the gas pressure sensor and the new pressure displayed in real-time on the handheld device. The pressure inside the flask will continue to increase until equilibrium (constant pressure) is achieved between liquid and gaseous alcohol NOTE: The data used here are for illustrative purposes. The data you obtain will be different. Complete the following table. Record the beginning and ending water bath temperatures as well as the beginning and equilibrium pressures for each run. Calculate the average temperature, C, and vapor pressure, mmHg, for each run. Vapor pressure, Pvap=PequilPinitial.. Enter Tavg and Pvapor from the preceding table in the following table. Complete the table by calculating the average temperature in degrees Kelvin, the inverse of the average temperature in degrees Kelvin, Tavg1,K1 and the natural logarithm of the vapor pressure, In Pvapor,foreachrun.Reporttheresultsofthelasttwocalculationstoonemore significant digit than allowed by the rules for significant digits. Refer to TREATMENT OF DATA for guidance on significant digits and logarithms. VAPOR PRESSURE AND HEAT OF VAPORIZATION DISCUSSION (Reference: Measurement, Uncertainty, and Significant Figures; Graphing Basics; Excel Graphing Primer. BLBMWS, Ch 11 sections 4-5 and Ch 10 sections 3-6.) The vapor pressure of a liquid is the pressure exerted by its vapor in equilibrium with its liquid phase. Equilibrium is achieved when the rates of the opposing processes, vaporization, and condensation, become equal. When this occurs, the vapor pressure becomes constant (if the temperature stays constant), and if the temperature of the liquid increases the vapor pressure also increases. This situation is more completely described as a state of dynamic equilibrium two opposing processes occurring at the same rate. When the vapor pressure becomes equal to the opposing (applied) pressure, the liquid boils. When the opposing pressure is one atmosphere (760mmHg), this temperature is called the normal boiling point of the liquid. Many organic liquids are volatile and have measurable vapor pressures at or near room temperature, 25C. The heat (enthalpy) of vaporization of a liquid can be figured out from such measurements using the Clausius-Clapeyron equation: (1) lnP=(RTHvap)+C=(RHvapT1)+C where: PHvapRTC=vaporpressure,mmHg=molarheatofvaporization,kJ/mol=gasconstant=8.314J/molK=8.314103kJ/molK=Kelvintemperature,K=aconstant According to equation 1 , a plot of lnPvsT1 is linear. And the slope, m, of this line equals: (2) m=RHvap Hence, Hvap is readily obtained from such a graph by finding the slope and substituting its value into equation 2 and solving. An estimate of the normal boiling point of the liquid can be found by solving the regression equation (trendline) for T at a vapor pressure of 760mmHg. In this experiment, you will determine the vapor pressure of isopropyl alcohol, C3H8O, at four different temperatures. When this data is graphed as described above the Hvap is determined from the slope (equation 2) and the normal boiling point is found using the regression equation (trendline) by solving it for T at 760mmHg. The experimental setup consists of a 125-mL Erlenmeyer flask connected to a gas pressure sensor which in turn is connected to a LabQuest 2 (or LabQuest) handheld device that displays the measured pressure. The flask is immersed in a constant temperature water bath to bring it to the desired temperature and to allow for pressure equilibration. It is then sealed, and a small volume of isopropyl alcohol is introduced into it without allowing air to enter or leave the flask. The pressure inside the flask will begin to increase as some of the alcohol vaporizes. The pressure change will be detected by the gas pressure sensor and the new pressure displayed in real-time on the handheld device. The pressure inside the flask will continue to increase until equilibrium (constant pressure) is achieved between liquid and gaseous alcohol