I need help with part 2 problem 2. I have PowerPoint slides for reference.

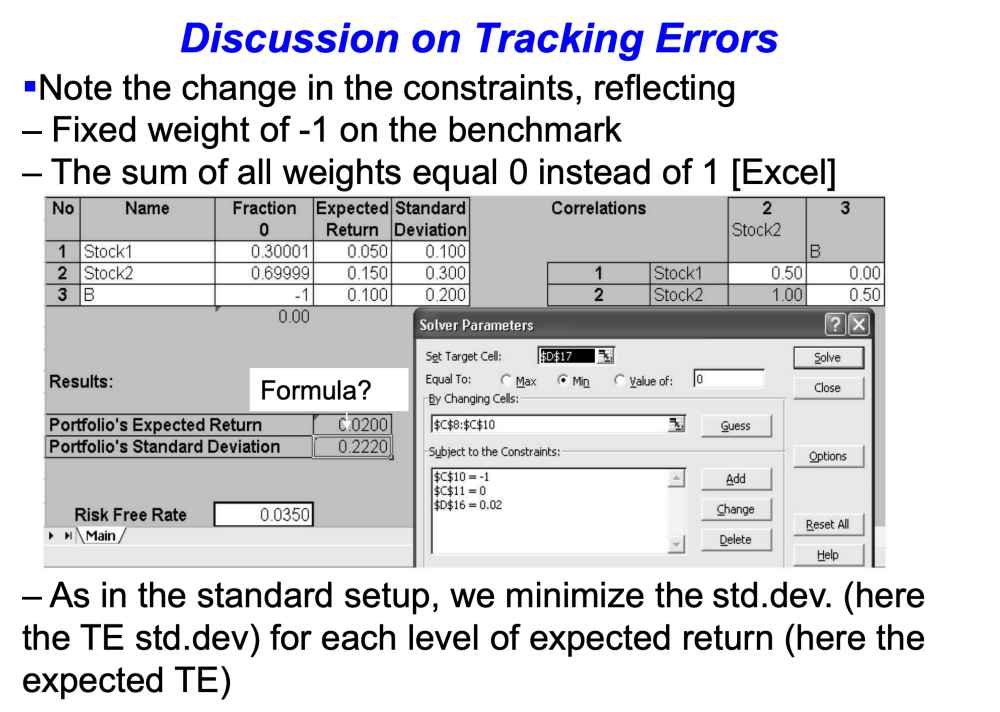
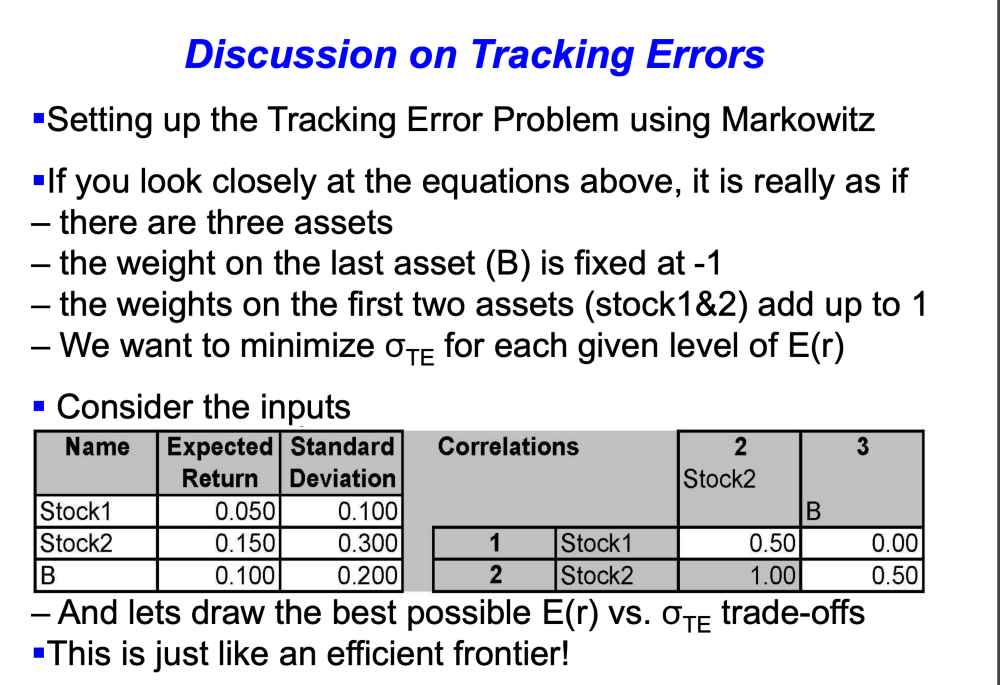
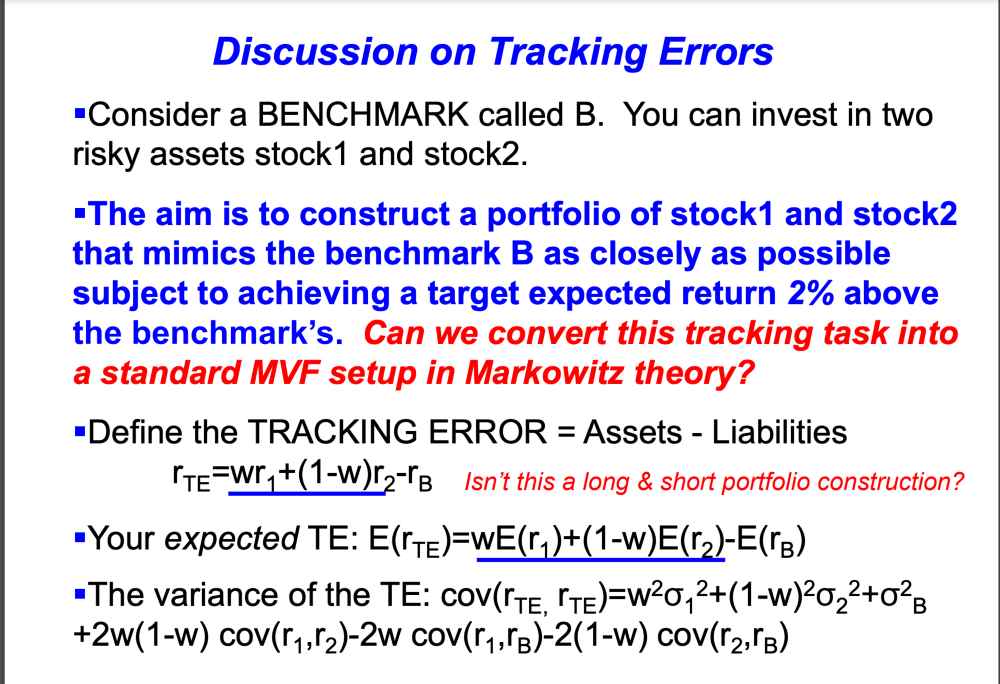
-Setting up the Tracking Error Problem using Markowitz - If you look closely at the equations above, it is really as if - there are three assets - the weight on the last asset (B) is fixed at -1 - the weights on the first two assets (stock1\&2) add up to 1 - We want to minimize TE for each given level of E(r) - Consider the inputs - And lets draw the best possible E(r) vs. TE trade-offs -This is just like an efficient frontier! Discussion on Tracking Errors -Note the change in the constraints, reflecting - Fixed weight of -1 on the benchmark - The sum of all weights equal 0 instead of 1 [Excel] \begin{tabular}{ll|} \hline & Results: \\ & Formula? \\ & \\ Risk Free Rate & \\ 1. Main/ \end{tabular} - As in the standard setup, we minimize the std.dev. (here the TE std.dev) for each level of expected return (here the expected TE) Discussion on Tracking Errors - Consider a BENCHMARK called B. You can invest in two risky assets stock1 and stock2. -The aim is to construct a portfolio of stock1 and stock2 that mimics the benchmark B as closely as possible subject to achieving a target expected return 2% above the benchmark's. Can we convert this tracking task into a standard MVF setup in Markowitz theory? -Define the TRACKING ERROR = Assets - Liabilities rTE=wr1+(1w)r2rB Isn't this a long \& short portfolio construction? -Your expected TE: E(rTE)=wE(r1)+(1w)E(r2)E(rB) - The variance of the TE: cov(rTE,rTE)=w212+(1w)222+BB +2w(1w)cov(r1,r2)2wcov(r1,rB)2(1w)cov(r2,rB) Part II. Application of Multi-Factor Models in Portfolio Management (Lecture 5) Suppose you are the manager of the fund of domestic equity funds. - You have no stock picking ability - You do have factor forecasting/timing ability Consider investing in six portfolios, formed by sorting all stocks according to their market capitalization and book-to-market ratio: S/L (small growth), S/M (small core), S/H (small value), B/L (large growth), B/M (large core), and B/H (large value). - You believe the following four-factor model holds: - FRR is the excess return on the stock market index - FTs is the yield spread between 10-year T-bond and 1-year T-bill (constant maturity). - F Fs is the yield spread between Moody's Seasoned Baa and Aaa corporate bonds. - For is Oil inflation (percentage change in Spot Crude Oil Prices: WTI). (The data for FTS,FYS,FOL is from http://research.stlouisfed.org) Following the discussion in class, use the data in the spreadsheet file "fundret.xls" to answer questions below. (1) Suppose that at the beginning of Year 2023 you want to minimize your total portfolio variance and target at least 12% per year in expected returns, but 1. You would not take oil-price risk. 2. You would like your portfolio to move one-to-one with the market. How do you construct such a portfolio? Instruction: Step 1: Estimate each underlying asset's factor loadings Step 2: Construct each underlying asset's expected (excess) returns based on the 4-factor model Step 3: Construct the covariance matrix of 4 factors Step 4: Construct underlying assets' return covariance matrix based on the 4-factor model Step 5: Set up the constraints Step 6: Set up the solver in Excel (2) Suppose that at the beginning of Year 2023 you create an optimal portfolio tracking Russell 3000 and earning at least 2% per year above the expected return on the Russell 3000 . You want to minimize the variance of the tracking errors. What is your optimal portfolio? What is the factor sensitivity of your tracking errors to these risk factors? (3) Same as the (2). Additionally, you want to neutralize the tracking error risk exposures to both FRMRF and FOi. What is your new optimal portfolio? (4) Suppose that at the beginning of Year 2023 you create a " 2x Russell 3000 Index" ETF, which is a portfolio delivering twice the returns of Russell 3000 Index. You want to minimize the variance of the tracking errors. What is your optimal portfolio? What is the factor sensitivity of your tracking errors? (In practice, leveraged ETFs might invest in equity index swaps, index futures, and options on securities.) 2 -Setting up the Tracking Error Problem using Markowitz - If you look closely at the equations above, it is really as if - there are three assets - the weight on the last asset (B) is fixed at -1 - the weights on the first two assets (stock1\&2) add up to 1 - We want to minimize TE for each given level of E(r) - Consider the inputs - And lets draw the best possible E(r) vs. TE trade-offs -This is just like an efficient frontier! Discussion on Tracking Errors -Note the change in the constraints, reflecting - Fixed weight of -1 on the benchmark - The sum of all weights equal 0 instead of 1 [Excel] \begin{tabular}{ll|} \hline & Results: \\ & Formula? \\ & \\ Risk Free Rate & \\ 1. Main/ \end{tabular} - As in the standard setup, we minimize the std.dev. (here the TE std.dev) for each level of expected return (here the expected TE) Discussion on Tracking Errors - Consider a BENCHMARK called B. You can invest in two risky assets stock1 and stock2. -The aim is to construct a portfolio of stock1 and stock2 that mimics the benchmark B as closely as possible subject to achieving a target expected return 2% above the benchmark's. Can we convert this tracking task into a standard MVF setup in Markowitz theory? -Define the TRACKING ERROR = Assets - Liabilities rTE=wr1+(1w)r2rB Isn't this a long \& short portfolio construction? -Your expected TE: E(rTE)=wE(r1)+(1w)E(r2)E(rB) - The variance of the TE: cov(rTE,rTE)=w212+(1w)222+BB +2w(1w)cov(r1,r2)2wcov(r1,rB)2(1w)cov(r2,rB) Part II. Application of Multi-Factor Models in Portfolio Management (Lecture 5) Suppose you are the manager of the fund of domestic equity funds. - You have no stock picking ability - You do have factor forecasting/timing ability Consider investing in six portfolios, formed by sorting all stocks according to their market capitalization and book-to-market ratio: S/L (small growth), S/M (small core), S/H (small value), B/L (large growth), B/M (large core), and B/H (large value). - You believe the following four-factor model holds: - FRR is the excess return on the stock market index - FTs is the yield spread between 10-year T-bond and 1-year T-bill (constant maturity). - F Fs is the yield spread between Moody's Seasoned Baa and Aaa corporate bonds. - For is Oil inflation (percentage change in Spot Crude Oil Prices: WTI). (The data for FTS,FYS,FOL is from http://research.stlouisfed.org) Following the discussion in class, use the data in the spreadsheet file "fundret.xls" to answer questions below. (1) Suppose that at the beginning of Year 2023 you want to minimize your total portfolio variance and target at least 12% per year in expected returns, but 1. You would not take oil-price risk. 2. You would like your portfolio to move one-to-one with the market. How do you construct such a portfolio? Instruction: Step 1: Estimate each underlying asset's factor loadings Step 2: Construct each underlying asset's expected (excess) returns based on the 4-factor model Step 3: Construct the covariance matrix of 4 factors Step 4: Construct underlying assets' return covariance matrix based on the 4-factor model Step 5: Set up the constraints Step 6: Set up the solver in Excel (2) Suppose that at the beginning of Year 2023 you create an optimal portfolio tracking Russell 3000 and earning at least 2% per year above the expected return on the Russell 3000 . You want to minimize the variance of the tracking errors. What is your optimal portfolio? What is the factor sensitivity of your tracking errors to these risk factors? (3) Same as the (2). Additionally, you want to neutralize the tracking error risk exposures to both FRMRF and FOi. What is your new optimal portfolio? (4) Suppose that at the beginning of Year 2023 you create a " 2x Russell 3000 Index" ETF, which is a portfolio delivering twice the returns of Russell 3000 Index. You want to minimize the variance of the tracking errors. What is your optimal portfolio? What is the factor sensitivity of your tracking errors? (In practice, leveraged ETFs might invest in equity index swaps, index futures, and options on securities.) 2