i need help with these too!

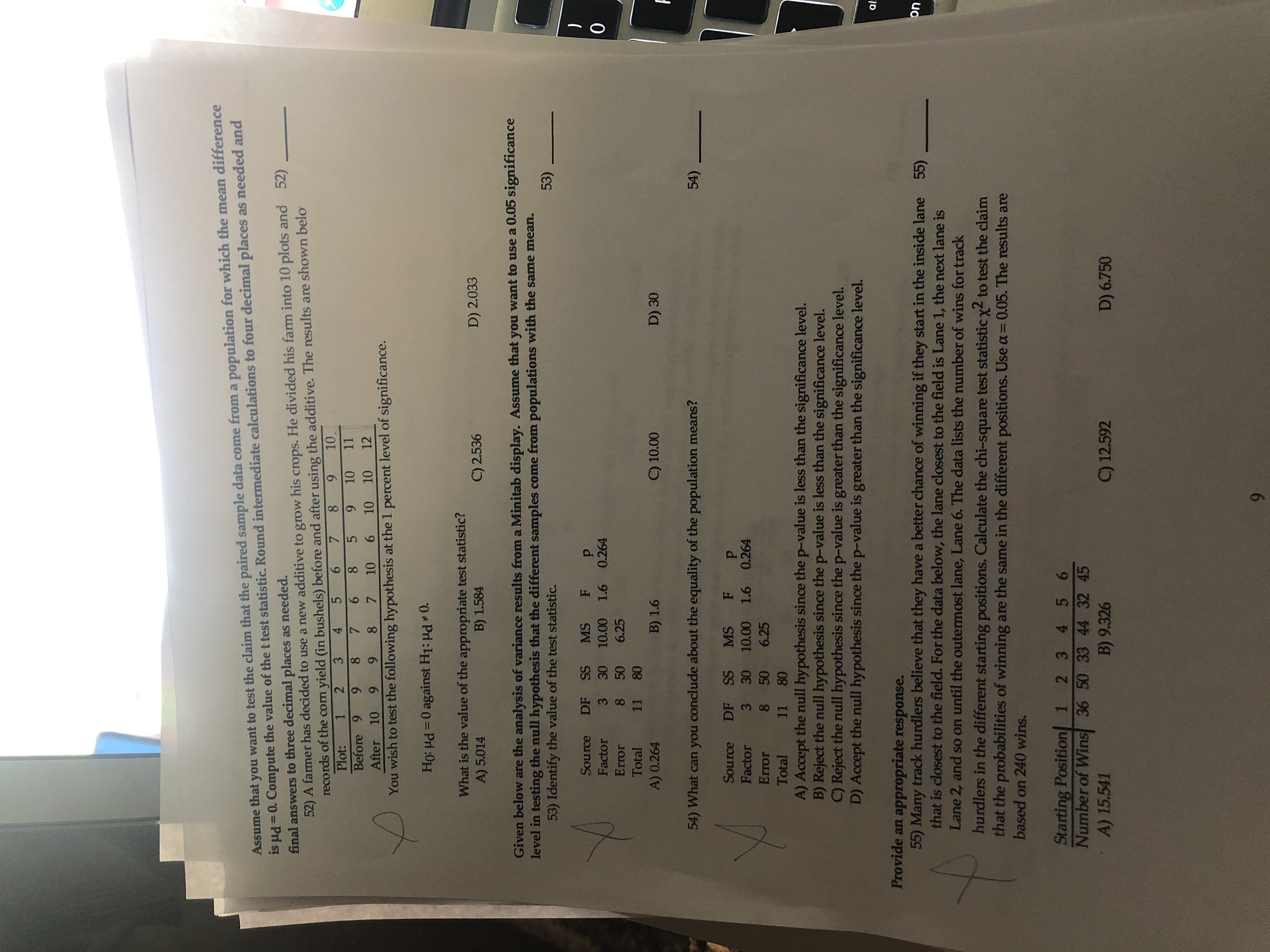
Assume that you want to test the claim that the paired sample data come from a population for which the mean difference is Hd = 0. Compute the value of the t test statistic. Round intermediate calculations to four decimal places as needed and final answers to three decimal places as needed. 52) A farmer has decided to use a new additive to grow his crops. He divided his farm into 10 plots and 52) records of the corn yield (in bushels) before and after using the additive. The results are shown belo Plot: 1 Before 9 3 5 6 7 After 10 9 6 8 8 5 9 9 10 10 6 10 11 10 10 12 You wish to test the following hypothesis at the 1 percent level of significance. Ho: Md = 0 against H1: Md * 0. What is the value of the appropriate test statistic? A) 5.014 B) 1.584 C) 2.536 D) 2.033 Given below are the analysis of variance results from a Minitab display. Assume that you want to use a 0.05 significance level in testing the null hypothesis that the different samples come from populations with the same mean. 53) Identify the value of the test statistic. 53) Source DF SS MS F Factor 3 30 10.00 1.6 0.264 Error 8 50 6.25 Total 11 80 A) 0.264 B) 1.6 C) 10.00 D) 30 54) What can you conclude about the equality of the population means? 54) Source DF SS MS F Factor 3 30 10.00 1.6 0.264 Error 8 50 6.25 Total 11 80 A) Accept the null hypothesis since the p-value is less than the significance level. B) Reject the null hypothesis since the p-value is less than the significance level. C) Reject the null hypothesis since the p-value is greater than the significance level. D) Accept the null hypothesis since the p-value is greater than the significance level. Provide an appropriate response. 55) Many track hurdlers believe that they have a better chance of winning if they start in the inside lane 55) that is closest to the field. For the data below, the lane closest to the field is Lane 1, the next lane is Lane 2, and so on until the outermost lane, Lane 6. The data lists the number of wins for track hurdlers in the different starting positions. Calculate the chi-square test statistic x2 to test the claim based on 240 wins. that the probabilities of winning are the same in the different positions. Use a = 0.05. The results are Starting Position 1 2 3 4 5 6 Number of Wins 36 50 33 44 32 45 A) 15.541 B) 9.326 C) 12.592 D) 6.750