I need the answer to question 2.12 from Sreve's Mathematics of Finance 1. Iam attaching problem 2.11 as well sice it seems problem 2.12 needs us to use soem theory from problem 2.11.

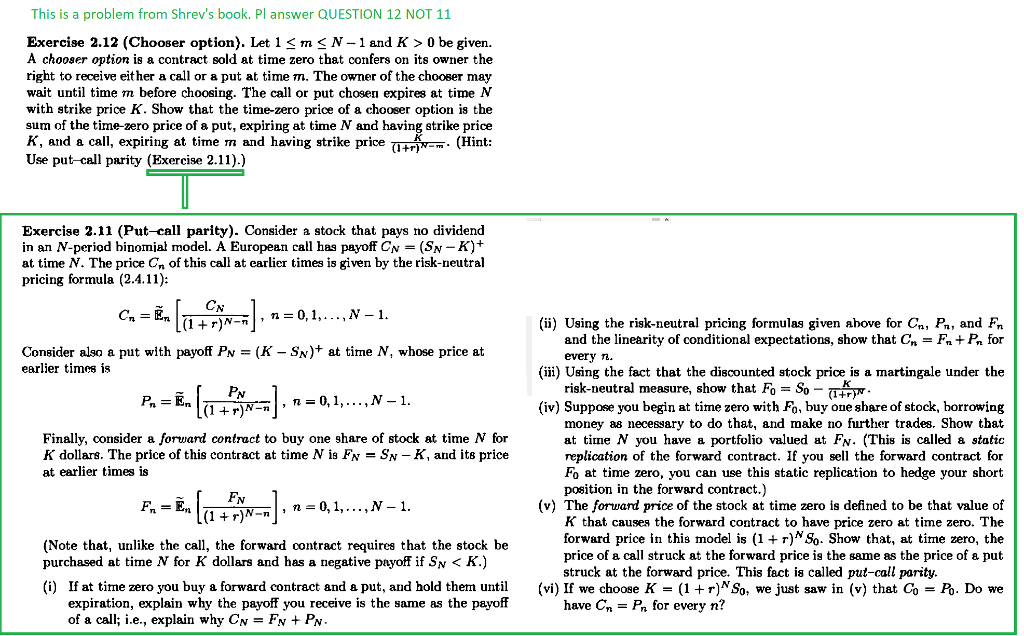
This is a problem from Shrev's book. Pl answer QUESTION 12 NOT 11 Exercise 2.12 (Chooser option). Let 1 0 be given. A chooser option is a contract sold at time zero that confers on its owner the right to receive either a call or a put at time m. The owner of the chooser may wait until time m before choosing. The call or put chosen expires at time N with strike price K. Show that the time-zero price of a chooser option is the sum of the time-zero price of a put, expiring at time N and having strike price K, and a call, expiring at time m and having strike price TH . (Hint Use put-call parity (Exercise 2.11).) Exercise 2.11 (Put-call parity). Consider a stock that pays no dividend in an N-period binomial model. A European call has payoff CN = (SN + at time N. The price Cn of this call at earlier times is given by the risk-neutral pricing formula (2.4.11) Cv (ii) Using the risk-neutral pricing formulas given above for Cn, Pn, and Fni (iii) Using the fact that the discounted stock price is a martingale under the (iv) Suppose you begin at time zero with Fo, buy one share of stock, borrowing Consider also a put with payoff P earlier times is and the linearity of conditional expectations, show that Cn- Fn+Pa for every n. (K - SN)+ at time N, whose price at risk-neutral measure, show that F So- 1 +r)N- Finally, consider a forward contract to buy one share of stock at time N for K dollars. The price of this contract at time N is FN SN - K, and its price at earlier times is money as necessary to do that, and make no further trades. Show that at time N you have a portfolio valued at Fv. (This is called a static replication of the forward contract. If you sell the forward contract for Fo at time zero, you can use this static replication to hedge your short position in the forward contract.) (v) The forward price of the stock at time zero is defined to be that value of K that causes the forward contract to have price zero at time zero. The forward price in this model is (1 +r)NSo. Show that, at time zero, the price of a call struck at the forward price is the same as the price of a put struck at the forward price. This fsct is called put-call parity (Note that, unlike the call, the forward contract requires that the stock be purchased at time N for K dollars and has a negative payoff if Sn 0 be given. A chooser option is a contract sold at time zero that confers on its owner the right to receive either a call or a put at time m. The owner of the chooser may wait until time m before choosing. The call or put chosen expires at time N with strike price K. Show that the time-zero price of a chooser option is the sum of the time-zero price of a put, expiring at time N and having strike price K, and a call, expiring at time m and having strike price TH . (Hint Use put-call parity (Exercise 2.11).) Exercise 2.11 (Put-call parity). Consider a stock that pays no dividend in an N-period binomial model. A European call has payoff CN = (SN + at time N. The price Cn of this call at earlier times is given by the risk-neutral pricing formula (2.4.11) Cv (ii) Using the risk-neutral pricing formulas given above for Cn, Pn, and Fni (iii) Using the fact that the discounted stock price is a martingale under the (iv) Suppose you begin at time zero with Fo, buy one share of stock, borrowing Consider also a put with payoff P earlier times is and the linearity of conditional expectations, show that Cn- Fn+Pa for every n. (K - SN)+ at time N, whose price at risk-neutral measure, show that F So- 1 +r)N- Finally, consider a forward contract to buy one share of stock at time N for K dollars. The price of this contract at time N is FN SN - K, and its price at earlier times is money as necessary to do that, and make no further trades. Show that at time N you have a portfolio valued at Fv. (This is called a static replication of the forward contract. If you sell the forward contract for Fo at time zero, you can use this static replication to hedge your short position in the forward contract.) (v) The forward price of the stock at time zero is defined to be that value of K that causes the forward contract to have price zero at time zero. The forward price in this model is (1 +r)NSo. Show that, at time zero, the price of a call struck at the forward price is the same as the price of a put struck at the forward price. This fsct is called put-call parity (Note that, unlike the call, the forward contract requires that the stock be purchased at time N for K dollars and has a negative payoff if Sn