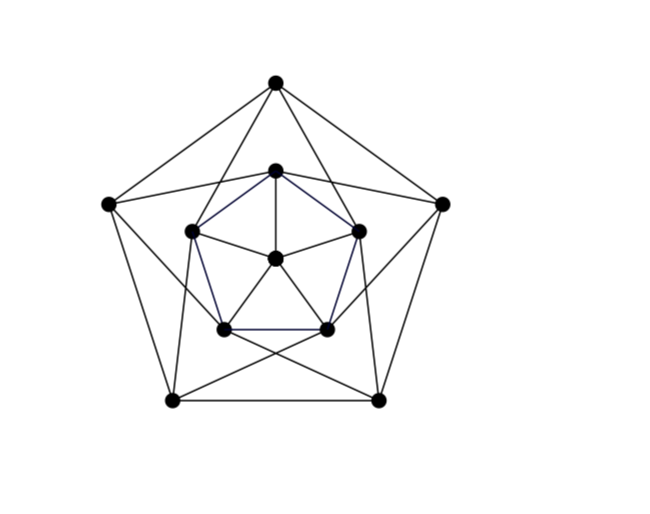
I need to prove that this graph is not planar by using the Euler formula where v - vertices f-faces e-edge

v-e+f =2 and so we know that it is planar if 3f

The graph has

v - 11

e-25

by Euler for

mula be f-16

because faces need to be counted on a plane graph we know its not plane so we can't count them so we use the formula to find the faces

I get 48

But it does not work for this graph.

Im wondering if my inequalities are correct if it should be different from 3f

but I'm nor entirely sure can someone please explain how I would do this

\f