I only need part 3B, 3A is already on chegg.
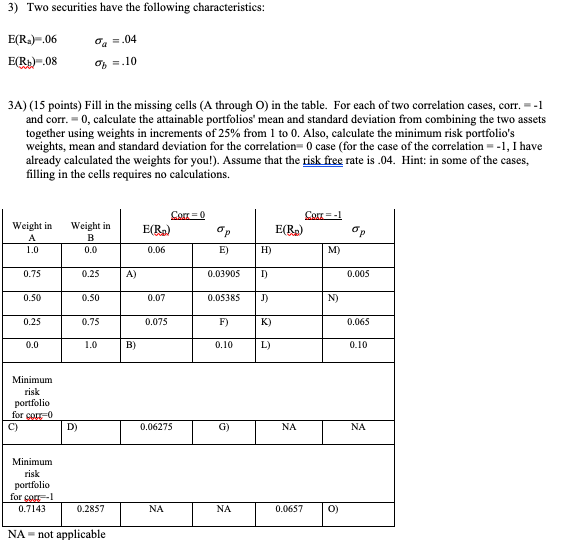
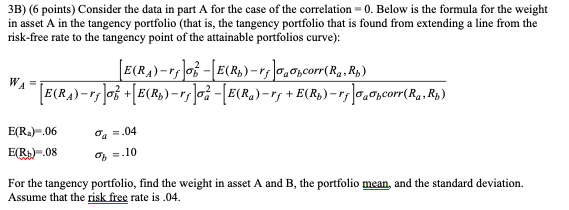
3) Two securities have the following characteristics: E(Ra)-.06 E(Rp).08 a =.04 0 = 10 3A) (15 points) Fill in the missing cells (A through O) in the table. For each of two correlation cases, corr. = -1 and corr. -0, calculate the attainable portfolios' mean and standard deviation from combining the two assets together using weights in increments of 25% from 1 to 0. Also, calculate the minimum risk portfolio's weights, mean and standard deviation for the correlation-0 case (for the case of the correlation -1, I have already calculated the weights for you!). Assume that the risk free rate is .04. Hint: in some of the cases, filling in the cells requires no calculations. ORTO = -1 Weight in Weight in op 1.0 0.0 0.75 0.005 E(R) E(Rp) 0.06 EH) 0.03905D 0.07 0.05385 ) 0.075F ) K) B) 0.10 ) 0.50 0.50 0.07 N) 0.25 0.75 0.065 0.0 1.0 0.10 Minimum risk portfolio for cor=0 0.06275 G) NA NA Minimum risk portfolio for co -1 0.7143 0.2857 NA = not applicable E(R)-.06 E(R)-.08 0 .04 ) = 10 Work for question 1: 3B) (6 points) Consider the data in part A for the case of the correlation-0. Below is the formula for the weight in asset A in the tangency portfolio (that is, the tangency portfolio that is found from extending a line from the risk-free rate to the tangency point of the attainable portfolios curve): E(RA)-rslo - E(R)-roncorr(R , R) "" (E(R) - ry Jo +[E(Rp)-rs]o2 -[E(ra)r; + E(R))=r; Joecorr(Ra, Ro) E(R.)-.06 E(Rp).08 =.04 = 10 0 For the tangency portfolio, find the weight in asset A and B, the portfolio mean, and the standard deviation. Assume that the risk free rate is .04. 3) Two securities have the following characteristics: E(Ra)-.06 E(Rp).08 a =.04 0 = 10 3A) (15 points) Fill in the missing cells (A through O) in the table. For each of two correlation cases, corr. = -1 and corr. -0, calculate the attainable portfolios' mean and standard deviation from combining the two assets together using weights in increments of 25% from 1 to 0. Also, calculate the minimum risk portfolio's weights, mean and standard deviation for the correlation-0 case (for the case of the correlation -1, I have already calculated the weights for you!). Assume that the risk free rate is .04. Hint: in some of the cases, filling in the cells requires no calculations. ORTO = -1 Weight in Weight in op 1.0 0.0 0.75 0.005 E(R) E(Rp) 0.06 EH) 0.03905D 0.07 0.05385 ) 0.075F ) K) B) 0.10 ) 0.50 0.50 0.07 N) 0.25 0.75 0.065 0.0 1.0 0.10 Minimum risk portfolio for cor=0 0.06275 G) NA NA Minimum risk portfolio for co -1 0.7143 0.2857 NA = not applicable E(R)-.06 E(R)-.08 0 .04 ) = 10 Work for question 1: 3B) (6 points) Consider the data in part A for the case of the correlation-0. Below is the formula for the weight in asset A in the tangency portfolio (that is, the tangency portfolio that is found from extending a line from the risk-free rate to the tangency point of the attainable portfolios curve): E(RA)-rslo - E(R)-roncorr(R , R) "" (E(R) - ry Jo +[E(Rp)-rs]o2 -[E(ra)r; + E(R))=r; Joecorr(Ra, Ro) E(R.)-.06 E(Rp).08 =.04 = 10 0 For the tangency portfolio, find the weight in asset A and B, the portfolio mean, and the standard deviation. Assume that the risk free rate is .04









