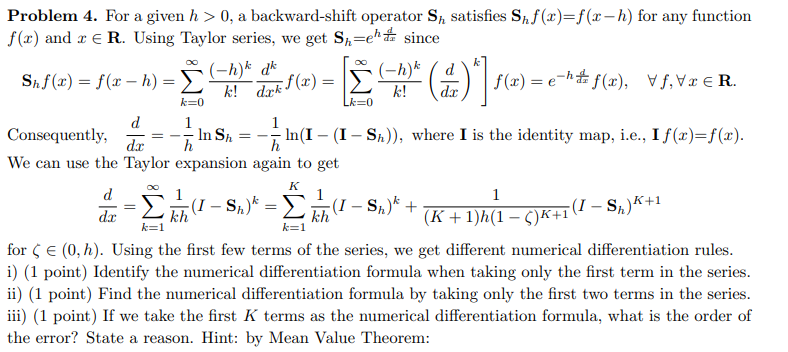
i) Verify that g(x, h)=f(x+h)2f(x)+f(xh), i.e., the central difference approxi- mation to f"(x), satisfies g(x, h)=f"(x)+(4)(x) + O(h) (thus the error is O(h)). ii) (1 point) For smooth f, use extrapolation technique to find a difference formula for f"(z) that has an error order O(h). iii) (1 point) Using the formula obtained in ii) and the data ZU {(0.2, 1.7)} to estimate the second derivative at x=0.2 with h = 0.2. Problem 4. For a given h > 0, a backward-shift operator S satisfies Sf(x)=f(x-h) for any function f(x) and R. Using Taylor series, we get Sheh Shf(x) = f(xh) = Consequently, d dx = (-h)k dk k! dk f(x) = k=0 In Sh h = 1 since (-h)k k! [()] | f(x)= ef(x), V, Vx R. In(I (I-Sh)), where I is the identity map, i.e., I f(x)=(x). - We can use the Taylor expansion again to get for d dx = k=1 K 1 (1 - S)* = (1 - S) * + kh kh k=1 1 - (K+1)h(1 C)K+1 (I Sh) K+1 (0, h). Using the first few terms of the series, we get different numerical differentiation rules. i) (1 point) Identify the numerical differentiation formula when taking only the first term in the series. ii) (1 point) Find the numerical differentiation formula by taking only the first two terms in the series. iii) (1 point) If we take the first K terms as the numerical differentiation formula, what is the order of the error? State a reason. Hint: by Mean Value Theorem: