I will give a thumb : )

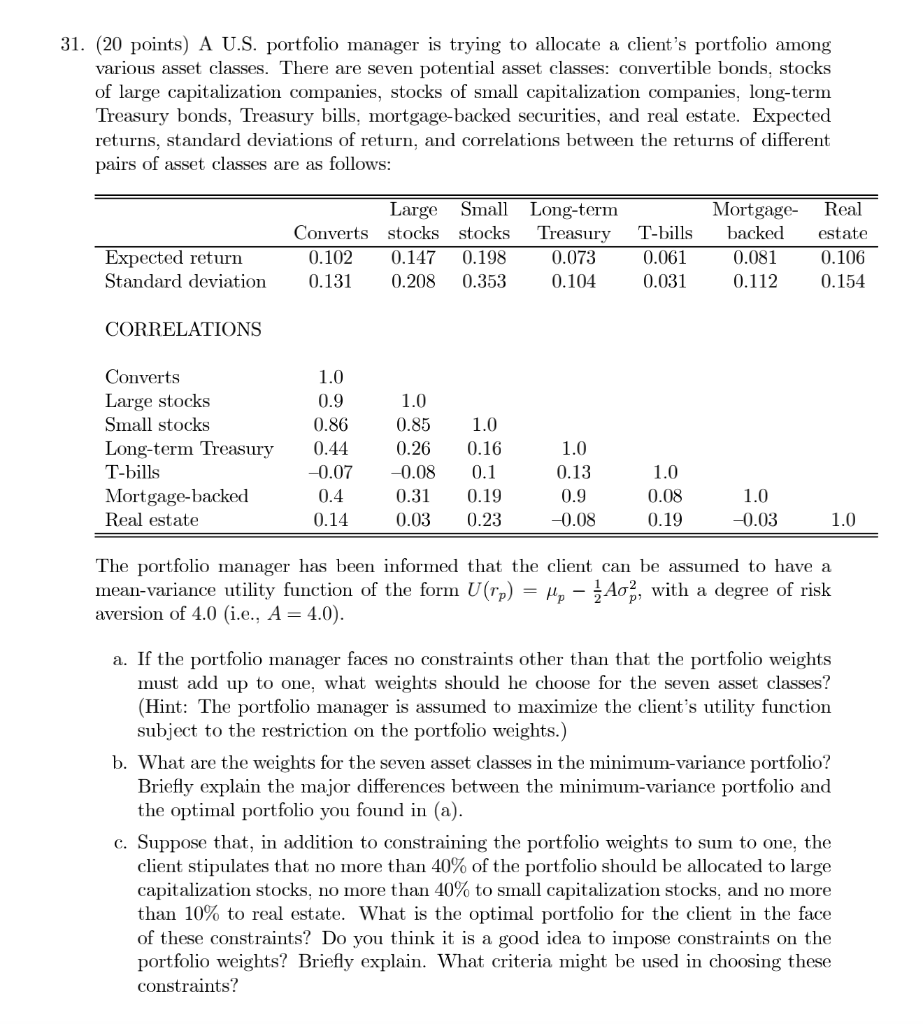
31. (20 points) A U.S. portfolio manager is trying to allocate a client's portfolio among various asset classes. There are seven potential asset classes: convertible bonds, stocks of large capitalization companies, stocks of small capitalization companies, long-term Treasury bonds, Treasury bills, mortgage-backed securities, and real estate. Expected returns, standard deviations of return, and correlations between the returns of different pairs of asset classes are as follows: Large Converts stocks 0.102 0.147 0.131 0.208 Small stocks 0.198 0.353 Long-term Treasury 0.073 0.104 Mortgage- backed 0.081 0.112 T-bills 0.061 0.031 Real estate 0.106 0.154 Expected return Standard deviation CORRELATIONS Converts Large stocks Small stocks Long-term Treasury T-bills Mortgage-backed Real estate 1.0 0.9 0.86 0.44 -0.07 0.4 0.14 1.0 0.85 0.26 -0.08 0.31 0.03 1.0 0.16 0.1 0.19 0.23 1.0 0.13 0.9 -0.08 1.0 0.08 0.19 1.0 -0.03 1.0 The portfolio manager has been informed that the client can be assumed to have a mean-variance utility function of the form U (rp) = Mp A0%, with a degree of risk aversion of 4.0 (i.e., A= 4.0). a. If the portfolio manager faces no constraints other than that the portfolio weights must add up to one, what weights should he choose for the seven asset classes? (Hint: The portfolio manager is assumed to maximize the client's utility function subject to the restriction on the portfolio weights.) b. What are the weights for the seven asset classes in the minimum-variance portfolio? Briefly explain the major differences between the minimum-variance portfolio and the optimal portfolio you found in (a). c. Suppose that, in addition to constraining the portfolio weights to sum to one, the client stipulates that no more than 40% of the portfolio should be allocated to large capitalization stocks, no more than 40% to small capitalization stocks, and no more than 10% to real estate. What is the optimal portfolio for the client in the face of these constraints? Do you think it is a good idea to impose constraints on the portfolio weights? Briefly explain. What criteria might be used in choosing these constraints? 31. (20 points) A U.S. portfolio manager is trying to allocate a client's portfolio among various asset classes. There are seven potential asset classes: convertible bonds, stocks of large capitalization companies, stocks of small capitalization companies, long-term Treasury bonds, Treasury bills, mortgage-backed securities, and real estate. Expected returns, standard deviations of return, and correlations between the returns of different pairs of asset classes are as follows: Large Converts stocks 0.102 0.147 0.131 0.208 Small stocks 0.198 0.353 Long-term Treasury 0.073 0.104 Mortgage- backed 0.081 0.112 T-bills 0.061 0.031 Real estate 0.106 0.154 Expected return Standard deviation CORRELATIONS Converts Large stocks Small stocks Long-term Treasury T-bills Mortgage-backed Real estate 1.0 0.9 0.86 0.44 -0.07 0.4 0.14 1.0 0.85 0.26 -0.08 0.31 0.03 1.0 0.16 0.1 0.19 0.23 1.0 0.13 0.9 -0.08 1.0 0.08 0.19 1.0 -0.03 1.0 The portfolio manager has been informed that the client can be assumed to have a mean-variance utility function of the form U (rp) = Mp A0%, with a degree of risk aversion of 4.0 (i.e., A= 4.0). a. If the portfolio manager faces no constraints other than that the portfolio weights must add up to one, what weights should he choose for the seven asset classes? (Hint: The portfolio manager is assumed to maximize the client's utility function subject to the restriction on the portfolio weights.) b. What are the weights for the seven asset classes in the minimum-variance portfolio? Briefly explain the major differences between the minimum-variance portfolio and the optimal portfolio you found in (a). c. Suppose that, in addition to constraining the portfolio weights to sum to one, the client stipulates that no more than 40% of the portfolio should be allocated to large capitalization stocks, no more than 40% to small capitalization stocks, and no more than 10% to real estate. What is the optimal portfolio for the client in the face of these constraints? Do you think it is a good idea to impose constraints on the portfolio weights? Briefly explain. What criteria might be used in choosing these constraints