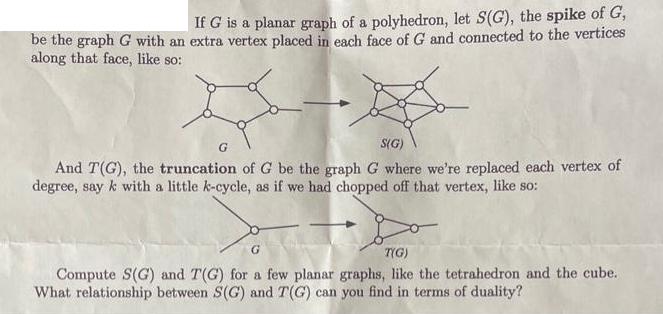
If G is a planar graph of a polyhedron, let S(G), the spike of G, be the graph G with an extra vertex placed in each face of G and connected to the vertices along that face, like so: X-R S(G) And T(G), the truncation of G be the graph G where we're replaced each vertex of degree, say k with a little k-cycle, as if we had chopped off that vertex, like so: G T(G) Compute S(G) and T(G) for a few planar graphs, like the tetrahedron and the cube. What relationship between S(G) and T(G) can you find in terms of duality?