Question: If u is a non-constant real-valued harmonic function in a region , then u cannot attain a maximum (or a minimum) in 22. Suppose

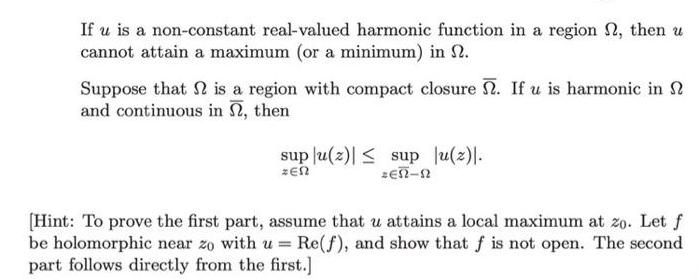
If u is a non-constant real-valued harmonic function in a region , then u cannot attain a maximum (or a minimum) in 22. Suppose that is a region with compact closure . If u is harmonic in and continuous in , then sup |u(2) sup |u(z)|. ZEN - [Hint: To prove the first part, assume that u attains a local maximum at zo. Let f be holomorphic near zo with u = Re(f), and show that f is not open. The second part follows directly from the first.]

Step by Step Solution

★★★★★

3.52 Rating (166 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

s Solution Here we 70n have to assume the ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...

In this exercise, we will explore some logical relationships between families of tastes that satisfy different assumptions. A: Suppose we define a strong and a weak version of convexity as follows:...

In each case either show that the statement is true or give an example showing that it is false. Throughout, X, Y, Z, X1, X2,..., Xn denote vectors in Rn. (a) If U is a subspace of Rn and X+Y is in...

I don't know how to solve the problems. Help me. Pl.l Formally prove the union bound which states that for any events A., A2. ..., AM (not necessarily mutually exclusive) M Pr 52mm]. i=1 M U A: i=1...

71. A certain stock costs 40 today and will pay an annual dividend of 6 for the next 4 years. An investor wishes to purchase a 4-year prepaid forward contract for this stock. The first dividend will...

. Use a double integral to nd the volume of the solid bounded by the cylinder y2 + 2:2 = 2, and the planes 3: : 2y. :I: = U, z : 0 in the first octant. . Find the volume of the wedge cut from the...

Qq123...answer please (a) Represent the IG procedure as an extensive-form game-frame (a sufficiently informative sketch is enough; no need to write the payoffs). (b) Represent the SP procedure as an...

Design a class named Person and its two subclasses named Student and Employee. Make Faculty and Staff subclasses of Employee. A person has a name, address, phone number, and email address. A student...

13.6 Suppose that there are panel data for T = 2 time periods for a randomized controlled experiment, where the first observation (t = 1) is taken before the experiment and the second observation (t...

how do I solve this? Give an example of a non constant vector valued function of three variables r(t) = (x(t), y(t), z(t)) such that r/(t) . r(t) = 0

Wang Distributors has an annual demand for an airport metal detector of 1,410 units. The cost of a typical detector to Wang is $400. Carrying cost is estimated to be 19% of the unit cost, and the...

Explain the principles of delegation

State the importance of motivation

Discuss the various steps involved in the process of planning

What are the challenges associated with tunneling in urban areas?

What are the main differences between rigid and flexible pavements?

4/6 An alphabetical list of Cullumber Company adjusted accounts at its fiscal year end, August 31,2024 , follows. All accounts have normal balances. Additional information: 1. Of the notes payable,...

Imagine that your best friend knows you are taking a psychology course and wonders what psychology is all about. How would you define psychology for your friend? Write an essay on the discipline of...

Distortionary Taxes with Redistribution: Consider a 2-person exchange economy in which I own 200 units of x1 and 100 units of x2 while you own 100 units of x1 and 200 units of x2. A: Suppose you and...

Return to the analysis of my undying love for my wife expressed through weekly purchases of roses (as introduced in end-of-chapter exercise 6.4). A. Recall that initially roses cost $5 each and, with...

Suppose you were just told that you will receive an end-of-the-year bonus of $15,000 from your company. Suppose further that your marginal income tax rate is 33.33% which means that you will have to...

Ross Company is a corporation providing medical diagnostic services. Ross has used the cash method since inception because its gross receipts did not exceed $5,000,000. This year, its average annual...

Mauve Corporation began operations as a farm supplies business and used a fiscal year ending October 31. The company gradually went out of the farm supplies business and into the mail-order Christmas...

Shumpert, Inc., entered into a contract that was to take two years to complete, with an estimated cost of $900,000. The contract price was $1,300,000. Costs of the contract for 2015, the first year,...