I'm a little confused about this. Why is arccos of 0 pi/2, 3pi/2, and 5pi/2? Isn't it just pi/2?

And since time can't be negative, the answer can't be t+v = pi/2

Also, when evaluating an inverse trig function set equal to 0, do I find the inverse trig of both sides (such as shown in this example?)

Thanks, let me know.

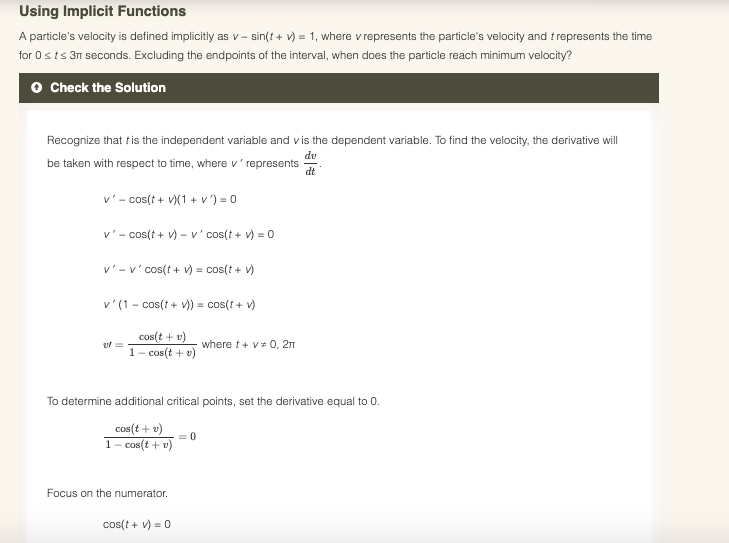
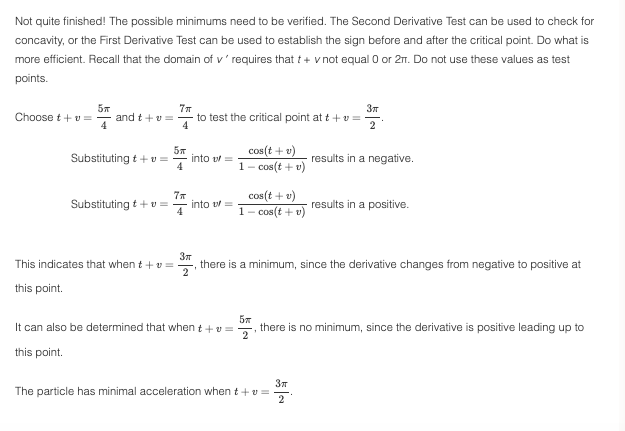
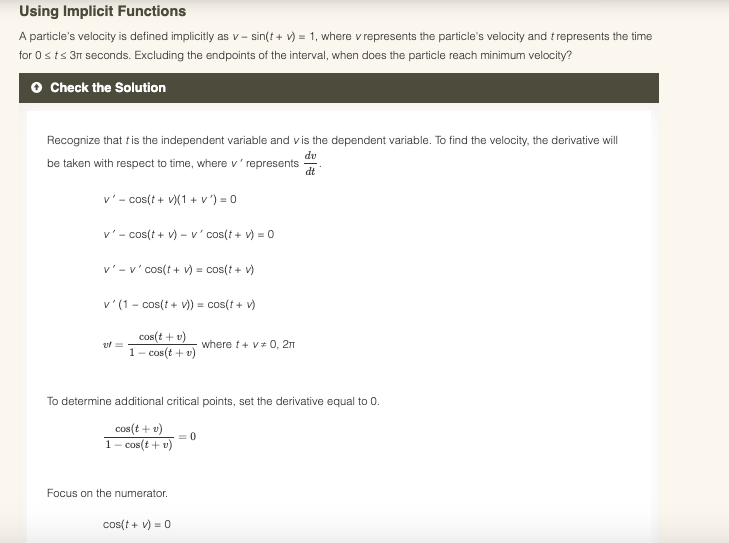
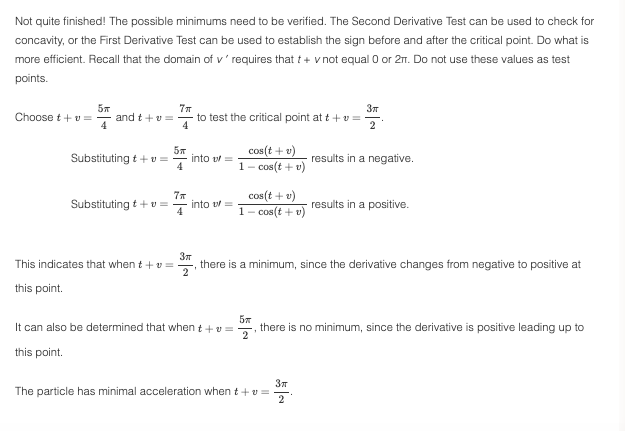
Using Implicit Functions A particle's velocity is defined implicitly as v - sin(t + v) = 1, where vrepresents the particle's velocity and f represents the time for 0 s is 3n seconds. Excluding the endpoints of the interval, when does the particle reach minimum velocity? Check the Solution Recognize that f is the independent variable and vis the dependent variable. To find the velocity, the derivative will be taken with respect to time, where v * represents du dit v ' - cos(t + V)(1 + v ') = 0 v ' - cos(t + v) - v ' cos(t + v) = 0 v ' - v 'cos(t + v) = cos(t + v) v'(1 - cos(t + V)) = cos(t + v) cos(t + v) 1 - cos(t + u) where f + v * 0, 2n To determine additional critical points, set the derivative equal to 0. cos (t + u) 1 - cos(t + v) :0 Focus on the numerator. cos(t + V) = 0Focus on the numerator. cos(t + v) = 0 f + v= cos (0) t+1=. 2'2 2 Now check these possible times by testing each value of : + vin the original implicit function, v - sin(t + v) = 1. t+1= t+1= 2 t+1= 2 v - sin() = 1 J - sin()= 1 U - sin V= 2 - 28 -0.429 1= 4.712 2 2 - 2 # 5.854 - time cannot be negative - may be a minimum - may be a minimum Not quite finished! The possible minimums need to be verified. The Second Derivative Test can be used to check for concavity, or the First Derivative Test can be used to establish the sign before and after the critical point. Do what is more efficient. Recall that the domain of v ' requires that t + wnot equal 0 or 2n. Do not use these values as test points.Not quite finished! The possible minimums need to be verified. The Second Derivative Test can be used to check for concavity, or the First Derivative Test can be used to establish the sign before and after the critical point. Do what is more efficient. Recall that the domain of v ' requires that : + not equal 0 or 21. Do not use these values as test points. Choose t+ v = and t + = - to test the critical point at t + u = 2 cos (t + v) Substituting t + v = into w = 1 - cos(t + v) results in a negative. cos (t + u) Substituting + = into w = 1 - cos(t + v) results in a positive. This indicates that when t + u = 3 IT there is a minimum, since the derivative changes from negative to positive at this point. It can also be determined that when t + u = , there is no minimum, since the derivative is positive leading up to this point. The particle has minimal acceleration when t + u = 2