Imagine that a scientist Jane is in the world as described by The Three-Body Problem. The Trisolaran invasion force has arrived at the Earth secretly and currently they are searching for scientists who may be threatening to them. In fact, Jane estimates that 0.05% of the population is Trisolarans. Jane claims that she has an alien detector which can identify Trisolarans. According to her experience, 95% of the time Trisolarans can be correctly detected, but 10% of the time the detector may report that a person under examination is a Trisolarans but in fact that person is not.

Now a suspect was brought to Jane for examination. Jane intuitively felt that the suspect might be an alien. Confirming her intuition, the detector rang its alarm and reported that the suspect was an alien. However, as a scientist, Jane knew that she needed to apply Bayesian Inference to answer this question: how possible the suspect is really an alien?

Let d be the detector's report.

Let h1 be the hypothesis that the suspect is an alien.

Let h2 be the hypothesis that the suspect is not an alien.

Based on Bayesian Inference, answer the following questions:

Question 1: What is the value of the prior probability P (h2)?

Question 2: What is the value of the likelihood P (d | h2)?

Question 3: What is the value of the normalizing term P (d)?

hint: P (d) can be calculated with P (d | h1) P (h1) + P (d | h2) P (h2)

Question 4: What is the value of the posterior probability P (h2 | d)?

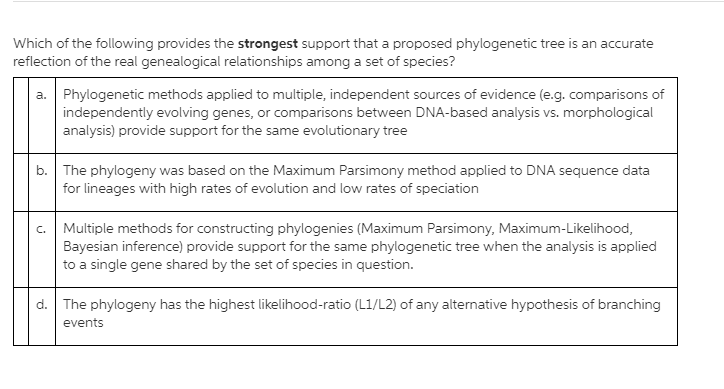
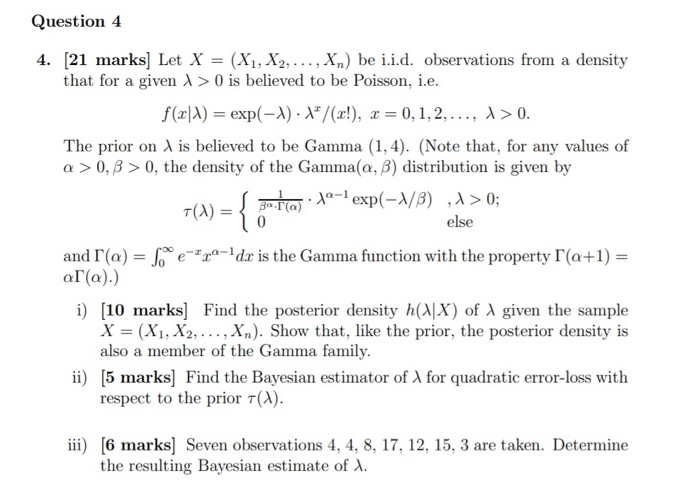
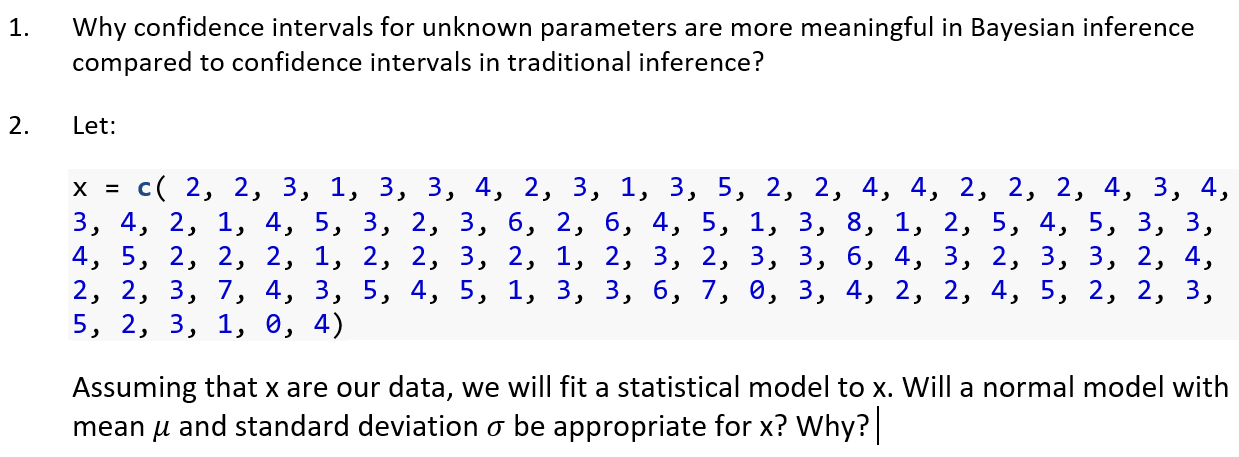
1|."v.I"hich of the following provides the strongest support that a proposed phylogenetic tree is an accurate reflection of the real genealogical relationships among a set of species? Phylogenetic methods applied to multiple; independent sources of evidence {e.g. comparisons of independently evolving genes, or comparisons between DNAbased analysis vs. morphological analysis] provide support for the same evolutionary tree The phylogeny was based on the Maximum Parsimony method applied to DNA sequence data for lineages with high rates of evolution and low rates of speciation Multiple methods for constructing phylogenies [Maximum Parsimony, Maximum-Likelihood, Bayesian inference] provide support for the same phylogenetic tree when the analysis is applied to a single gene shared by the set of species in question. The phylogeny has the highest likelihood-ratio{L1fL2} of any altemative hypothesis of branching events Question 4 4. [21 marks] Let X = {XhX9,...,Xn) be i.i.d. observations from a density that for a given A 3.:- U is believed to he Poisson, i.e. you) = armi) - won, a: = o,1,e...., i s o. The prior on A is believed to be Gamma (1,4). [Note that, for any values of o :e i}, :3 :a- U, the density of the Gamma(o,,8] distribution is given by 1 _ cll _ T r{.i}={ gnurm 3' esp{ M13} ;ie}+ and Mo) = I: e"s\"'1d:r is the Gamma function with the property l"{o:+1] = oI'(o).] i} [ID marks] Find the posterior density MAM) of A given the sample X = (X11X3,...,Xn}. Show that, like the prior, the posterior density is also a member of the Gamma family. ii} [5 marks] Find the Bayesian estimator of .1 for quadratic error-loss with respect to the prior 11).}. iii} [it marks] Seven observations 4, 4* 3, 1'1", 12, 15* 3 are taken Determine the Insulting Bayesian estimate of A. 7. [14 POINTS] ACME Pharmaceuticals has developed a new arthritis medication. Based on prelimi- mary experiments, the statisticians believe the proportion @ of arthritis sufferers who will experience relief with this medication is a random variable with the following distribution: .50 .65 .60 65 P(0 = 0) .10 40 40 .10 The medication was administered to twenty individuals, fifteen of whom reported feeling relief. The likelihood function is binomial: P(Y - 15/0 - 9) = (75)915(1 -8). Complete the following table for Bayesian inference. Suppose it is assumed in this simple model that & can only take values from {0.50, 0.55, 0.60, 0.65). Note you must compute the normalization constant . P(Y = 1510 - 0) x P(0 - 8). P(0 = 0) P(Y - 1510 = 0) P(Y = 1510 - 0) x P(0 - 0) P(0 = 0|Y = 15) 0.50 0.10 0.014786 0.0014786 0.55 0.036471 0.60 0.40 0.65 0.10 0.0127199 Normalization constant = > P(Y = 15/0 = 0) xP(0 = 0) =Why confidence intervals for unknown parameters are more meaningful in Bayesian inference compared to confidence intervals in traditional inference? Let: X=c(2, 2, 3, 1, 3, 3,4, 2, 3, 1, 3, 5, 2, 2,4,4, 2, 2, 2,4, 3,4, 3, 4, 2: 1, 4, 5, 3, 2, 3, 5, 2: 5, 4, 5, 1, 3, 8, 1, 2: 5, 4, 5, 3, 3, 4, 5, 2, 2, 2, 1, 2, 2, 3, 2, 1, 2, 3, 2, 3, 3, 5, 4, 3, 2, 3, 3, 2, 4, 2, 2, 3: 7, 4, 3, 5, 4, 5, 1, 3: 3, 6, 7, '3, 3, 4, 2, 2: 4, 5, 2, 2, 3, 5, 2, 3, 1, 9, 4) Assuming that x are our data, we will fit a statistical model to x. Will a normal model with mean u and standard deviation 0' be appropriate for x? Why?|