Question
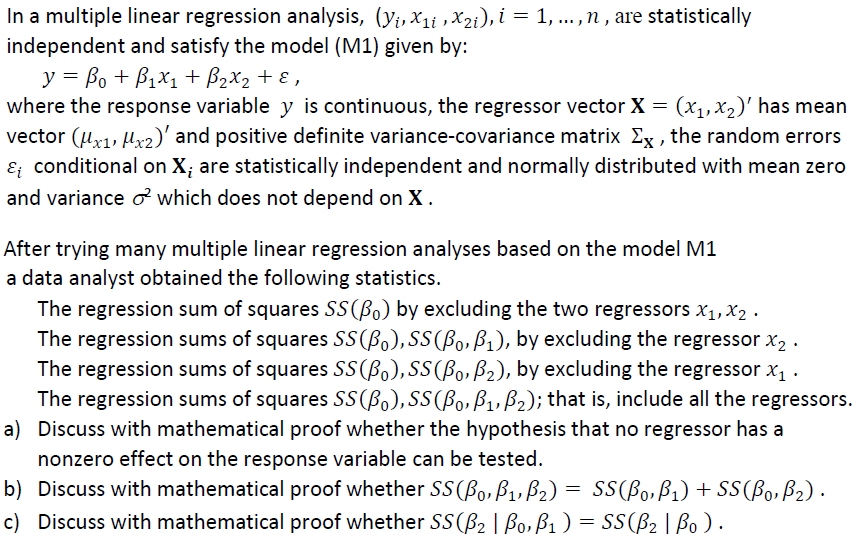
In a multiple linear regression analysis, ( y i , x 1 i , x 2 i ), i = 1, ... , n ,
In a multiple linear regression analysis, (yi , x1i , x2i ), i = 1, ... , n , are statistically independent and satisfy the model (M1) given by:
y = B0 + B1x1 + B2x2 + ? ,
where the response variable y is continuous, the regressor vector X = (x1, x2)T has mean vector (?x1, ? x2)T and positive definite variance-covariance matrix ?X , the random errors ?i conditional on X? are statistically independent and normally distributed with mean zero and variance ?2 which does not depend on X .
After trying many multiple linear regression analyses based on the model M1 in Problem 1 above, a data analyst obtained the following statistics.
The regression sum of squares SS(B0) by excluding the two regressors x1, x2 .
The regression sums of squares SS(B0), SS(B0, B1), by excluding the regressor x2.
The regression sums of squares SS(B0), SS(B0, B2), by excluding the regressor x1.
The regression sums of squares SS(B0), SS(B0, B1, B2); that is, include all the regressors.
a) Discuss with mathematical proof whether the hypothesis that no regressor has a nonzero effect on the response variable can be tested.
b) Discuss with mathematical proof whether SS(B0, B1, B2) = SS(B0, B1) + SS(B0, B2) .
c) Discuss with mathematical proof whether S?(B2 | B0, B1 ) = SS(B2 | B0 ) .
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


