In economics, goods which present a non-exclusion of use but a rivalry in their consumption are called common goods. Often used by a community of users, these goods would be doomed to be overexploited. In this problem, it is "The tragedy of the commons", article by Garrett Hardin first published in 1968 by the journal Science, which has had an extraordinary destiny, becoming a reference in disciplines as diverse as economics, ecology, geography, philosophy, anthropology and even science. right. We measure who is more is his success in fact that his words could be used to defend sometimes opposing theses. We will think in particular of the way in which it has become both the favorite argument of defenders of a libertarian conception of exclusive property and those of a drastic limitation of individual property rights for ecological reasons. Such contradictions can only be a sign of the richness of the subject of Hardin, open to the most divergent selective interpretations. In this problem, we study "the tragedies of the commons" from the perspective of the theory of games. Consider a pasture in a village where there are N goat herders grazing there their flock. Goats are a source of several valuable products: apart from meat whose consumption is very widespread, there is of course manure, but above all milk for its marketing and the manufacture of cheese and their skins for the rapidly expanding leather industry.

At the start of the season, each breeder buys a certain number of doelings at the unit price c. Let xi be the number of doelings -- considered as a continuous variable -- purchased by breeder i who grazes his herd in the pasture. The total number of does eating grass in the pasture is then X = x1 + ... + xi + ... + xN. Let us assume that the maximum carrying capacity of this pasture corresponds to the number of animals in good fattening condition at the end of the season and therefore salable. Let Xmax be this maximum number of animals. Let v[X] be the value at the end of the season of an animal as a function of the grass of the pasture. The profit that a breeder, let's say i, derives from his herd is given by

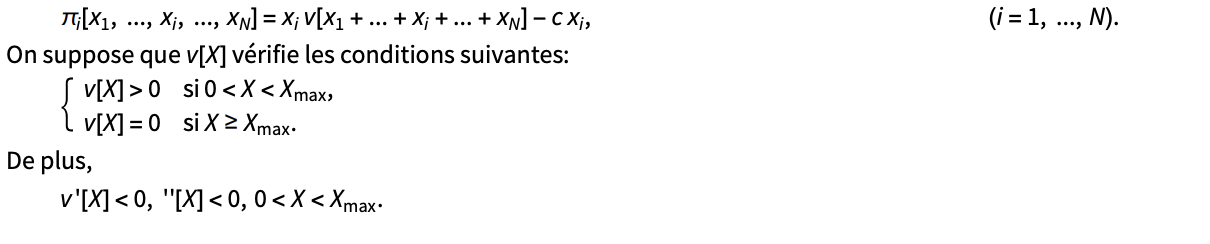
;[X1, Xi,..., X] = X; V[X + ... + X; + ... + XN] C Xi, (i = 1, ..., N). On suppose que v[X] vrifie les conditions suivantes: V[X]>0 si0