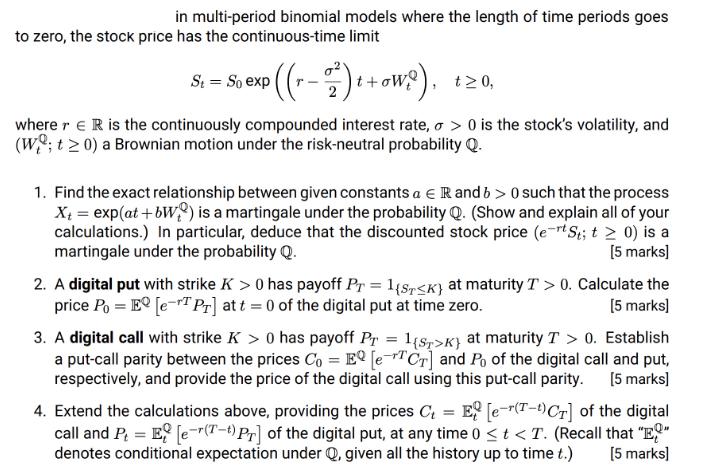
in multi-period binomial models where the length of time periods goes to zero, the stock price has the continuous-time limit S = So exp ((r - 2) + + ow) t0, where r = R is the continuously compounded interest rate, > 0 is the stock's volatility, and (W; t 0) a Brownian motion under the risk-neutral probability Q. 1. Find the exact relationship between given constants a R and 6> 0 such that the process X+= exp(at+bW) is a martingale under the probability Q. (Show and explain all of your calculations.) In particular, deduce that the discounted stock price (e-rt St; t > 0) is a martingale under the probability Q. [5 marks] 2. A digital put with strike K > 0 has payoff PT = 1{STK} at maturity T > 0. Calculate the price PoE [e-TPT] at t = 0 of the digital put at time zero. [5 marks] 3. A digital call with strike K> 0 has payoff PT = 1{ST>K} at maturity T > 0. Establish a put-call parity between the prices Co = EQ [CT] and Po of the digital call and put, respectively, and provide the price of the digital call using this put-call parity. [5 marks] 4. Extend the calculations above, providing the prices C = E [e-(T-CT] of the digital call and PE [er(T-) PT] of the digital put, at any time 0