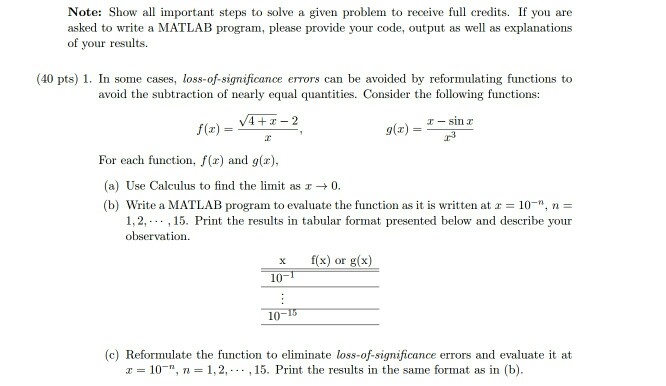
In some cases, loss-of-significance errors can be avoided by reformulating functions to avoid the subtraction of nearly equal quantities. Consider the following functions: f(x) = squareroot 4 + x - 2/x, g(x) = x - sin x/x^3 For each function, f(x) and g(x), (a) Use Calculus to find the limit as x rightarrow 0. (b) Write a MATLAB program to evaluate the function as it is written at x = 10^-n, n = 1, 2, ..., 15. Print the results in tabular format presented below and describe your observation. (c) Reformulate the function to eliminate loss-of-significance errors and evaluate it at x = 10^-n, n = 1, 2, ..., 15. Print the results in the same format as in (b)