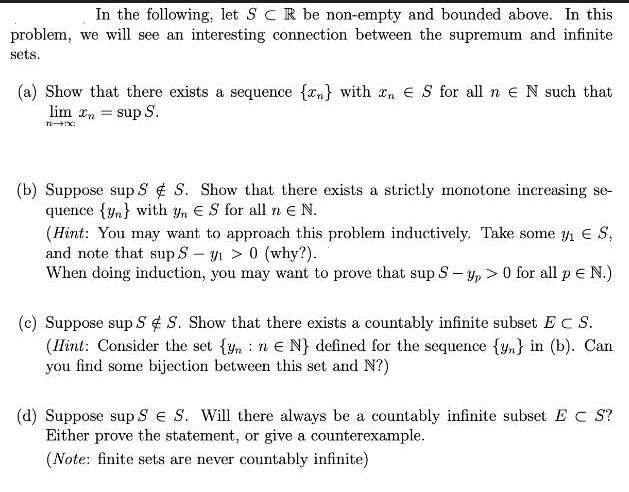
In the following, let S CR be non-empty and bounded above. In this problem, we will see an interesting connection between the supremum and infinite sets. (a) Show that there exists a sequence {n} with an ES for all n N such that = sup S. lim In 314-71 (b) Suppose sup S S. Show that there exists a strictly monotone increasing se- quence {n} with yn ES for all n N. (Hint: You may want to approach this problem inductively. Take some y S, and note that sup Sy > 0 (why?). When doing induction, you may want to prove that sup S-yp > 0 for all p = N.) (c) Suppose sup S S. Show that there exists a countably infinite subset EC S. (Hint: Consider the set {yn ne N} defined for the sequence {y} in (b). Can you find some bijection between this set and N?) (d) Suppose sup SE S. Will there always be a countably infinite subset E C S? Either prove the statement, or give a counterexample. (Note: finite sets are never countably infinite)